Article contents
Impact dynamics of compound drops of fluids with density contrast
Published online by Cambridge University Press: 02 June 2023
Abstract
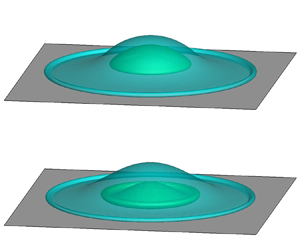
The dynamics of compound drops impacting on a flat substrate is numerically investigated using a ternary-fluid diffuse-interface method, with the aim of assessing the effect of a density difference between the inner and outer droplets (denoted by  $\lambda$) on the evolution of the interfaces. With the help of numerical simulations, we find that, at the intermediate stage of drop impact, the inner droplet exhibits a self-similar deformation at
$\lambda$) on the evolution of the interfaces. With the help of numerical simulations, we find that, at the intermediate stage of drop impact, the inner droplet exhibits a self-similar deformation at  $\lambda =1$ and relatively high Weber number, and experiences more or less a uniform acceleration for various
$\lambda =1$ and relatively high Weber number, and experiences more or less a uniform acceleration for various  $\lambda$. In particular, the acceleration magnitude at
$\lambda$. In particular, the acceleration magnitude at  $\lambda \ne 1$ can be correlated with the acceleration at
$\lambda \ne 1$ can be correlated with the acceleration at  $\lambda =1$ and the Atwood number. When the inner droplet is denser than the outer one, a lamella occurs at the spreading front of the inner droplet. We present a scaling analysis of the thickness of the lamella, and the resultant theoretical prediction is in good agreement with numerical results. At the maximal spreading of the compound drop, a bulging structure is formed around the symmetry axis due to the presence of the inner droplet, thereby effectively reducing the liquid supply to the spreading front and leading to a decrease of maximal spreading ratio
$\lambda =1$ and the Atwood number. When the inner droplet is denser than the outer one, a lamella occurs at the spreading front of the inner droplet. We present a scaling analysis of the thickness of the lamella, and the resultant theoretical prediction is in good agreement with numerical results. At the maximal spreading of the compound drop, a bulging structure is formed around the symmetry axis due to the presence of the inner droplet, thereby effectively reducing the liquid supply to the spreading front and leading to a decrease of maximal spreading ratio  $\beta _{max}$ as compared with a pure drop. We proposed a corrected Weber number
$\beta _{max}$ as compared with a pure drop. We proposed a corrected Weber number  $We^*_\lambda$ by taking account of the combined effects of
$We^*_\lambda$ by taking account of the combined effects of  $\lambda$, volume fraction of the inner droplet, Weber number and morphology of the compound drop. Integrating
$\lambda$, volume fraction of the inner droplet, Weber number and morphology of the compound drop. Integrating  $We^*_\lambda$ with the universal model of
$We^*_\lambda$ with the universal model of  $\beta _{max}$ for impacting pure drops, we successfully build up a new model for predicting the maximal spreading ratio of impacting compound drops with various
$\beta _{max}$ for impacting pure drops, we successfully build up a new model for predicting the maximal spreading ratio of impacting compound drops with various  $\lambda$.
$\lambda$.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 8
- Cited by


