Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yan, Zheng
Li, Xinliang
and
Yu, Changping
2022.
Helicity budget in turbulent channel flows with streamwise rotation.
Physics of Fluids,
Vol. 34,
Issue. 6,
Chan, C. I.
and
Chin, R. C.
2022.
Decomposition of the Reynolds shear stress in a turbulent boundary layer modified by miniature vortex generators.
Physical Review Fluids,
Vol. 7,
Issue. 5,
Kawata, T.
and
Tsukahara, T.
2022.
Spectral analysis on dissimilarity between turbulent momentum and heat transfers in plane Couette turbulence.
Physics of Fluids,
Vol. 34,
Issue. 7,
Chan, C.I.
and
Chin, R.C.
2022.
Investigation of the influence of miniature vortex generators on the large-scale motions of a turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 932,
Issue. ,
Gungor, Taygun R.
Maciel, Yvan
and
Gungor, Ayse G.
2022.
Energy transfer mechanisms in adverse pressure gradient turbulent boundary layers: production and inter-component redistribution.
Journal of Fluid Mechanics,
Vol. 948,
Issue. ,
Abdelaziz, Misarah
Djenidi, L.
Ghayesh, Mergen H.
and
Chin, Rey
2022.
Outer turbulent boundary layer similarities for different 2D surface roughnesses at matched Reynolds number.
International Journal of Heat and Fluid Flow,
Vol. 94,
Issue. ,
p.
108940.
Chan, C. I.
Örlü, R.
Schlatter, P.
and
Chin, R. C.
2022.
Large-scale and small-scale contribution to the skin friction reduction in a modified turbulent boundary layer by a large-eddy break-up device.
Physical Review Fluids,
Vol. 7,
Issue. 3,
Kawata, Takuya
and
Tsukahara, Takahiro
2022.
Spectral Analysis on Transport Budgets of Turbulent Heat Fluxes in Plane Couette Turbulence.
Energies,
Vol. 15,
Issue. 14,
p.
5258.
Ma, Rui
Gao, Zheng-hong
Lu, Lian-shan
and
Chen, Shu-sheng
2022.
Skin-friction drag reduction by local porous uniform blowing in spatially developing compressible turbulent boundary layers.
Physics of Fluids,
Vol. 34,
Issue. 12,
Zhang, Shen
Gui, Nan
Yang, Xingtuan
Tu, Jiyuan
and
Jiang, Shengyao
2023.
Rotational vector-based analysis of turbulent structures in channel flow using large eddy simulation simulation.
Fluid Dynamics Research,
Vol. 55,
Issue. 4,
p.
045505.
Chan, C.I.
and
Chin, R.C.
2023.
POD analysis of the turbulent boundary layer flow downstream of miniature vortex generators.
International Journal of Heat and Fluid Flow,
Vol. 103,
Issue. ,
p.
109175.
Fujino, Jun
Motoori, Yutaro
and
Goto, Susumu
2023.
Hierarchy of coherent vortices in turbulence behind a cylinder.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Abdelaziz, Misarah
Djenidi, L.
Ghayesh, Mergen H.
and
Chin, Rey
2023.
On the effect of streamwise and spanwise spacing to height ratios of three-dimensional sinusoidal roughness on turbulent boundary layers.
Physics of Fluids,
Vol. 35,
Issue. 2,
2023.
On the scale locality and vortex stretching in homogeneous shear turbulence.
Physics of Fluids,
Vol. 35,
Issue. 7,
Kinjangi, Dinesh Kumar
and
Foti, Daniel
2023.
Characterization of energy transfer and triadic interactions of coherent structures in turbulent wakes.
Journal of Fluid Mechanics,
Vol. 971,
Issue. ,
Abdelaziz, Misarah
Djenidi, L.
Ghayesh, Mergen H.
and
Chin, Rey
2023.
Influence of skewed three-dimensional sinusoidal surface roughness on turbulent boundary layers.
Physics of Fluids,
Vol. 35,
Issue. 5,
Nugroho, Setyo
Nugroho, Bagus
Fusil, Eric
and
Chin, Rey
2023.
Effects of varied roughness coverage area on drag in a turbulent boundary layer using numerical simulations.
Ocean Engineering,
Vol. 287,
Issue. ,
p.
115721.
Chan, C.I.
and
Chin, R.C.
2023.
Turbulent boundary layer flow over a three-dimensional sinusoidal surface.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Kong, Jiahao
Bennetts, Luke G.
Nugroho, Bagus
and
Chin, R. C.
2023.
Systematic study of the Reynolds number and streamwise spacing effects in two-dimensional square-bar rough-wall turbulent boundary layers.
Physical Review Fluids,
Vol. 8,
Issue. 1,
Fan, Yitong
and
Li, Weipeng
2023.
Spectral analysis of turbulence kinetic and internal energy budgets in hypersonic turbulent boundary layers.
Physical Review Fluids,
Vol. 8,
Issue. 4,
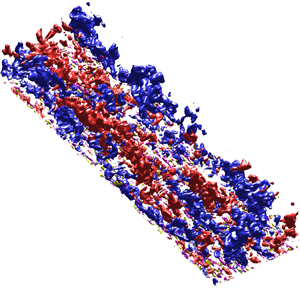
 $Re_\tau \simeq 2020$. Here, we extend the framework of Kawata & Alfredsson (Phys. Rev. Lett., vol. 120, 2018, p. 244501) for plane Couette flows to zero-pressure-gradient boundary layers. The equation contains three fundamental fluxes, which govern the Reynolds stress transport: (i) a scale flux of the interaction between small-scale and large-scale structures, and two spatial fluxes dominated by (ii) pressure and (iii) turbulent transport along the wall-normal direction. The scale flux reveals evidence of the inverse turbulent kinetic energy transfer, from small to large scales, occurring at the near-wall region, whereby for the scale flux of the Reynolds shear stress transport, the inverse transfer extends across the entire boundary layer. The wall-normal fluxes reveal the interactions occurring between scales at the buffer and logarithmic regions. In addition, there is interaction between the large-scale structures and the free stream flow occurring at the edge of the boundary layer, which was not observed in the Couette flow. Flow structures associated with inverse interscale transport of Reynolds shear stress are identified by applying conditional analysis to the spectrally decomposed velocity fields. While the inverse transport is interpreted as the net energy transfer from small-scale ejections (
$Re_\tau \simeq 2020$. Here, we extend the framework of Kawata & Alfredsson (Phys. Rev. Lett., vol. 120, 2018, p. 244501) for plane Couette flows to zero-pressure-gradient boundary layers. The equation contains three fundamental fluxes, which govern the Reynolds stress transport: (i) a scale flux of the interaction between small-scale and large-scale structures, and two spatial fluxes dominated by (ii) pressure and (iii) turbulent transport along the wall-normal direction. The scale flux reveals evidence of the inverse turbulent kinetic energy transfer, from small to large scales, occurring at the near-wall region, whereby for the scale flux of the Reynolds shear stress transport, the inverse transfer extends across the entire boundary layer. The wall-normal fluxes reveal the interactions occurring between scales at the buffer and logarithmic regions. In addition, there is interaction between the large-scale structures and the free stream flow occurring at the edge of the boundary layer, which was not observed in the Couette flow. Flow structures associated with inverse interscale transport of Reynolds shear stress are identified by applying conditional analysis to the spectrally decomposed velocity fields. While the inverse transport is interpreted as the net energy transfer from small-scale ejections ( $Q2$) and sweeps (
$Q2$) and sweeps ( $Q4$) to the large-scale counterparts, conditional time estimates of the direct and inverse interscale transport reveal that both processes play a substantial role across a broad range of scales.
$Q4$) to the large-scale counterparts, conditional time estimates of the direct and inverse interscale transport reveal that both processes play a substantial role across a broad range of scales.