Published online by Cambridge University Press: 05 March 2019
We investigated experimentally the motion of elongated finite-length cylinders (length  $L$, diameter
$L$, diameter 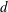 $d$) freely falling under the effect of buoyancy in a low-viscosity fluid otherwise at rest. For cylinders with densities
$d$) freely falling under the effect of buoyancy in a low-viscosity fluid otherwise at rest. For cylinders with densities 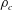 $\unicode[STIX]{x1D70C}_{c}$ close to the density
$\unicode[STIX]{x1D70C}_{c}$ close to the density 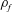 $\unicode[STIX]{x1D70C}_{f}$ of the fluid (
$\unicode[STIX]{x1D70C}_{f}$ of the fluid ( $\overline{\unicode[STIX]{x1D70C}}=\unicode[STIX]{x1D70C}_{c}/\unicode[STIX]{x1D70C}_{f}\simeq 1.16$), we explored the effect of the body volume by varying the Archimedes number
$\overline{\unicode[STIX]{x1D70C}}=\unicode[STIX]{x1D70C}_{c}/\unicode[STIX]{x1D70C}_{f}\simeq 1.16$), we explored the effect of the body volume by varying the Archimedes number 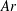 $Ar$ (based on the body equivalent diameter) between 200 and 1100, as well as the effect of their length-to-diameter ratios
$Ar$ (based on the body equivalent diameter) between 200 and 1100, as well as the effect of their length-to-diameter ratios 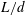 $L/d$ ranging from 2 to 20. A shadowgraphy technique involving two cameras mounted on a travelling cart was used to track the cylinders along their fall over a distance longer than
$L/d$ ranging from 2 to 20. A shadowgraphy technique involving two cameras mounted on a travelling cart was used to track the cylinders along their fall over a distance longer than 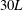 $30L$. A dedicated image processing algorithm was further implemented to properly reconstruct the position and orientation of the cylinders in the three-dimensional space. In the range of parameters explored, we identified three main types of paths, matching regimes known to exist for three-dimensional bodies (short-length cylinders, disks and spheres). Two of these are stationary, namely, the rectilinear motion and the large-amplitude oscillatory motion (also referred to as fluttering or zigzag motion), and their characterization is the focus of the present paper. Furthermore, in the transitional region between these two regimes, we observed irregular low-amplitude oscillatory motions, that may be assimilated to the A-regimes or quasi-vertical regimes of the literature. Flow visualization using dye released from the bodies uncovered the existence of different types of vortex shedding in the wake of the cylinders, according to the style of path. The detailed analysis of the body kinematics in the fluttering regime brought to light a series of remarkable properties. In particular, when normalized with the characteristic velocity scale
$30L$. A dedicated image processing algorithm was further implemented to properly reconstruct the position and orientation of the cylinders in the three-dimensional space. In the range of parameters explored, we identified three main types of paths, matching regimes known to exist for three-dimensional bodies (short-length cylinders, disks and spheres). Two of these are stationary, namely, the rectilinear motion and the large-amplitude oscillatory motion (also referred to as fluttering or zigzag motion), and their characterization is the focus of the present paper. Furthermore, in the transitional region between these two regimes, we observed irregular low-amplitude oscillatory motions, that may be assimilated to the A-regimes or quasi-vertical regimes of the literature. Flow visualization using dye released from the bodies uncovered the existence of different types of vortex shedding in the wake of the cylinders, according to the style of path. The detailed analysis of the body kinematics in the fluttering regime brought to light a series of remarkable properties. In particular, when normalized with the characteristic velocity scale  $u_{0}=\sqrt{(\overline{\unicode[STIX]{x1D70C}}-1)gd}$ and the characteristic length scale
$u_{0}=\sqrt{(\overline{\unicode[STIX]{x1D70C}}-1)gd}$ and the characteristic length scale 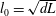 $l_{0}=\sqrt{dL}$, the mean vertical velocity
$l_{0}=\sqrt{dL}$, the mean vertical velocity 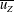 $\overline{u_{Z}}$ and the frequency
$\overline{u_{Z}}$ and the frequency 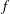 $f$ of the oscillations become almost independent of
$f$ of the oscillations become almost independent of 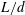 $L/d$ and
$L/d$ and  $Ar$. The use of the length scale
$Ar$. The use of the length scale 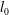 $l_{0}$ and of the gravitational velocity scale to build the Strouhal number
$l_{0}$ and of the gravitational velocity scale to build the Strouhal number  $St^{\ast }=fl_{0}/u_{0}$ allowed us to generalize to short (
$St^{\ast }=fl_{0}/u_{0}$ allowed us to generalize to short (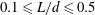 $0.1\leqslant L/d\leqslant 0.5$) and elongated cylinders (
$0.1\leqslant L/d\leqslant 0.5$) and elongated cylinders (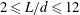 $2\leqslant L/d\leqslant 12$), the result
$2\leqslant L/d\leqslant 12$), the result 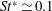 $St^{\ast }\simeq 0.1$. An interpretation of
$St^{\ast }\simeq 0.1$. An interpretation of 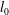 $l_{0}$ as a characteristic length scale associated with the oscillatory recirculation thickness generated near the body ends is proposed. In addition, the rotation rate of the cylinders scales with
$l_{0}$ as a characteristic length scale associated with the oscillatory recirculation thickness generated near the body ends is proposed. In addition, the rotation rate of the cylinders scales with 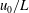 $u_{0}/L$, for all
$u_{0}/L$, for all 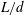 $L/d$ and
$L/d$ and 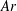 $Ar$ investigated. Furthermore, the phase difference between the oscillations of the velocity component
$Ar$ investigated. Furthermore, the phase difference between the oscillations of the velocity component  $u$ along the cylinder axis and of the inclination angle
$u$ along the cylinder axis and of the inclination angle 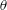 $\unicode[STIX]{x1D703}$ of the cylinder is approximately constant, whatever the elongation ratio
$\unicode[STIX]{x1D703}$ of the cylinder is approximately constant, whatever the elongation ratio 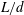 $L/d$ and the Archimedes number
$L/d$ and the Archimedes number 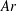 $Ar$.
$Ar$.