Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-13T15:51:29.325Z Has data issue: false hasContentIssue false
  • English
  • Français

A Kolmogorov-like exact relation for compressible polytropic turbulence

Published online by Cambridge University Press:  21 February 2014

Supratik Banerjee Open the ORCID record for Supratik Banerjee [Opens in a new window]  and
Sébastien Galtier
Get access Rights & Permissions [Opens in a new window]

Abstract

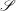Compressible hydrodynamic turbulence is studied under the assumption of a polytropic closure. Following Kolmogorov, we derive an exact relation for some two-point correlation functions in the asymptotic limit of a high Reynolds number. The inertial range is characterized by: (i) a flux term implying in particular the enthalpy; and (ii) a purely compressible term $\mathcal{S}$ which may act as a source or a sink for the mean energy transfer rate. At subsonic scales, we predict dimensionally that the isotropic 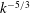$k^{-5/3}$ energy spectrum for the density-weighted velocity field ($\rho ^{1/3} \boldsymbol {v}$), previously obtained for isothermal turbulence, is modified by a polytropic contribution, whereas at supersonic scales 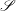$\mathcal{S}$ may impose another scaling depending on the polytropic index. In both cases, it is shown that the fluctuating sound speed is a key ingredient for understanding polytropic compressible turbulence.

JFM classification

Turbulent Flows: Compressible turbulence Turbulent Flows: Homogeneous turbulence Turbulent Flows: Turbulence theory
Type
Papers
Information
Journal of Fluid Mechanics , Volume 742 , 10 March 2014 , pp. 230 - 242
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aluie, H. 2011 Compressible turbulence: the cascade and its locality. Phys. Rev. Lett. 106, 174502.CrossRefGoogle ScholarPubMed
Aluie, H. 2013 Scale decomposition in compressible turbulence. Physica D 247, 54–65.Google Scholar
Antonia, R. A., Ould-Rouis, M., Anselmet, F. & Zhu, Y. 1997 Analogy between predictions of Kolmogorov and Yaglom. J. Fluid Mech. 332, 395–409.Google Scholar
Armstrong, J. W., Cordes, J. M. & Rickett, B. J. 1981 Scale decomposition in compressible turbulence. Nature 291, 561–564.CrossRefGoogle Scholar
Banerjee, S. & Galtier, S. 2013 Exact relation with two-point correlation functions and phenomenological approach for compressible magnetohydrodynamic turbulence. Phys. Rev. E 87, 013019.Google Scholar
Bataille, F. & Zhou, Ye 1999 Nature of the energy transfer process in compressible turbulence. Phys. Rev. E 59, 5417–5426.Google Scholar
Belmont, G., Grappin, R., Mottez, F., Pantellinin, F. & Pelletier, G. 2014 Collisionless Plasmas in Astrophysics. Wiley-VCH.Google Scholar
Benzi, R., Biferale, L., Fisher, R. T., Kadanoff, L. P., Lamb, D. Q. & Toschi, F. 2008 Intermittency and universality in fully developed inviscid and weakly compressible turbulent flows. Phys. Rev. Lett. 100 (23), 234503.CrossRefGoogle ScholarPubMed
Bhattacharjee, A., Ng, C. S. & Spangler, S. R. 1998 Weakly compressible magnetohydrodynamic turbulence in the solar wind and the interstellar medium. Astrophys. J. 494, 409–418.Google Scholar
Biskamp, D. 2008 Magnetohydrodynamic Turbulence. Cambridge University Press.Google Scholar
Biskamp, D., Schwarz, E. & Drake, J. F. 1996 Two-dimensional electron magnetohydrodynamic turbulence. Phys. Rev. Lett. 76, 1264–1267.Google Scholar
Buchlin, E., Aletti, V., Galtier, S., Velli, M., Einaudi, G. & Vial, J. C. 2003 A simplified numerical model of coronal energy dissipation based on reduced MHD. Astron. Astrophys. 406 (3), 1061–1070.CrossRefGoogle Scholar
Carbone, V., Marino, R., Sorriso-Valvo, L., Noullez, A. & Bruno, R. 2009 Scaling laws of turbulence and heating of fast solar wind: the role of density fluctuations. Phys. Rev. Lett. 103, 061102.Google Scholar
Elmegreen, B. G. & Scalo, J. 2004 Interstellar turbulence I: observations and processes. Annu. Rev. Astron. Astrophys. 42, 211–273.Google Scholar
Falkovich, G., Fouxon, I. & Oz, Y. 2010 New relations for correlation functions in Navier–Stokes turbulence. J. Fluid Mech. 644, 465–472.Google Scholar
Federrath, C. 2013 On the universality of supersonic turbulence. Mon. Not. R. Astron. Soc. on line, arXiv:1306.3989v1.CrossRefGoogle Scholar
Federrath, C., Roman-Duval, J., Klessen, R. S., Schmidt, W. & Low, M. Mac 2010 Comparing the statistics of interstellar turbulence in simulations and observations: solenoidal versus compressive turbulence forcing. Astron. Astrophys. 512, A81.CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence: The Legacy of A. N. Kolmogorov. Cambridge University Press.Google Scholar
Galtier, S. 2008 von Karman–Howarth equations for hall magnetohydrodynamic flows. Phys. Rev. E 77, R015302.Google Scholar
Galtier, S. 2009 Wave turbulence in magnetized plasmas. Nonlinear Process. Geophys. 16, 83–98.Google Scholar
Galtier, S. 2012 A vectorial law for solar wind turbulence. Astrophys. J. 746, 184, 1–4.Google Scholar
Galtier, S. & Banerjee, S. 2011 Exact relation for correlation functions in compressible isothermal turbulence. Phys. Rev. Lett. 107, 134501.Google Scholar
Hennebelle, P. & Audit, E. 2007 On the structure of the turbulent interstellar atomic hydrogen. I. Physical characteristics. Influence and nature of turbulence in a thermally bistable flow. Astron. Astrophys. 465, 431–443.Google Scholar
Hennebelle, P. & Chabrier, G. 2011 Analytical star formation rate from gravoturbulent fragmentation. Astrophys. J. 743, L29.Google Scholar
Horedt, G. P. 2004 Polytropes: Applications in Astrophysics and Related Fields. Kluwer Academic.Google Scholar
Hu, Y. Q., Esser, R. & Habbal, S. R. 1997 A fast solar wind model with anisotropic proton temperature. J. Geophys. Res. 102, 14661–14676.Google Scholar
Kim, J. & Ryu, D. 2005 Density power spectrum of compressible hydrodynamic turbulent flows. Astrophys. J. 630, L45–L48.CrossRefGoogle Scholar
Kolmogorov, A. N. 1941 The local structure of turbulence in incompressible viscous fluids at very large Reynolds numbers. Dokl. Akad. Nauk SSSR 30, 301–305.Google Scholar
Kraichnan, R. H. 1965 Inertial range spectrum in hydromagnetic turbulence. Phys. Fluids 8, 1385–1387.Google Scholar
Kritsuk, A. G., Norman, M. L., Padoan, P. & Wagner, R. 2007 The statistics of supersonic isothermal turbulence. Astrophys. J. 665, 416–431.Google Scholar
Kritsuk, A. G., Wagner, R. & Norman, M. L. 2013 Energy cascade and scaling in supersonic isothermal turbulence. J. Fluid Mech. R. 729, R1.Google Scholar
Laveder, D., Passot, T. & Sulem, P. L. 2013 Intermittent dissipation and lack of universality in one-dimensional alfvenic turbulence. Phys. Lett. A 377, 1535–1541.Google Scholar
Lee, S., Lele, S. K. & Moin, P. 1991 Eddy shocklets in decaying compressible turbulence. Phys. Fluids A 3, 657–664.Google Scholar
Lindborg, E. 2007 Third-order structure function relations for quasi-geostrophic turbulence. J. Fluid Mech. 572, 255–260.Google Scholar
L’vov, V. S., L’vov, Y., Newell, A. C. & Zakharov, V. 1997 Statistical description of acoustic turbulence. Phys. Rev. E 56, 390–405.Google Scholar
Marino, R., Sorriso-Valvo, L., Carbone, V., Noullez, A. & Bruno, R. 2010 Compressive turbulent cascade and heating in the solar wind. Twelfth International Solar Wind Conference 1216, 156–159.Google Scholar
Meyrand, R. & Galtier, S. 2010 A universal law for solar-wind turbulence at electron scales. Astrophys. J. 721, 1421–1424.Google Scholar
Meyrand, R. & Galtier, S. 2012 Spontaneous chiral symmetry breaking of hall mhd turbulence. Phys. Rev. Lett. 109, 194501.Google Scholar
Newell, A. C. & Aucoin, P. J. 1971 Semi-dispersive wave systems. J. Fluid Mech. 49, 593–609.Google Scholar
Passot, T. & Pouquet, A. 1987 Numerical simulation of compressible homogeneous flows in the turbulent regime. J. Fluid Mech. 181, 441–466.CrossRefGoogle Scholar
Passot, T., Pouquet, A. & Woodward, P. 1988 On the plausibility of Kolmogorov-type spectra in molecular clouds. Astron. Astrophys. 197, 228–234.Google Scholar
Passot, T. & Vazquez-Semadini, E. 1998 Density probability distribution in one-dimensional polytropic gas dynamics. Phys. Rev. E 58, 4501–4510.Google Scholar
Podesta, J. J. 2008 Laws for third-order moments in homogeneous anisotropic incompressible magnetohydrodynamic turbulence. J. Fluid Mech. 609, 171–194.Google Scholar
Politano, H. & Pouquet, A. 1998 A von Kármán–Howarth equation for MHD fluids and its consequences on third-order longitudinal structure and correlation functions. Phys. Rev. E 57, R21–R24.Google Scholar
Porter, D. H., Pouquet, A. & Woodward, P. R. 1992 Three-dimensional supersonic homogeneous turbulence: a numerical study. Phys. Rev. Lett. 68, 3156–3159.Google Scholar
Porter, D. H., Pouquet, A. & Woodward, P. R. 1994 Kolmogorov-like spectra in decaying three-dimensional supersonic flows. Phys. Fluids 6, 2133–2142.Google Scholar
Pouquet, A. 1993 Magnetohydrodynamic turbulence. In Astrophysical Fluid Dynamics (ed. Zahn, J.-P. & Zinn-Justin, J.), pp. 139–227. Elsevier.Google Scholar
Renard, M. & Chieze, J. P. 1993 The fragmentation of molecular clouds: critical (Jeans) mass in the vicinity of thermal instability and influence of visible extinction variations. Astron. Astrophys. 267, 549–558.Google Scholar
Sagaut, P. & Cambon, C. 2008 Homogeneous Turbulence Dynamics. Cambridge University Press.Google Scholar
Schmidt, W., Federrath, C., Hupp, M., Kern, S. & Niemeyer, J. C. 2009 Numerical simulations of compressively driven interstellar turbulence. I. Isothermal gas. Astron. Astrophys. 494, 127–145.CrossRefGoogle Scholar
Schmidt, W., Federrath, C. & Klessen, R. 2008 Is the scaling of supersonic turbulence universal?. Phys. Rev. Lett. 101, 194505.Google Scholar
Tu, C.-Y. & Marsch, E. 1997 Two-fluid model for heating of the solar corona and acceleration of the solar wind by high-frequency Alfven waves. Solar Phys. 171, 363–391.Google Scholar
Vázquez-Semadeni, E., Gazol, A., Passot, T. & Sanchez-Salcedo, J. 2003 Thermal instability and magnetic pressure in the turbulent interstellar medium. In Turbulence and Magnetic Fields in Astrophysics (ed. Falgarone, E. & Passot, T.), Lecture Notes in Physics, vol. 614, pp. 213–251. Springer.CrossRefGoogle Scholar
Vázquez-Semadeni, E., Ostriker, E. C., Passot, T., Gammie, C. F. & Stone, J. M. 2000 In Protostars and Planets IV (ed. Boss, A.P., Mannings, V. & Russel, S.S.), University Arizona.Google Scholar
Vázquez-Semadeni, E., Passot, T. & Pouquet, A. 1996 Influence of cooling-induced compressibility on the structure of turbulent flows and gravitational collapse. Astrophys. J. 473, 881–893.Google Scholar
Wagner, R., Falkovich, G., Kritsuk, A. G. & Norman, M. L. 2012 Flux correlations in supersonic isothermal turbulence. J. Fluid Mech. 713, 482–490.Google Scholar
Yaglom, A. M. 1949 Local structure of the temperature field in a turbulent flow. Dokl. Akad. Nauk SSSR 69, 743–746.Google Scholar
Zakharov, V. E. & Sagdeev, R. Z. 1970 Spectrum of acoustic turbulence. Sov. Phys. Dokl. 15, 439–441.Google Scholar