Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-10T22:18:16.008Z Has data issue: false hasContentIssue false
  • English
  • Français

Length of near-wall plumes in turbulent convection

Published online by Cambridge University Press:  20 September 2011

Baburaj A. Puthenveettil ,
G. S. Gunasegarane ,
Yogesh K. Agrawal ,
Daniel Schmeling ,
Johannes Bosbach  and
Jaywant H. Arakeri
Baburaj A. Puthenveettil*
Affiliation:
Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, 600036, India
G. S. Gunasegarane
Affiliation:
Department of Applied Mechanics, Indian Institute of Technology Madras, Chennai, 600036, India
Yogesh K. Agrawal
Affiliation:
Department of Mechanical Engineering, National Institute of Technology, Durgapur, 713209, India
Daniel Schmeling
Affiliation:
Institute of Aerodynamics and Flow Technology, German Aerospace Center (DLR), Göttingen, 37073, Germany
Johannes Bosbach
Affiliation:
Institute of Aerodynamics and Flow Technology, German Aerospace Center (DLR), Göttingen, 37073, Germany
Jaywant H. Arakeri
Affiliation:
Department of Mechanical Engineering, Indian Institute of Science, Bangalore, 560012, India
*
†Email address for correspondence: apbraj@iitm.ac.in
Get access Rights & Permissions [Opens in a new window]

Abstract

We present planforms of line plumes formed on horizontal surfaces in turbulent convection, along with the length of line plumes measured from these planforms, in a six decade range of Rayleigh numbers (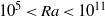) and at three Prandtl numbers (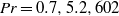). Using geometric constraints on the relations for the mean plume spacings, we obtain expressions for the total length of near-wall plumes on horizontal surfaces in turbulent convection. The plume length per unit area (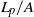), made dimensionless by the near-wall length scale in turbulent convection (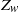), remains constant for a given fluid. The Nusselt number is shown to be directly proportional to for a given fluid layer of height 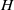. The increase in 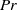has a weak influence in decreasing 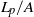. These expressions match the measurements, thereby showing that the assumption of laminar natural convection boundary layers in turbulent convection is consistent with the observed total length of line plumes. We then show that similar relationships are obtained based on the assumption that the line plumes are the outcome of the instability of laminar natural convection boundary layers on the horizontal surfaces.

JFM classification

Convection: Buoyant boundary layers Convection: Plumes/thermals Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 685 , 25 October 2011 , pp. 335 - 364
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Adrian, R. J., Ferreira, R. T. D. S. & Boberg, T. 1986 Turbulent thermal convection in wide horizontal fluid layers. Exp. Fluids 4, 121–141.CrossRefGoogle Scholar
2. Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large-scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503.CrossRefGoogle Scholar
3. Baker, J. D. 1966 A technique for the precise measurement of small fluid velocities. J. Fluid Mech. 26 (3), 573–575.CrossRefGoogle Scholar
4. Canny, J. 1986 A computational approach to edge detection. IEEE Trans. Pattern Anal. Mach. Intell. 8 (6), 679–698.CrossRefGoogle ScholarPubMed
5. Fuji, T. 1963 Theory of the steady laminar natural convection above a horizontal line source and a point heat source. Intl J. Heat Mass Transfer 6, 597–606.CrossRefGoogle Scholar
6. Funfschilling, D. & Ahlers, G. 2004 Plume motion and large scale circulation in a cylindrical Rayleigh–Bénard cell. Phys. Rev. Lett. 92 (19), 194502.CrossRefGoogle Scholar
7. Gebhart, B., Pera, L. & Schorr, A. W. 1970 Steady laminar natural convection plume above a horizontal line heat source. Intl J. Heat Mass Transfer 13, 161–171.CrossRefGoogle Scholar
8. Gebhart, B., Jaluria, Y., Mahajan, R. L. & Sammakia, B. 1988 Buoyancy Induced Flows and Transport. Hemisphere Publishing.Google Scholar
9. Gill, W. N., Zeh, D. W. & del Casal, E. 1965 Free convection on a horizontal plate. Z. Angew. Math. Phys. 16, 532–541.CrossRefGoogle Scholar
10. Globe, S. & Dropkin, D. 1959 Natural-convection heat transfer in liquids confined by two horizontal plates and heated from below. Trans. ASME, 24–28.CrossRefGoogle Scholar
11. Grossman, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27.CrossRefGoogle Scholar
12. Grossman, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh Bénard convection: the role of plumes. Phys. Fluids 16, 4462–4472.CrossRefGoogle Scholar
13. Gunasegarane, G. S. & Puthenveettil, B. A. 2010 Merging of sheet plumes in turbulent convection. In Proceedings of the 37th National and the 4th International Conference on Fluid Mechanics and Fluid Power (ed. B. V. S. S. Prasad). IIT Madras, Chennai, India, ISBN 978-81-910571-1-9, Valardocs, Chennai.Google Scholar
14. Haramina, T. & Tilgner, A. 2004 Coherent structures in boundary layers of Rayleigh–Bénard convection. Phys. Rev. E 69, 056306.CrossRefGoogle ScholarPubMed
15. Howard, L. N. 1964 Convection at high Rayleigh number. In Proceedings of the 11th International Congress Applied Mechanics, Munich (ed. H. Görtler), pp. 1109–1115.Google Scholar
16. Husar, R. B. & Sparrow, E. M. 1968 Patterns of free convection flow adjacent to horizontal heated surfaces. Intl J. Heat Mass Transfer 11, 1208–1211.CrossRefGoogle Scholar
17. Niemela, J. J., Skrbek, L., Sreenivasan, K. R. & Donnely, R. J. 2001 The wind in confined thermal convection. J. Fluid Mech. 449, 169–178.CrossRefGoogle Scholar
18. Pera, L. & Gebhart, B. 1973 On the stability of natural convection boundary layer flow over horizontal and slightly inclined surfaces. Intl J. Heat Mass Transfer 16, 1147–1163.CrossRefGoogle Scholar
19. Puthenveettil, B. A., Ananthakrishna, G. & Arakeri, J. H. 2005 Multifractal nature of plume structure in high Rayleigh number convection. J. Fluid Mech. 526, 245–256.CrossRefGoogle Scholar
20. Puthenveettil, B. A. & Arakeri, J. H. 2005 Plume structure in high Rayleigh number convection. J. Fluid Mech. 542, 217–249.CrossRefGoogle Scholar
21. Puthenveettil, B. A. & Arakeri, J. H. 2008 Convection due to an unstable density difference across a permeable membrane. J. Fluid Mech. 609, 139–170.CrossRefGoogle Scholar
22. Ramareddy, G. V. & Puthenveettil, B. A. 2011 The Pe ∼ 1 regime of convection across a horizontal permeable membrane. J. Fluid Mech. 679, 476–504.CrossRefGoogle Scholar
23. Rotem, Z. & Classen, L. 1969 Natural convection above unconfined horizontal surfaces. J. Fluid Mech. 39 (part 1), 173–192.CrossRefGoogle Scholar
24. Shishkina, O. & Wagner, C. 2007 Local fluxes in turbulent Rayleigh–Bénard convection. Phys. Fluids 19, 085107.CrossRefGoogle Scholar
25. Shishkina, O. & Wagner, C. 2008 Analyis of sheet-like thermal plumes in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 599, 383–404.CrossRefGoogle Scholar
26. Stevens, R. J. A. M., Verzicco, R. & Lohse, D. 2010 Radial boundary layer structure and Nusselt number in Rayleigh Bénard convection. J. Fluid Mech. 643, 495–507.CrossRefGoogle Scholar
27. Tamai, N. & Asaeda, T. 1984 Sheet like plumes near a heated bottom plate at large Rayleigh number. J. Geophys. Res. 89, 727–734.CrossRefGoogle Scholar
28. Theerthan, S. A. & Arakeri, J. H. 1998 A model for near wall dynamics in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 373, 221–254.CrossRefGoogle Scholar
29. Theerthan, S. A. & Arakeri, J. H. 2000 Plan form structure and heat transfer in turbulent free convection over horizontal surfaces. Phys. Fluids 12, 884–894.CrossRefGoogle Scholar
30. Townsend, A. A. 1959 Temperature fluctuations over a heated horizontal surface. J. Fluid Mech. 5, 209–211.CrossRefGoogle Scholar
31. Xia, K. Q., Lam, S. & Zhou, S. Q. 2002 Heat flux measurement in high Prandtl number turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 88 (6), 064501.CrossRefGoogle ScholarPubMed
32. Zhou, Q., Sun, C. & Xia, K. Q. 2007 Morphological evolution of thermal plumes in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 98, 074501.CrossRefGoogle ScholarPubMed
33. Zhou, Q. & Xia, K. Q. 2010 Physical and geometrical properties of thermal plumes in turbulent Rayleigh Bénard convection. New J. Phys. 12, 075006.CrossRefGoogle Scholar
34. Zocchi, G., Moses, E. & Libchaber, A. 1990 Coherent structures in turbulent convection, an experimental study. Physica A 166, 387–407.CrossRefGoogle Scholar