Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-10T06:55:30.677Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear modal instabilities of hypersonic flow over an elliptic cone

Published online by Cambridge University Press:  09 September 2016

Pedro Paredes ,
Ryan Gosse ,
Vassilis Theofilis  and
Roger Kimmel
Pedro Paredes*
Affiliation:
School of Aeronautics, Universidad Politécnica de Madrid, Madrid 28040, Spain
Ryan Gosse
Affiliation:
Air Force Research Laboratory, WPAFB, OH 45433, USA
Vassilis Theofilis
Affiliation:
School of Aeronautics, Universidad Politécnica de Madrid, Madrid 28040, Spain School of Engineering, University of Liverpool, Browlow Hill, Liverpool L69 3GH, UK
Roger Kimmel
Affiliation:
Air Force Research Laboratory, WPAFB, OH 45433, USA
*
†Email address for correspondence: pedro.paredes@upm.es
Get access Rights & Permissions [Opens in a new window]

Abstract

Steady laminar flow over a rounded-tip 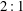$2\,:\,1$ elliptic cone of 0.86 m length at zero angle of attack and yaw has been computed at Mach number 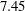$7.45$ and unit Reynolds number $Re^{\prime }=1.015\times 10^{7}~\text{m}^{-1}$. The flow conditions are selected to match the planned flight of the Hypersonic Flight Research Experimentation HIFiRE-5 test geometry at an altitude of 21.8 km. Spatial linear BiGlobal modal instability analysis of this flow has been performed at selected streamwise locations on planes normal to the cone symmetry axis, resolving the entire flow domain in a coupled manner while exploiting flow symmetries. Four amplified classes of linear eigenmodes have been unravelled. The shear layer formed near the cone minor-axis centreline gives rise to amplified symmetric and antisymmetric centreline instability modes, classified as shear-layer instabilities. At the attachment line formed along the major axis of the cone, both symmetric and antisymmetric instabilities are also discovered and identified as boundary-layer second Mack modes. In both cases of centreline and attachment-line modes, symmetric instabilities are found to be more unstable than their antisymmetric counterparts. Furthermore, spatial BiGlobal analysis is used for the first time to resolve oblique second modes and cross-flow instabilities in the boundary layer between the major- and minor-axis meridians. Contrary to predictions for the incompressible regime for swept infinite wing flow, the cross-flow instabilities are not found to be linked to the attachment-line instabilities. In fact, cross-flow modes peak along most of the surface of the cone, but vanish towards the attachment line. On the other hand, the leading oblique second modes peak near the leading edge and their associated frequencies are in the range of the attachment-line instability frequencies. Consequently, the attachment-line instabilities are observed to be related to oblique second modes at the major-axis meridian. The linear amplification of centreline and attachment-line instability modes is found to be strong enough to lead to laminar–turbulent flow transition within the length of the test object. The predictions of global linear theory are compared with those of local instability analysis, also performed here under the assumption of locally parallel flow, where use of this assumption is permissible. Fair agreement is obtained for symmetric centreline and symmetric attachment-line modes, while for all other classes of linear disturbances use of the proposed global analysis methodology is warranted for accurate linear instability predictions.

JFM classification

Boundary Layers: Boundary layer stability Compressible Flows: Compressible boundary layers Instability: Transition to turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 804 , 10 October 2016 , pp. 442 - 466
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amestoy, P. R., Duff, I. S., L’Excellent, J.-Y. & Koster, J. 2001 A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Applics. 1, 15–41.Google Scholar
Amestoy, P. R., Guermouche, A., L’Excellent, J.-Y. & Pralet, S. 2006 Hybrid scheduling for the parallel solution of linear systems. Parallel Comput. 2, 136–156.Google Scholar
Arnoldi, W. E. 1951 The principle of minimized iterations in the solution of the matrix eigenvalue problem. Q. Appl. Maths 9, 17–29.Google Scholar
Balakumar, P. & Reed, H. 1991 Stability of three-dimensional supersonic boundary layers. Phys. Fluids A 3, 617–632.Google Scholar
Bartkowicz, M. D., Subbareddy, P. K. & Candler, G. V.2010 Simulation of boundary layer transition of elliptic cones in hypersonic flow. AIAA Paper 2010-1064.Google Scholar
Bertolotti, F. P.2000 On the connection between cross-flow vortices and attachment–line instabilities. In Proceedings of the IUTAM Laminar-Turbulent Symposium V, Sedona, AZ, USA (ed. W. Saric & H. Fasel), pp. 625–630 Springer.Google Scholar
Borg, M. P., Kimmel, R. L. & Stanfield, S.2011 HIFiRE-5 attachment-line and crossflow instabilities in a quiet hypersonic wind tunnel. AIAA Paper 2011-3247.Google Scholar
Borg, M. P., Kimmel, R. L. & Stanfield, S.2012 Crossflow instability for HIFiRE-5 in a quiet hypersonic wind tunnel. AIAA Paper 2012-2821.CrossRefGoogle Scholar
Borg, M. P., Kimmel, R. L. & Stanfield, S. 2015 Traveling crossflow instability for the HIFiRE-5 elliptic cone. J. Spacecr. Rockets 52, 664–673.Google Scholar
Bridges, T. J. & Morris, P. J. 1984 Differential eigenvalue problems in which the parameter appears nonlinearly. J. Comput. Phys. 55, 437–460.Google Scholar
Chang, C.-L.2004 LASTRAC.3d: Transition prediction in 3D boundary layers. AIAA Paper 2004-2542.CrossRefGoogle Scholar
Choudhari, M., Chang, C.-L., Jentink, T., Li, F., Berger, K., Candler, G. & Kimmel, R.2009 Transition analysis for the HIFiRE-5 vehicle. AIAA Paper 2009-4056.Google Scholar
De Tullio, N., Paredes, P., Sandham, N. D. & Theofilis, V. 2013 Roughness-induced instability and breakdown to turbulence in a supersonic boundary-layer. J. Fluid Mech. 735, 613–646.Google Scholar
Dinzl, D. J. & Candler, G. V.2015 Analysis of crossflow instability on HIFiRE-5 using direct numerical simulations. AIAA Paper 2015-0279.Google Scholar
Fedorov, A. V. 2011 Transition and stability of high-speed boundary layers. Annu. Rev. Fluid Mech. 43, 79–95.Google Scholar
Gosse, R., Kimmel, R. & Johnson, H.2010 CFD study of the HIFiRE-5 flight experiment. AIAA Paper 2010-4854.CrossRefGoogle Scholar
Gosse, R., Kimmel, R. & Johnson, H. B. 2014 Study of boundary-layer transition on Hypersonic International Flight Research Experimentation 5. J. Spacecr. Rockets 3, 617–632.Google Scholar
Herbert, T. 1997 Parabolized stability equations. Annu. Rev. Fluid Mech. 29, 245–283.Google Scholar
Hermanns, M. & Hernández, J. A. 2008 Stable high-order finite-difference methods based on non-uniform grid point distributions. Intl J. Numer. Meth. Fluids 56, 233–255.Google Scholar
Huntley, M. B.2000 Transition on elliptic cones at Mach 8. PhD thesis, Princeton University.Google Scholar
Huntley, M. & Smits, A. J. 2000 Transition studies on an elliptic cone in Mach 8 flow using filtered Rayleigh scattering. Eur. J. Mech. (B/Fluids) 19 (5), 695–706.Google Scholar
Johnson, H. & Candler, G.2010 Three-dimensional hypersonic boundary layer instability analysis with STABL-3D. AIAA Paper 2010-5005.Google Scholar
Juliano, T. J., Adamczak, D. & Kimmel, R. L.2014 HIFiRE-5 flight test heating analysis. AIAA Paper 2014-0076.Google Scholar
Juliano, T. J., Adamczak, D. & Kimmel, R. L. 2015a HIFiRE-5 flight test results. J. Spacecr. Rockets 52 (3), 650–663.Google Scholar
Juliano, T. J., Borg, M. P. & Schneider, S. P. 2015b Quiet tunnel measurements of HIFiRE-5 boundary-layer transition. AIAA J. 53 (4), 832–846.Google Scholar
Juliano, T. J. & Schneider, S. P.2010 Instability and transition on the HIFiRE-5 in a Mach-6 quiet tunnel. AIAA Paper 2010-5004.Google Scholar
Kimmel, R., Adamczak, D., Berger, K. & Choudhari, M.2010 HIFiRE-5 flight vehicle design. AIAA Paper 2010-4985.Google Scholar
Kimmel, R. L., Adamczak, D. & Juliano, T. J.2013 HIFiRE-5 flight test preliminary results. AIAA Paper 2013-0377.Google Scholar
Kroonenberg, A., Radespiel, R., Candler, G. & Estorf, M.2010 Infrared measurements of boundary-layer transition on an inclined cone at Mach 6. AIAA Paper 2010-1063.Google Scholar
Li, F. & Choudhari, M. 2011 Spatially developing secondary instabilities in compressible swept airfoil boundary layers. Theor. Comput. Fluid Dyn. 25, 65–84.CrossRefGoogle Scholar
Li, F., Choudhari, M., Chang, C. L., Kimmel, R., Adamczak, D. & Smith, M. 2015 Transition analysis for the ascent phase of HIFiRE-1 flight experiment. J. Spacecr. Rockets 52 (5), 1283–1293.Google Scholar
Li, F., Choudhari, M., Chang, C., White, J., Kimmel, R., Adamczak, D., Borg, M., Stanfield, S. & Smith, M.2012 Stability analysis for HIFiRE experiments. AIAA Paper 2012-2961.Google Scholar
Mack, C. J., Schmid, P. J. & Sesterhenn, J. L. 2008 Global stability of swept flow around a parabolic body: connecting attachment-line and crossflow modes. J. Fluid Mech. 611, 205–214.Google Scholar
Mack, L. M.1984 Boundary layer linear stability theory. In AGARD–R–709 Special Course on Stability and Transition of Laminar Flow, pp. 3.1–3.81.Google Scholar
Malik, M. R., Li, F. & Choudhari, M.2007 Analysis of crossflow transition flight experiment aboard Pegasus launch vehicle. AIAA 2007-4487.CrossRefGoogle Scholar
Mughal, M. S.2006 Stability analysis of complex wing geometries: parabolised stability equations in generalized non-orthogonal coordinates. AIAA Paper 2006-3222.Google Scholar
Nompelis, I., Drayna, T. & Candler, G.2005 A parallel unstructured implicit solver for hypersonic reacting flow simulation. AIAA Paper 2005-4867.Google Scholar
Paredes, P.2014 Advances in global instability computations: from incompressible to hypersonic flow. PhD thesis, Universidad Politécnica de Madrid.Google Scholar
Paredes, P., Hanifi, A., Theofilis, V. & Henningson, D. 2015 The nonlinear PSE-3D concept for transition prediction in flows with a single slowly-varying spatial direction. Proc. IUTAM 14C, 35–44.Google Scholar
Paredes, P., Hermanns, M., Le Clainche, S. & Theofilis, V. 2013 Order 104 speedup in global linear instability analysis using matrix formation. Comput. Meth. Appl. Mech. Engng 253, 287–304.Google Scholar
Paredes, P., Perez, E., Reed, H. L. & Theofilis, V.2014 High-frequency instabilities along the windward face of a hypersonic yawed circular cone. AIAA Paper 2014-2774.Google Scholar
Paredes, P. & Theofilis, V. 2015 Centerline instabilities on the Hypersonic International Flight Research Experimentation HIFiRE-5 elliptic cone model. J. Fluids Struct. 53, 36–49.Google Scholar
Perez, E., Reed, H. L. & Kuehl, J. J.2012 Instabilities on a hypersonic yawed straight cone. AIAA Paper 2013-2879.Google Scholar
Poggie, J. & Kimmel, R. L.1998 Traveling instabilities in elliptic cone boundary-layer transition at $Ma=8$ . AIAA Paper 98-0435.Google Scholar
Reed, H. L. & Saric, W. S. 1989 Stability of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 21, 235–284.Google Scholar
Reed, H. L., Saric, W. S. & Arnal, D. 1996 Linear stability theory applied to boundary layers. Annu. Rev. Fluid Mech. 28, 389–428.Google Scholar
Saad, Y. 1980 Variations of Arnoldi’s method for computing eigenelements of large unsymmetric matrices. Linear Algebr. Applics 34, 269–295.Google Scholar
Saric, W. S., Reed, H. L. & White, E. B. 2003 Stability and transition of three-dimensional boundary layers. Annu. Rev. Fluid Mech. 35, 413–440.Google Scholar
Schmisseur, J. D., Schneider, S. P. & Collicott, S. H.1998 Receptivity of the Mach-4 boundary-layer on an elliptic cone to laser-generated localized free stream perturbations. AIAA Paper 98-0532.CrossRefGoogle Scholar
Schmisseur, J. D., Schneider, S. P. & Collicott, S. H.1999 Response of the Mach-4 boundary layer on an elliptic cone to laser-generated free stream perturbations. AIAA Paper 99-0410.Google Scholar
Schneider, S. P. 2004 Hypersonic laminar-turbulent transition on circular cones and scramjet forebodies. Prog. Aeronaut. Sci. 40, 1–50.Google Scholar
Schneider, S. P. 2008 Development of hypersonic quiet tunnels. J. Spacecr. Rockets 45 (4), 641–664.Google Scholar
Schneider, S. P. 2015 Developing mechanism-based methods for estimating hypersonic boundary-layer transition in flight: the role of quiet tunnels. Prog. Aeronaut. Sci. 72, 17–29.Google Scholar
Stetson, K. & Kimmel, R. L.1992 On hypersonic boundary-layer stability. AIAA Paper 1992-0737.CrossRefGoogle Scholar
Theofilis, V. 1995 Spatial stability of incompressible attachment line flow. Theor. Comput. Fluid Dyn. 7, 159–171.Google Scholar
Theofilis, V.2002 Inviscid global instability of compressible flow over an elliptic cylinder: algorithmic developments. Tech. Rep. F61775-00-WE069. Final Report, European Office of Aerospace Research and Development, available at http://www.dtic.mil/cgi-bin/GetTRDoc?AD=ADA406446&Location=U2&doc=GetTRDoc.pdf.Google Scholar
Theofilis, V. 2003 Advances in global linear instability of nonparallel and three-dimensional flows. Prog. Aeronaut. Sci. 39 (4), 249–315.Google Scholar
Theofilis, V. 2011 Global linear instability. Annu. Rev. Fluid Mech. 43, 319–352.Google Scholar
Wilkinson, J. H. 1965 The Algebraic Eigenvalue Problem. Clarendon.Google Scholar