Article contents
Mach reflection of three-dimensional curved shock waves on V-shaped blunt leading edges
Published online by Cambridge University Press: 22 November 2023
Abstract
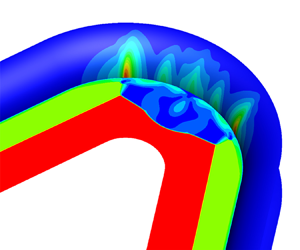
Theoretical investigation of the primary Mach reflection (MR) configuration on V-shaped blunt leading edges (VBLEs) forms the focus of this study. By ignoring the secondary interactions, a theoretical method based on a simplified form of the continuity relation is developed to predict the shock configurations, including the detached shock, the Mach stem, the transmitted shock and the triple point. The comparison of the theoretical results with both numerical and previous experimental results shows the reliability of the theoretical approach in predicting shock structures across a wide range of free stream and geometric parameters. The theoretical model provides a detailed comprehension of the occurrence mechanism of inverse MRs on VBLEs and the influence of the free stream and geometric parameters on primary MR configurations. Along with the primary MR configuration, the curved shock or compression waves generated by the crotch are solved and offer insight into the transition from the MR to the regular reflection from the same family (sRR). The increase of the ratio  $R/r$ and the free stream Mach number
$R/r$ and the free stream Mach number  $M_0$ appears to facilitate the transition, while the effect of the half-span angle
$M_0$ appears to facilitate the transition, while the effect of the half-span angle  $\beta$ is non-monotonic. The predicted shock positions allow for the identification of the transition boundary between the primary MR and sRR. It is found that
$\beta$ is non-monotonic. The predicted shock positions allow for the identification of the transition boundary between the primary MR and sRR. It is found that  $R/r$ below a threshold (for a set
$R/r$ below a threshold (for a set  $M_0$ value) produces MR, irrespective of
$M_0$ value) produces MR, irrespective of  $\beta$. If this threshold is exceeded, the configuration can transition from the primary MR to sRR and then back to the primary MR as
$\beta$. If this threshold is exceeded, the configuration can transition from the primary MR to sRR and then back to the primary MR as  $\beta$ increases.
$\beta$ increases.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 5
- Cited by


