Hostname: page-component-78c5997874-t5tsf Total loading time: 0 Render date: 2024-11-11T00:24:07.421Z Has data issue: false hasContentIssue false
  • English
  • Français

Mechanism of detonation stabilization in a supersonic model combustor

Published online by Cambridge University Press:  18 January 2021

Xiaodong Cai Open the ORCID record for Xiaodong Cai [Opens in a new window] ,
Ralf Deiterding Open the ORCID record for Ralf Deiterding [Opens in a new window] ,
Jianhan Liang ,
Yasser Mahmoudi  and
Mingbo Sun
Xiaodong Cai
Affiliation:
Science and Technology on Scramjet Laboratory, National University of Defense Technology, Changsha410073, PR China
Ralf Deiterding
Affiliation:
Aerodynamics and Flight Mechanics Research Group, University of Southampton, Boldrewood Campus, SouthamptonSO16 7QF, UK
Jianhan Liang*
Affiliation:
Science and Technology on Scramjet Laboratory, National University of Defense Technology, Changsha410073, PR China
Yasser Mahmoudi
Affiliation:
School of Mechanical and Aerospace Engineering, Queen's University Belfast, BelfastBT9 5AH, UK
Mingbo Sun*
Affiliation:
Science and Technology on Scramjet Laboratory, National University of Defense Technology, Changsha410073, PR China
*
† Email addresses for correspondence: jhleon@vip.sina.com, xd.cai.aero@outlook.com
† Email addresses for correspondence: jhleon@vip.sina.com, xd.cai.aero@outlook.com
Get access Rights & Permissions [Opens in a new window]

Abstract

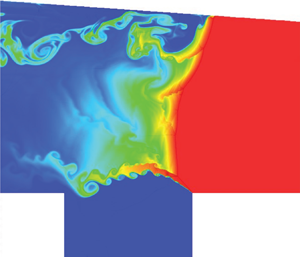
The present work studies numerically the quasi-steady propagation of a hydrogen/oxygen detonation in a supersonic model combustor consisting of a cavity and an expanding wall. The two-dimensional reactive compressible Navier–Stokes equations with a one-step and two-species reaction model are solved using a hybrid sixth-order weighted essentially non-oscillatory-centred difference scheme combined with a structured adaptive mesh refinement technique. The results show that, after the shutdown of the hot jet, the detonation wave is successfully stabilized quasi-steadily in the supersonic model combustor together with periodic fluctuations of the detonation front. The formation of the quasi-steady propagation of detonation in the model combustor is mainly due to the combined effects of (i) pressure oscillations generated in the cavity, which facilitate the detonation propagation, and (ii) lateral mass divergence brought by the expanding wall, which can lead to detonation attenuation, and an unburned jet associated with large-scale vortices resulting from a Prandtl–Meyer expansion fan. This expansion fan is generated because of the expanding wall, which can contribute to the detonation stabilization. It is found that, for an incoming velocity lower than the Chapman–Jouguet value, a quasi-steady propagation of the detonation wave cannot be achieved. However, for incoming velocity higher than the Chapman–Jouguet value, a stabilization can be realized. This is effectively due to the formation of a periodic process, including four stages of forward propagation, detonation attenuation, backward propagation and detonation bifurcation, indicating the influence of the supersonic model combustor on the overall process.

JFM classification

Compressible Flows: Detonation waves Reacting Flows: Detonations
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 910 , 10 March 2021 , A40
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bane, S. P. M., Ziegler, J. L. & Shepherd, J. E. 2010 Development of one-step chemistry models for flame and ignition simulation. Report No. GALCITFM: 2010.002. California Institute of Technology, pp. 1–53.Google Scholar
Berger, M. & Olier, J. 1984 Adaptive mesh refinement for hyperbolic partial differential equations. J. Comput. Phys. 53, 484–512.CrossRefGoogle Scholar
Cai, X. D., Deiterding, R., Liang, J. H., Lin, Z. Y. & Sun, M. B. 2018 a Detonation interaction with cavity in supersonic combustible mixture. AIAA J. 56, 2096–2102.CrossRefGoogle Scholar
Cai, X. D., Deiterding, R., Liang, J. H. & Mahmoudi, Y. 2017 Adaptive simulations of viscous detonations initiated by a hot jet using a high-order hybrid WENO-CD scheme. Proc. Combust. Inst. 36, 2725–2733.CrossRefGoogle Scholar
Cai, X. D., Deiterding, R., Liang, J. H., Sun, M. B. & Dong, D. Z. 2019 Dynamic detonation stabilization in supersonic expanding channels. Phys. Rev. Fluids 4, 083201.CrossRefGoogle Scholar
Cai, X. D., Deiterding, R., Liang, J. H., Sun, M. B. & Mahmoudi, Y. 2018 b Diffusion and mixing effects in hot jet initiation and propagation of hydrogen detonations. J. Fluid Mech. 836, 324–351.CrossRefGoogle Scholar
Cai, X. D., Liang, J. H., Deiterding, R., Mahmoudi, Y. & Sun, M. B. 2018 c Experimental and numerical investigations on propagating modes of detonations: detonation wave/boundary layer interaction. Combust. Flame 190, 201–215.CrossRefGoogle Scholar
Deiterding, R. 2003 Parallel adaptive simulation of multi-dimensional detonation structures. PhD thesis, Technical University of Cottbus, Cottbus, Germany.Google Scholar
Deiterding, R. 2009 A parallel adaptive method for simulating shock-induced combustion with detailed chemical kinetics in complex domains. Comput. Struct. 87, 769–783.CrossRefGoogle Scholar
Dimotakis, P. E. 2005 Turbulent mixing. Annu. Rev. Fluid Mech. 37, 329–356.CrossRefGoogle Scholar
Gamezo, V. N., Ogawa, T. & Oran, E. S. 2007 Numerical simulations of flame propagation and DDT in obstructed channels filled with hydrogen–air mixture. Proc. Combust. Inst. 31, 2463–2471.CrossRefGoogle Scholar
Gottlieb, S., Ketcheson, D. I. & Shu, C. W. 2009 High order strong stability preserving time discretizations. J. Sci. Comput. 38, 251.CrossRefGoogle Scholar
Grogan, K. P. & Ihme, M. 2015 Weak and strong ignition of hydrogen/oxygen mixtures in shock-tube systems. Proc. Combust. Inst. 35, 2181–2189.CrossRefGoogle Scholar
Hill, D. J. & Pullin, D. I. 2004 Hybrid tuned center-difference-WENO method for large eddy simulations in the presence of strong shocks. J. Comput. Phys. 194, 435–450.CrossRefGoogle Scholar
Kailasanath, K. 2008 Review of propulsion applications of detonation waves. AIAA J. 38, 1698–1708.CrossRefGoogle Scholar
Kang, S. H., Lee, Y. J., Yang, S. S., Smart, M. K. & Suraweera, M. K. 2011 Cowl and cavity effects on mixing and combustion in scramjet engines. J. Propul. Power 27, 1169–1177.CrossRefGoogle Scholar
Kao, S. & Shepherd, J. E. 2008 Numerical solution methods for control volume explosions and ZND detonation structure. GALCIT Rep. FM2006.007. California Institute of Technology.Google Scholar
Kaps, P. & Rentrop, P. 1979 Generalized Runge–Kutta methods of order four with step size control for stiff ordinary differential equations. Numer. Math. 33, 55–68.CrossRefGoogle Scholar
Lombardini, M. 2008 Richtmyer-Meshkov instability in converging geometries. PhD thesis, California Institute of Technology, California.Google Scholar
Lu, F. K. & Braun, E. M. 2014 Rotating detonation wave propulsion: experimental challenges, modeling, and engine concepts. J. Propul. Power 30, 1125–1142.CrossRefGoogle Scholar
Mahmoudi, Y., Karimi, N., Deiterding, R. & Emami, S. 2014 Hydrodynamic instabilities in gaseous detonations: comparison of Euler, Navier–Stokes, and large-Eddy simulation. J. Propul. Power 30, 384–396.CrossRefGoogle Scholar
Massa, L., Austin, J. M. & Jackson, T. L. 2007 Triple-point shear layers in gaseous detonation waves. J. Fluid Mech. 586, 205–248.CrossRefGoogle Scholar
Maxwell, B. M., Bhattacharjee, R. R., Lau-Chapdelaine, S. S. M., Falle, S. A. E. G., Sharpe, G. J. & Radulescu, M. I. 2017 Influence of turbulent fluctuations on detonation propagation. J. Fluid Mech. 818, 646–696.CrossRefGoogle Scholar
Mazaheri, K., Mahmoudi, Y. & Radulescu, M. I. 2012 Diffusion and hydrodynamic instabilities in gaseous detonations. Combust. Flame 113, 2138–2154.CrossRefGoogle Scholar
Oran, E. S. & Gamezo, V. N. 2007 Origins of the deflagration-to-detonation transition in gas-phase combustion. Combust. Flame 148, 4–47.CrossRefGoogle Scholar
Pantano, C., Deiterding, R., Hill, D. J. & Pullin, D. I. 2007 A low numerical dissipation patch-based adaptive mesh refinement method for large-eddy simulation of compressible flows. J. Comput. Phys. 221, 63–87.CrossRefGoogle Scholar
Peng, H., Huang, Y., Deiterding, R., Luan, Z., Xing, F. & You, Y. 2018 Effects of jet in crossflow on flame acceleration and deflagration to detonation transition in methane-oxygen mixture. Combust. Flame 198, 69–80.CrossRefGoogle Scholar
Potturi, A. S. & Edwards, J. R. 2015 Large-eddy/Reynolds-averaged Navier–Stokes simulation of cavity-stabilized ethylene combustion. Combust. Flame 162, 1176–1192.CrossRefGoogle Scholar
Radulescu, M. I. 2018 A detonation paradox: why inviscid detonation simulations predict the incorrect trend for the role of instability in gaseous cellular detonations. Combust. Flame 195, 151–162.CrossRefGoogle Scholar
Radulescu, M. I. & Borzou, B. 2018 Dynamics of detonations with a constant mean flow divergence. J. Fluid Mech. 845, 346–377.CrossRefGoogle Scholar
Radulescu, M. I., Sharpe, G. J., Law, C. K. & Lee, J. H. S. 2007 The hydrodynamic structure of unstable cellular detonations. J. Fluid Mech. 580, 31–81.CrossRefGoogle Scholar
Radulescu, M. I., Sharpe, G. J., Lee, J. H. S., Kiyanda, C. B., Higgins, A. J. & Hanson, R. K. 2005 The ignition mechanism in irregular structure gaseous detonations. Proc. Combust. Inst. 30 (2), 1859–1867.CrossRefGoogle Scholar
Romick, C. M., Aslam, T. D. & Powers, J. M. 2012 The effect of diffusion on the dynamics of unsteady detonations. J. Fluid Mech. 699, 453–464.CrossRefGoogle Scholar
Shen, H. & Parsani, M. 2017 The role of multidimensional instabilities in direct initiation of gaseous detonations in free space. J. Fluid Mech. 813, R4.CrossRefGoogle Scholar
Shi, X. F., Zhu, Y. J., Yang, J. M. & Luo, X. S. 2019 Mach stem deformation in pseudo-steady shock wave reflections. J. Fluid Mech. 861, 407–421.CrossRefGoogle Scholar
Sun, M. B., Wang, Z. G., Liang, J. H. & Geng, H. 2008 Flame characteristics in cupersonic combustor with hydrogen injection upstream of cavity flameholder. J. Propul. Power 24, 688–696.CrossRefGoogle Scholar
Tatman, B. J., Rockwell, R. D., Goyne, C. P., Mcdanile, J. C. & Donohue, J. M. 2013 Experimental study of vitiation effects on flameholding in a cavity flameholder. J. Propul. Power 29, 417–423.CrossRefGoogle Scholar
Teng, H. H., Jiang, Z. L. & Ng, H. D. 2014 Numerical study on unstable surfaces of oblique detonations. J. Fluid Mech. 744, 111–128.CrossRefGoogle Scholar
Wang, Y., Han, W., Deiterding, R. & Chen, Z. 2018 Effects of disturbance on detonation initiation in H2–O2–N2 mixture. Phys. Rev. Fluids 3, 123201.CrossRefGoogle Scholar
Wolański, P. 2013 Detonative propulsion. Proc. Combust. Inst. 34, 125–158.CrossRefGoogle Scholar
Xiao, H. H. & Oran, E. S. 2019 Shock focusing and detonation initiation at a flame front. Combust. Flame 203, 397–406.CrossRefGoogle Scholar
Yeom, H. W., Seo, B. G. & Sung, H. G. 2013 Numerical analysis of a scramjet engine with intake sidewalls and cavity flameholder. AIAA J. 51, 1566–1575.CrossRefGoogle Scholar
Zhang, X. J., Wei, H. Q., Zhou, L., Cai, X. D. & Deiterding, R. 2020 Relationship of flame propagation and combustion mode transition of end-gas based on pressure wave in confined space. Combust. Flame 214, 371–386.CrossRefGoogle Scholar
Ziegler, J. L., Deiterding, R., Shepherd, J. E. & Pullin, D. I. 2011 An adaptive high-order hybrid scheme for compressive, viscous flows with detailed chemistry. J. Comput. Phys. 230, 7598–7630.CrossRefGoogle Scholar