Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-13T13:19:34.072Z Has data issue: false hasContentIssue false
  • English
  • Français

Motion of self-rewetting drop on a substrate with a constant temperature gradient

Published online by Cambridge University Press:  29 March 2021

Ze-Lai Xu ,
Jun-Yuan Chen ,
Hao-Ran Liu Open the ORCID record for Hao-Ran Liu [Opens in a new window] ,
Kirti Chandra Sahu Open the ORCID record for Kirti Chandra Sahu [Opens in a new window]  and
Hang Ding Open the ORCID record for Hang Ding [Opens in a new window]
Ze-Lai Xu
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
Jun-Yuan Chen
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
Hao-Ran Liu
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
Kirti Chandra Sahu*
Affiliation:
Department of Chemical Engineering, Indian Institute of Technology Hyderabad, Sangareddy, 502 285 Telangana, India
Hang Ding*
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei230026, PR China
*
†Email addresses for correspondence: ksahu@che.iith.ac.in, hding@ustc.edu.cn
†Email addresses for correspondence: ksahu@che.iith.ac.in, hding@ustc.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

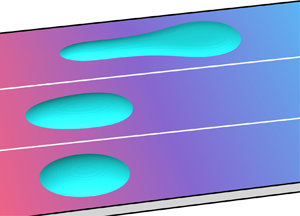
We investigate the dynamics of a self-rewetting drop placed on a substrate with a constant temperature gradient via three-dimensional numerical simulations using a conservative level-set approach to track the interface of the drop. The surface tension of a so-called self-rewetting fluid exhibits a parabolic dependence on temperature with a well-defined minimum. Two distinct drop behaviours, namely deformation and elongation, are observed when it is placed at the location of the minimum surface tension. The drop spreads slightly and reaches a pseudo-steady state in the deformation regime, while it continuously spreads until breakup in the elongation regime. Theoretical models based on the forces exerted on the drop have been developed to predict the critical condition at which the drop undergoes the transition between the two regimes, and the predictions are in good agreement with the numerical results. We also investigate the effect of the initial position of the drop with respect to the location of the minimum surface tension on the spreading and migration dynamics. It is found that, at early times, the migration of the drop obeys an exponential function with time, but it diverges at the later stage due to an increase in the drop deformation.

JFM classification

Drops and Bubbles: Drops Drops and Bubbles: Thermocapillarity Interfacial Flows (free surface): Contact lines
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 915 , 25 May 2021 , A116
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Balla, M., Tripathi, M.K., Sahu, K.C., Karapetsas, G. & Matar, O.K. 2019 Non-isothermal bubble rise dynamics in a self-rewetting fluid: three-dimensional effects. J. Fluid Mech. 858, 689–713.CrossRefGoogle Scholar
Bonn, D., Eggers, J., Indekeu, J., Meunier, J. & Rolley, E. 2009 Wetting and spreading. Rev. Mod. Phys. 81 (2), 739–805.CrossRefGoogle Scholar
Bouasse, H. 1924 Capillarite: Phenomenes Superficiels. Librairie Delgrave.Google Scholar
Brochard, F. 1989 Motions of droplets on solid surfaces induced by chemical or thermal gradients. Langmuir 5 (2), 432–438.CrossRefGoogle Scholar
Brzoska, J.B., Brochard-Wyart, F. & Rondelez, F. 1993 Motions of droplets on hydrophobic model surfaces induced by thermal gradients. Langmuir 9 (8), 2220–2224.CrossRefGoogle Scholar
Chaudhury, K. & Chakraborty, S. 2015 Spreading of a droplet over a non-isothermal substrate: multiple scaling regimes. Langmuir 31 (14), 4169–4175.CrossRefGoogle Scholar
Chen, J.Z., Troian, S.M., Darhuber, A.A. & Wagner, S. 2005 Effect of contact angle hysteresis on thermocapillary droplet actuation. J. Appl. Phys. 97 (1), 014906.CrossRefGoogle Scholar
Chiu, P.H. & Lin, Y.T. 2011 A conservative phase field method for solving incompressible two-phase flows. J. Comput. Phys. 230, 185–204.CrossRefGoogle Scholar
Ding, H. & Spelt, P.D.M. 2007 Wetting condition in diffuse interface simulations of contact line motion. Phys. Rev. E 75, 046708.CrossRefGoogle ScholarPubMed
Ding, H., Spelt, P.D.M. & Shu, C. 2007 Diffuse interface model for incompressible two-phase flows with large density ratios. J. Comput. Phys. 226, 2078–2095.CrossRefGoogle Scholar
Fath, A. & Bothe, D. 2015 Direct numerical simulations of thermocapillary migration of a droplet attached to a solid wall. Intl J. Multiphase Flow 77 (1), 209–221.CrossRefGoogle Scholar
Ford, M.L. & Nadim, A. 1994 Thermocapillary migration of an attached drop on a solid surface. Phys. Fluids 6 (9), 3183–3185.CrossRefGoogle Scholar
de Gennes, P.G. 1985 Wetting: statics and dynamics. Rev. Mod. Phys. 57 (3), 827.CrossRefGoogle Scholar
Gomba, J.M. & Homsy, G.M. 2010 Regimes of thermocapillary migration of droplets under partial wetting conditions. J. Fluid Mech. 647, 125–142.CrossRefGoogle Scholar
Gurrala, P., Katre, P., Balusamy, S., Banerjee, S. & Sahu, K.C. 2019 Evaporation of ethanol-water sessile droplet of different compositions at an elevated substrate temperature. Intl J. Heat Mass Transfer 145, 118770.CrossRefGoogle Scholar
Huh, C. & Scriven, L. 1971 Hydrodynamic model of steady movement of a solid/liquid/fluid contact line. J. Colloid Interface Sci. 35 (1), 85–101.CrossRefGoogle Scholar
Karapetsas, G., Sahu, K.C. & Matar, O.K. 2013 Effect of contact line dynamics on the thermocapillary motion of a droplet on an inclined plate. Langmuir 29, 8892–8906.CrossRefGoogle Scholar
Karapetsas, G., Sahu, K.C., Sefiane, K. & Matar, O.K 2014 Thermocapillary-driven motion of a sessile drop: effect of non-monotonic dependence of surface tension on temperature. Langmuir 30 (15), 4310–4321.CrossRefGoogle ScholarPubMed
Kim, J. 2005 A continuous surface tension force formulation for diffuse-interface models. J. Comput. Phys. 204, 784–804.CrossRefGoogle Scholar
Kumar, M, Bhardwaj, R. & Sahu, K.C. 2020 Wetting dynamics of a water droplet on micro-pillar surfaces with radially varying pitches. Langmuir 36 (19), 5312–5323.CrossRefGoogle Scholar
Liu, H., Valocchi, A.J., Zhang, Y. & Kang, Q. 2013 Phase-field-based lattice Boltzmann finite-difference model for simulating thermocapillary flows. Phys. Rev. E 87, 013010.CrossRefGoogle ScholarPubMed
Olsson, E. & Kreiss, G. 2005 A conservative level set method for two phase flow. J. Comput. Phys. 210, 225–246.CrossRefGoogle Scholar
Pratap, V., Moumen, N. & Subramanian, R.S. 2008 Thermocapillary motion of a liquid drop on a horizontal solid surface. Langmuir 24 (9), 5185–5193.CrossRefGoogle ScholarPubMed
Randive, P., Dalal, A., Sahu, K.C., Biswas, G. & Mukherjee, P.P. 2015 Wettability effects on contact line dynamics of droplet motion in an inclined channel. Phys. Rev. E 91 (5), 053006.CrossRefGoogle Scholar
Renardy, M., Renardy, Y. & Li, J. 2001 Numerical simulation of moving contact line problems using a volume-of-fluid method. J. Comput. Phys. 171 (1), 243–263.CrossRefGoogle Scholar
Savino, R., di Francescantonio, N., Fortezza, R. & Abe, Y. 2007 Heat pipes with binary mixtures and inverse Marangoni effects for microgravity applications. Acta Astronaut. 61 (1–6), 16–26.CrossRefGoogle Scholar
Tripathi, M.K., Sahu, K.C., Karapetsas, G., Sefiane, K. & Matar, O.K. 2015 Non-isothermal bubble rise: non-monotonic dependence of surface tension on temperature. J. Fluid Mech. 763, 82–108.CrossRefGoogle Scholar
Tryggvason, G., Scardovelli, R. & Zaleski, S. 2011 Direct Numerical Simulations of Gas–Liquid Multiphase Flows. Cambridge University Press.Google Scholar
Tsang, S., Wu, Z.H., Lin, C.H. & Sun, C.L. 2018 On the evaporative spray cooling with a self-rewetting fluid: chasing the heat. Appl. Therm. Engng 132, 196–208.CrossRefGoogle Scholar
Vochten, R. & Petre, G. 1973 Study of the heat of reversible adsorption at the air-solution interface. II. Experimental determination of the heat of reversible adsorption of some alcohols. J. Colloid Interface Sci. 42 (2), 320–327.CrossRefGoogle Scholar
Wu, S.C. 2015 Study of self-rewetting fluid applied to loop heat pipe. Intl J. Therm. Sci. 98, 374–380.CrossRefGoogle Scholar
Yi, S. 2014 Moving towards the cold region or the hot region? Thermocapillary migration of a droplet attached on a horizontal substrate. Phys. Fluids 26 (9), 3183–3185.Google Scholar