Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-13T01:33:20.043Z Has data issue: false hasContentIssue false
  • English
  • Français

Multiscale dynamic wetting of a droplet on a lyophilic pillar-arrayed surface

Published online by Cambridge University Press:  25 January 2013

Quanzi Yuan  and
Ya-Pu Zhao
Quanzi Yuan
Affiliation:
State Key Laboratory of Nonlinear Mechanics, Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, People’s Republic of China
Ya-Pu Zhao*
Affiliation:
State Key Laboratory of Nonlinear Mechanics, Institute of Mechanics, Chinese Academy of Sciences, Beijing 100190, People’s Republic of China
*
†Email address for correspondence: yzhao@imech.ac.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

Dynamic wetting of a droplet on lyophilic pillars was explored using a multiscale combination method of experiments and molecular dynamics simulations. The excess lyophilic area not only provided excess driving force, but also pinned the liquid around the pillars, which kept the moving contact line in a dynamic balance state every period of the pillars. The flow pattern and the flow field of the droplet on the pillar-arrayed surface, influenced by the concerted effect of the liquid–solid interactions and the surface roughness, were revealed from the continuum to the atomic level. Then, the scaling analysis was carried out employing molecular kinetic theory. Controlled by the droplet size, the density of roughness and the pillar height, two extreme regimes were distinguished, i.e. $R\sim {t}^{1/ 3} $ for the rough surface and 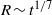$R\sim {t}^{1/ 7} $ for the smooth surface. The scaling laws were validated by both the experiments and the simulations. Our results may help in understanding the dynamic wetting of a droplet on a pillar-arrayed lyophilic substrate and assisting the future design of pillar-arrayed lyophilic surfaces in practical applications.

JFM classification

Interfacial Flows (free surface): Contact lines Drops and Bubbles: Drops Micro-/Nano-fluid dynamics: Micro-/Nano-fluid dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 716 , 10 February 2013 , pp. 171 - 188
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berendsen, H. J. C., Grigera, J. R. & Straatsma, T. P. 1987 The missing term in effective pair potentials. J. Phys. Chem. 91, 6269–6271.Google Scholar
Blake, T. D. 1993 Wettability. Dekker.Google Scholar
Blake, T. D. 2006 The physics of moving wetting lines. J. Colloid Interface Sci. 299, 1–13.Google Scholar
Blake, T. D. & De Coninck, J. 2002 The influence of solid–liquid interactions on dynamic wetting. Adv. Colloid Interface Sci. 96, 21–36.Google Scholar
Bonn, D., Eggers, J., Indekeu, J., Meunier, J. & Rolley, E. 2009 Wetting and spreading. Rev. Mod. Phys. 81, 739–805.Google Scholar
Concus, P. & Finn, R. 1969 On the behaviour of a capillary surface in a wedge. Proc. Natl Acad. Sci. USA 63, 292–299.Google Scholar
Courbin, L., Denieul, E., Dressaire, E., Roper, M., Ajdari, A. & Stone, H. A. 2007 Imbibition by polygonal spreading on microdecorated surfaces. Nat. Mater. 6, 661–664.Google Scholar
Cox, R. 1986 The dynamics of the spreading of liquids on a solid surface. Part 1. Viscous flow. J. Fluid Mech. 168, 169–194.Google Scholar
De Coninck, J. & Blake, T. D. 2008 Wetting and molecular dynamics simulations of simple liquids. Annu. Rev. Mater. Res. 38, 1–22.Google Scholar
Derjaguin, B. V., Churaev, N. V. & Muller, V. M. 1987 Surface Forces. Consultants Bureau.Google Scholar
Dussan, V. E. B. 1976 The moving contact line: the slip boundary condition. J. Fluid Mech. 77, 665–684.Google Scholar
Dussan, V. E. B. 1979 On the spreading of liquids on solid-surfaces: static and dynamic contact lines. Annu. Rev. Fluid Mech. 11, 371–400.CrossRefGoogle Scholar
Fetzer, R., Ramiasa, M. & Ralston, J. 2009 Dynamics of liquid–liquid displacement. Langmuir 25, 8069–8074.Google Scholar
Fu, J., Mao, P. & Han, J. 2009 Continuous-flow bioseparation using microfabricated anisotropic nanofluidic sieving structures. Nat. Protoc. 4, 1681–1698.Google Scholar
de Gennes, P. G. 1985 Wetting: statics and dynamics. Rev. Mod. Phys. 57, 827–863.Google Scholar
Glasstone, S., Laidler, K. & Eyring, H. 1941 The Theory of Rate Processes. McGraw-Hill.Google Scholar
González, M. A. & Abascal, J. L. F. 2010 The shear viscosity of rigid water models. J. Chem. Phys. 132, 096101.Google Scholar
Greenspan, H. 1978 On the motion of a small viscous droplet that wets a surface. J. Fluid Mech. 84, 125–143.Google Scholar
Hasimoto, H. 1959 On the periodic fundamental solutions of the Stokes equations and their application to viscous flow past a cubic array of spheres. J. Fluid Mech. 5, 317–328.Google Scholar
Hocking, L. 1976 A moving fluid interface on a rough surface. J. Fluid Mech. 76, 801–817.Google Scholar
Huh, C. & Scriven, L. 1971 Hydrodynamic model of steady movement of a solid/liquid/fluid contact line. J. Colloid Interface Sci. 35, 85–101.Google Scholar
Ishino, C., Reyssat, M., Reyssat, E., Okumura, K. & Quéré, D. 2007 Wicking within forests of micropillars. Europhys. Lett. 79, 56005.Google Scholar
Karniadakis, G., Beşkök, A. & Aluru, N. R. 2005 Microflows and Nanoflows: Fundamentals and Simulation. Springer.Google Scholar
Kim, S. J., Moon, M. W., Lee, K. R., Lee, D. Y., Chang, Y. S. & Kim, H. Y. 2011 Liquid spreading on superhydrophilic micropillar arrays. J. Fluid Mech. 680, 477–487.Google Scholar
McHale, G., Shirtcliffe, N., Aqil, S., Perry, C. & Newton, M. 2004 Topography driven spreading. Phys. Rev. Lett. 93, 36102.CrossRefGoogle ScholarPubMed
Nagrath, S., Sequist, L. V., Maheswaran, S., Bell, D. W., Irimia, D., Ulkus, L., Smith, M. R., Kwak, E. L., Digumarthy, S. & Muzikansky, A. 2007 Isolation of rare circulating tumour cells in cancer patients by microchip technology. Nature 450, 1235–1239.CrossRefGoogle ScholarPubMed
Parker, A. R. & Lawrence, C. R. 2001 Water capture by a desert beetle. Nature 414, 33–34.Google Scholar
Plimpton, S. 1995 Fast parallel algorithms for short-range molecular-dynamics. J. Comput. Phys. 117, 1–19.Google Scholar
Quéré, D. 2008 Wetting and roughness. Annu. Rev. Mater. Res. 38, 71–99.Google Scholar
Ren, W. Q. & E, W. N. 2007 Boundary conditions for the moving contact line problem. Phys. Fluids 19, 022101.Google Scholar
Ren, W. Q., Hu, D. & E, W. N. 2010 Continuum models for the contact line problem. Phys. Fluids 22, 102103.Google Scholar
Seemann, R., Brinkmann, M., Kramer, E., Lange, F. & Lipowsky, R. 2005 Wetting morphologies at microstructured surfaces. Proc. Natl Acad. Sci. USA 102, 1848–1852.Google Scholar
Skelley, A. M., Kirak, O., Suh, H., Jaenisch, R. & Voldman, J. 2009 Microfluidic control of cell pairing and fusion. Nat. Meth. 6, 147–152.Google Scholar
Srivastava, N., Din, C., Judson, A., MacDonald, N. C. & Meinhart, C. D. 2010 A unified scaling model for flow through a lattice of microfabricated posts. Lab on a Chip 10, 1148–1152.CrossRefGoogle ScholarPubMed
Tanner, L. 1979 The spreading of silicone oil drops on horizontal surfaces. J. Phys. D: Appl. Phys. 12, 1473–1484.Google Scholar
Teletzke, G. F., Davis, H. T. E. D. & Scriven, L. 1987 How liquids spread on solids. Chem. Engng Commun. 55, 41–82.Google Scholar
Vega, C. & De Miguel, E. 2007 Surface tension of the most popular models of water by using the test-area simulation method. J. Chem. Phys. 126, 154707.Google Scholar
Wang, C. L., Lu, H. J., Wang, Z. G., Xiu, P., Zhou, B., Zuo, G. H., Wan, R. Z., Hu, J. & Fang, H. P. 2009 Stable liquid water droplet on a water monolayer formed at room temperature on ionic model substrates. Phys. Rev. Lett. 103, 137801.Google Scholar
Weislogel, M. M. & Lichter, S. 1998 Capillary flow in an interior corner. J. Fluid Mech. 373, 349–378.Google Scholar
Wenzel, R. N. 1936 Resistance of solid surfaces to wetting by water. Ind. Engng Chem. 28, 988–994.Google Scholar
Xiao, R., Enright, R. & Wang, E. N. 2010 Prediction and optimization of liquid propagation in micropillar arrays. Langmuir 26, 15070–15075.Google Scholar
Young, T. 1805 An essay on the cohesion of fluids. Phil. Trans. R. Soc. Lond. 95, 65–87.Google Scholar
Yuan, Q. Z. & Zhao, Y. P. 2010 Precursor film in dynamic wetting, electrowetting, and electro-elasto-capillarity. Phys. Rev. Lett. 104, 246101.Google Scholar
Yuan, Q. Z. & Zhao, Y. P. 2012 Topology-dominated dynamic wetting of the precursor chain in a hydrophilic interior corner. Proc. R. Soc. A 468, 310–322.Google Scholar