Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-13T06:42:09.317Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear dynamics of two-layer channel flow with soluble surfactant below or above the critical micelle concentration

Published online by Cambridge University Press:  04 August 2020

A. Kalogirou Open the ORCID record for A. Kalogirou [Opens in a new window]  and
M. G. Blyth Open the ORCID record for M. G. Blyth [Opens in a new window]
A. Kalogirou*
Affiliation:
School of Mathematical Sciences, University of Nottingham, University Park, NottinghamNG7 2RD, UK
M. G. Blyth
Affiliation:
School of Mathematics, University of East Anglia, Norwich Research Park, NorwichNR4 7TJ, UK
*
†Email address for correspondence: anna.kalogirou@nottingham.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

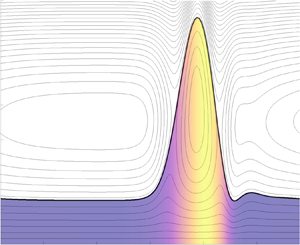
The nonlinear stability of an inertialess two-layer surfactant-laden Couette flow is considered. The two fluids are immiscible and have different thicknesses, viscosities and densities. One of the fluids is contaminated with a soluble surfactant whose concentration may be above the critical micelle concentration, in which case micelles are formed in the bulk of the fluid. A surfactant kinetic model is adopted that includes the adsorption and desorption of molecules to and from the interface, and the formation and breakup of micelles in the bulk. The lubrication approximation is applied and a strongly nonlinear system of equations is derived for the evolution of the interface and surfactant concentration at the interface, as well as the vertically averaged monomer and micelle concentrations in the bulk (as a result of fast vertical diffusion). The primary aim of this study is to determine the influence of surfactant solubility on the nonlinear dynamics. The nonlinear lubrication model is solved numerically in periodic domains and saturated travelling waves are obtained at large times. It is found that a sufficiently soluble surfactant can either destabilise or stabilise the interface depending on certain fluid properties. The stability behaviour of the system depends crucially on the values of the fluid viscosity ratio $m$ and thickness ratio $n$ in reference to the boundary $m=n^{2}$. If the surfactant exists at large concentrations that exceed the critical micelle concentration, then long waves are stable at large times, unless density stratification effects overcome the stabilising influence of micelles. Travelling wave bifurcation branches are also calculated and the impact of various parameters (such as the domain length or fluid thickness ratio) on the wave shapes, amplitudes and speeds is examined. The mechanism responsible for interfacial (in)stability is explained in terms of the phase difference between the interface deformation and concentration waves, which is shifted according to the sign of the crucial factor $(m-n^{2})$ and the strength of the surfactant solubility.

JFM classification

Waves/Free-surface Flows: Channel flow Interfacial Flows (free surface): Thin films
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , A7
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Afsar-Siddiqui, A. B., Luckham, P. F. & Matar, O. 2003 The spreading of surfactant solutions on thin liquid films. Adv. Colloid Interface Sci. 106, 183–236.CrossRefGoogle ScholarPubMed
Babchin, A. J., Frenkel, A. L., Levich, B. G. & Sivashinsky, G. I. 1983 Nonlinear saturation of Rayleigh–Taylor instability in thin films. Phys. Fluids 26, 3159–3161.CrossRefGoogle Scholar
Barthelet, P., Charru, F. & Fabre, J. 1995 Experimental study of interfacial long waves in a two-layer shear flow. J. Fluid Mech. 303, 23–53.CrossRefGoogle Scholar
Bassom, A. P., Blyth, M. G. & Papageorgiou, D. T. 2010 Nonlinear development of two-layer Couette–Poiseuille flow in the presence of surfactant. Phys. Fluids 22 (10), 102102.CrossRefGoogle Scholar
Berret, J.-C. 2006 Rheology of wormlike micelles: equilibrium properties and shear banding transitions. In Molecular Gels: Materials with Self-Assembled Fibrillar Networks (ed. Weiss, R. G. & Terech, P.), chap. 19, pp. 667–720. Springer.CrossRefGoogle Scholar
Blyth, M. G. & Pozrikidis, C. 2004 Effect of surfactants on the stability of two-layer channel flow. J.Fluid Mech. 505, 59–86.CrossRefGoogle Scholar
Blyth, M. G., Tseluiko, D., Lin, T.-S. & Kalliadasis, S. 2018 Two-dimensional pulse dynamics and the formation of bound states on electrified falling films. J. Fluid Mech. 855, 210–235.CrossRefGoogle Scholar
Breward, C. J. W. & Howell, P. D. 2004 Straining flow of a micellar surfactant solution. Eur. J. Appl. Maths 15, 511–531.CrossRefGoogle Scholar
Chang, C.-H. & Franses, E. I. 1995 Adsorption dynamics of surfactants at the air/water interface: a critical review of mathematical models, data, and mechanisms. Colloid Surf. A 100, 1–45.CrossRefGoogle Scholar
Chen, L.-H. & Lee, Y.-L. 2000 Adsorption behavior of surfactants and mass transfer in single-drop extraction. AIChE J. 46, 160–168.CrossRefGoogle Scholar
Craster, R. V., Matar, O. & Papageorgiou, D. T. 2009 Breakup of surfactant-laden jets above the critical micelle concentration. J. Fluid Mech. 629, 195–219.CrossRefGoogle Scholar
Danov, K. D., Vlahovska, P. M., Horozov, T., Dushskin, C. D., Kralchevsky, P. A., Mehreteab, A. & Broze, G. 1996 Adsorption from micellar surfactant solutions: nonlinear theory and experiment. J. Colloid Interface Sci. 183, 223–235.CrossRefGoogle Scholar
Doedel, E. J. & Oldman, B. E. 2009 AUTO-07P: Continuation and Bifurcation Software for Ordinary Differential Equations. Concordia University.Google Scholar
Edmonstone, B. D., Craster, R. V. & Matar, O. K. 2006 Surfactant-induced fingering phenomena beyond the critical micelle concentration. J. Fluid Mech. 564, 105–138.CrossRefGoogle Scholar
Frenkel, A. L. & Halpern, D. 2002 Stokes-flow instability due to interfacial surfactant. Phys. Fluids 14 (7), 1–4.CrossRefGoogle Scholar
Frenkel, A. L. & Halpern, D. 2017 Surfactant and gravity dependent instability of two-layer Couette flows and its nonlinear saturation. J. Fluid Mech. 826, 158–204.CrossRefGoogle Scholar
Frenkel, A. L., Halpern, D. & Schweiger, A. J. 2019 a Surfactant- and gravity-dependent instability of two-layer channel flows: linear theory covering all wavelengths. Part 1. ‘Long-wave’ regimes. J.Fluid Mech. 863, 150–184.CrossRefGoogle Scholar
Frenkel, A. L., Halpern, D. & Schweiger, A. J. 2019 b Surfactant- and gravity-dependent instability of two-layer channel flows: linear theory covering all wavelengths. Part 2. Mid-wave regimes. J.Fluid Mech. 863, 185–214.CrossRefGoogle Scholar
Gallagher, C. T., Leighton, D. T. & McCready, M. J. 1996 Experimental investigation of a two-layer shearing instability in a cylindrical Couette cell. Phys. Fluids 8 (9), 2385–2392.CrossRefGoogle Scholar
Georgantaki, A., Vlachogiannis, M. & Bontozoglou, V. 2016 Measurements of the stabilisation of liquid film flow by the soluble surfactant sodium dodecyl sulfate (SDS). Intl J. Multiphase Flow 86, 28–34.CrossRefGoogle Scholar
Gupta, L. & Wasan, D. T. 1974 Surface shear viscosity and related properties of adsorbed surfactant films. Ind. Engng Chem. Fundam. 13 (1), 26–33.CrossRefGoogle Scholar
Halpern, D. & Frenkel, A. L. 2003 Destabilization of a creeping flow by interfacial surfactant: linear theory extended to all wavenumbers. J. Fluid Mech. 485, 191–220.CrossRefGoogle Scholar
Hooper, A. P. 1985 Long-wave instability at the interface between two viscous fluids: thin layer effects. Phys. Fluids 28 (6), 1613–1618.CrossRefGoogle Scholar
Hooper, A. P. & Grimshaw, R. 1985 Nonlinear instability at the interface between two viscous fluids. Phys. Fluids 28 (1), 37–45.CrossRefGoogle Scholar
Jensen, O. E. & Grotberg, J. B. 1993 The spreading of heat or soluble surfactant along a thin liquid film. Phys. Fluids 5, 58–68.CrossRefGoogle Scholar
Kalogirou, A. 2018 Instability of two-layer film flows due to the interacting effects of surfactants, inertia and gravity. Phys. Fluids 30 (3), 030707.CrossRefGoogle Scholar
Kalogirou, A. & Blyth, M. G. 2019 The role of soluble surfactants in the linear stability of two-layer flow in a channel. J. Fluid Mech. 873, 18–48.CrossRefGoogle Scholar
Kalogirou, A. & Papageorgiou, D. T. 2016 Nonlinear dynamics of surfactant-laden two-fluid Couette flows in the presence of inertia. J. Fluid Mech. 802, 5–36.CrossRefGoogle Scholar
Kalogirou, A., Papageorgiou, D. T. & Smyrlis, Y.-S. 2012 Surfactant destabilisation and nonlinear phenomena in two-fluid shear flows at small Reynolds numbers. IMA J. Appl. Maths 77, 351–360.CrossRefGoogle Scholar
Langevin, D. 2014 Rheology of adsorbed surfactant monolayers at fluid surfaces. Annu. Rev. Fluid Mech. 46, 47–65.CrossRefGoogle Scholar
Maisch, P., Eisenhofer, L. M., Tam, K. C., Distler, A., Voigt, M. M., Brabec, C. J. & Egelhaaf, H.-J. 2019 A generic surfactant-free approach to overcome wetting limitations and its application to improve inkjet-printed P3HT: non-fullerene acceptor PV. J. Mater. Chem. A 7, 13215–13224.CrossRefGoogle Scholar
Mavromoustaki, A., Matar, O. & Craster, R. V. 2012 a Dynamics of a climbing surfactant-laden film – I: base-state flow. J. Colloid Interface Sci. 371, 107–120.CrossRefGoogle ScholarPubMed
Mavromoustaki, A., Matar, O. & Craster, R. V. 2012 b Dynamics of a climbing surfactant-laden film – II: stability. J. Colloid Interface Sci. 371, 121–135.CrossRefGoogle ScholarPubMed
Morgan, C. E., Breward, C. J. W., Griffiths, I. M., Howell, P. D., Penfold, J., Thomas, R.K., Tucker, I., Petkov, J. T. & Webster, J. R. P. 2012 Kinetics of surfactant desorption at an air-solution interface. Langmuir 28, 17339–17348.CrossRefGoogle ScholarPubMed
Ooms, G., Segal, A. & Cheung, S. Y. 1985 Propagation of long waves of finite amplitude at the interface of two viscous fluids. Intl J. Multiphase Flow 11, 481–502.CrossRefGoogle Scholar
Orzag, S. A. 1971 Accurate solution of the Orr–Sommerfeld stability equation. J. Fluid Mech. 50, 659–703.Google Scholar
Pereira, A. & Kalliadasis, S. 2008 Dynamics of a falling film with solutal Marangoni effect. Phys. Rev. E 78 (3), 036312.CrossRefGoogle ScholarPubMed
Petkova, R., Tcholakova, S. & Denkov, N. D. 2012 Foaming and foam stability for mixed polymer–surfactant solutions: effects of surfactant type and polymer charge. Langmuir 28, 4996–5009.CrossRefGoogle ScholarPubMed
Phan, C. M., Nguyen, A. V. & Evans, G. M. 2005 Dynamic adsorption of sodium dodecylbenzene sulphonate and dowfroth 250 onto the air-water interface. Miner. Engng 18, 599–603.CrossRefGoogle Scholar
Picardo, J. R., Radhakrishna, T. G. & Pushpavanam, S. 2016 Solutal Marangoni instability in layered two-phase flows. J. Fluid Mech. 793, 280–315.CrossRefGoogle Scholar
Ponce-Torres, A., Montanero, J. M., Herrada, M. A., Vega, E. J. & Vega, J. M. 2017 Influence of the surface viscosity on the breakup of a surfactant-laden drop. Phys. Rev. Lett. 118 (2), 024501.CrossRefGoogle ScholarPubMed
Power, H., Villegas, M. & Carmona, C. 1991 Nonlinear inertial effects on the instability of a single long wave of finite amplitude at the interface of two viscous fluids. Z. Angew. Math. Phys. 42, 663–679.CrossRefGoogle Scholar
Pozrikidis, C. 2004 Instability of multi-layer channel and film flows. Adv. Appl. Mech. 40, 179–239.CrossRefGoogle Scholar
Renardy, Y. Y. 1985 Instability at the interface between two shearing fluids in a channel. Phys. Fluids 28 (12), 3441–3443.CrossRefGoogle Scholar
Renardy, Y. 1989 Weakly nonlinear behavior of periodic disturbances in two-layer Couette–Poiseuille flow. Phys. Fluids A 1 (10), 1666–1676.CrossRefGoogle Scholar
Samanta, A. 2013 Effect of surfactant on two-layer channel flow. J. Fluid Mech. 735, 519–552.CrossRefGoogle Scholar
Shen, A. Q., Gleason, B., McKinley, G. H. & Stone, H. A. 2002 Fiber coating with surfactant solutions. Phys. Fluids 14, 4055–4068.CrossRefGoogle Scholar
Song, Q., Couzis, A., Somasundaran, P. & Maldarelli, C. 2006 A transport model for the adsorption of surfactant from micelle solutions onto a clean air/water interface in the limit of rapid aggregate disassembly relative to diffusion and supporting dynamic tension experiments. Colloids Surf. A 282–283, 162–182.Google Scholar
Thompson, J. & Blyth, M. G. 2016 Inertialess multilayer film flow with surfactant: stability and traveling waves. Phys. Rev. Fluids 1 (6), 063904.CrossRefGoogle Scholar
Tilley, B. S., Davis, S. H. & Bankoff, S. G. 1994 a Linear stability theory of two-layer fluid flow in an inclined channel. Phys. Fluids 6 (12), 3906–3922.CrossRefGoogle Scholar
Tilley, B. S., Davis, S. H. & Bankoff, S. G. 1994 b Nonlinear long-wave stability of superposed fluids in an inclined channel. J. Fluid Mech. 277, 55–83.CrossRefGoogle Scholar
Trefethen, L. N. 2000 Spectral Methods in Matlab. Society for Industrial and Applied Mathematics.CrossRefGoogle Scholar
Wei, H.-H. 2005 On the flow-induced Marangoni instability due to the presence of surfactant. J. Fluid Mech. 544, 173–200.CrossRefGoogle Scholar
Weinstein, S. J. & Ruschak, K. J. 2004 Coating flows. Annu. Rev. Fluid Mech. 36, 29–53.CrossRefGoogle Scholar
Yih, C.-H. 1967 Instability due to viscosity stratification. J. Fluid Mech. 27, 337–352.CrossRefGoogle Scholar
Zell, Z. A., Nowbahar, A., Mansard, V., Leal, L. G., Deshmukh, S. S., Mecca, J. M., Tucker, C. J. & Squires, T. M. 2014 Surface shear inviscidity of soluble surfactants. Proc. Natl Acad. Sci. USA 111 (10), 3677–3682.CrossRefGoogle ScholarPubMed

Kalogirou and Blyth supplementary movie 1

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:4, m = 0:5, 􀀀 = 0:5 and Kb ≫ 1. This movie, together with Movies 2 and 3, demonstrate stabilisation of the interface for a sufficiently strong surfactant solubility.

Download Kalogirou and Blyth supplementary movie 1(Video)
Video 92.1 KB

Kalogirou and Blyth supplementary movie 2

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:4, m = 0:5, 􀀀 = 0:5 and Kb = 5. This movie, together with Movies 1 and 3, demonstrate stabilisation of the interface for a sufficiently strong surfactant solubility.

Download Kalogirou and Blyth supplementary movie 2(Video)
Video 85 KB

Kalogirou and Blyth supplementary movie 3

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:4, m = 0:5, 􀀀 = 0:5 and Kb = 2. This movie, together with Movies 1 and 2, demonstrate stabilisation of the interface for a sufficiently strong surfactant solubility.

Download Kalogirou and Blyth supplementary movie 3(Video)
Video 59.4 KB

Kalogirou and Blyth supplementary movie 4

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:4, m = 5, 􀀀 = 0:5 and Kb ≫ 1. This movie, together with Movie 5, demonstrate destabilisation of the interface for a sufficiently strong surfactant solubility.

Download Kalogirou and Blyth supplementary movie 4(Video)
Video 133 KB

Kalogirou and Blyth supplementary movie 5

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:4, m = 5, 􀀀 = 0:5 and Kb = 2. This movie, together with Movie 4, demonstrate destabilisation of the interface for a sufficiently strong surfactant solubility.

Download Kalogirou and Blyth supplementary movie 5(Video)
Video 221.2 KB

Kalogirou and Blyth supplementary movie 6

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:2, m = 0:5, 􀀀 = 0:75 and Kb ≫ 1. This movie, together with Movie 7, demonstrate stabilisation of the interface for bulk concentrations above the critical micelle concentration.

Download Kalogirou and Blyth supplementary movie 6(Video)
Video 78 KB

Kalogirou and Blyth supplementary movie 7

Time evolution of the interfacial wave (top), interfacial surfactant concentration (middle), and solution norm (bottom) for h0 = 0:2, m = 0:5, 􀀀 = 0:75 and Kb = 3. This movie, together with Movie 6, demonstrate stabilisation of the interface for bulk concentrations above the critical micelle concentration.

Download Kalogirou and Blyth supplementary movie 7(Video)
Video 54 KB