Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-10T06:37:35.424Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonlinear input/output analysis: application to boundary layer transition

Published online by Cambridge University Press:  26 January 2021

Georgios Rigas Open the ORCID record for Georgios Rigas [Opens in a new window] ,
Denis Sipp Open the ORCID record for Denis Sipp [Opens in a new window]  and
Tim Colonius
Georgios Rigas*
Affiliation:
Division of Engineering and Applied Science, California Institute of Technology, Pasadena, CA91125, USA
Denis Sipp
Affiliation:
DAAA, ONERA, Université Paris Saclay, 8 rue des Vertugadins, 92190Meudon, France
Tim Colonius
Affiliation:
Division of Engineering and Applied Science, California Institute of Technology, Pasadena, CA91125, USA
*
Email address for correspondence: g.rigas@imperial.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

We extend linear input/output (resolvent) analysis to take into account nonlinear triadic interactions by considering a finite number of harmonics in the frequency domain using the harmonic balance method. Forcing mechanisms that maximise the drag are calculated using a gradient-based ascent algorithm. By including nonlinearity in the analysis, the proposed frequency-domain framework identifies the worst-case disturbances for laminar-turbulent transition. We demonstrate the framework on a flat-plate boundary layer by considering three-dimensional spanwise-periodic perturbations triggered by a few optimal forcing modes of finite amplitude. Two types of volumetric forcing are considered, one corresponding to a single frequency/spanwise wavenumber pair, and a multi-harmonic where a harmonic frequency and wavenumber are also added. Depending on the forcing strategy, we recover a range of transition scenarios associated with $K$-type and $H$-type mechanisms, including oblique and planar Tollmien–Schlichting waves, streaks and their breakdown. We show that nonlinearity plays a critical role in optimising growth by combining and redistributing energy between the linear mechanisms and the higher perturbation harmonics. With a very limited range of frequencies and wavenumbers, the calculations appear to reach the early stages of the turbulent regime through the generation and breakdown of hairpin and quasi-streamwise staggered vortices.

JFM classification

Instability: Transition to turbulence Boundary Layers: Boundary layer stability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 911 , 25 March 2021 , A15
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

†

Present address: Department of Aeronautics, Imperial College London, London SW7 2AZ, UK.

References

REFERENCES

Åkervik, E., Ehrenstein, U., Gallaire, F. & Henningson, D.S. 2008 Global two-dimensional stability measures of the flat plate boundary-layer flow. Eur. J. Mech. B/Fluids 27 (5), 501–513.CrossRefGoogle Scholar
Amestoy, P.R., Duff, I.S., Koster, J. & L'Excellent, J.-Y. 2001 A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Applics. 23 (1), 15–41.CrossRefGoogle Scholar
Andersson, P., Brandt, L., Bottaro, A. & Henningson, D.S. 2001 On the breakdown of boundary layer streaks. J. Fluid Mech. 428, 29–60.CrossRefGoogle Scholar
Arnold, D.N., Brezzi, F. & Fortin, M. 1984 A stable finite element for the Stokes equations. Calcolo 21 (4), 337–344.CrossRefGoogle Scholar
Asai, M., Minagawa, M. & Nishioka, M. 2002 The instability and breakdown of a near-wall low-speed streak. J. Fluid Mech. 455, 289–314.CrossRefGoogle Scholar
Bake, S., Meyer, D.G.W. & Rist, U. 2002 Turbulence mechanism in Klebanoff transition: a quantitative comparison of experiment and direct numerical simulation. J. Fluid Mech. 459, 217–243.CrossRefGoogle Scholar
Berlin, S., Lundbladh, A. & Henningson, D.S. 1994 Spatial simulations of oblique transition in a boundary layer. Phys. Fluids 6 (6), 1949–1951.CrossRefGoogle Scholar
Berlin, S., Wiegel, M. & Henningson, D.S. 1999 Numerical and experimental investigations of oblique boundary layer transition. J. Fluid Mech. 393, 23–57.CrossRefGoogle Scholar
Bertolotti, F.P., Herbert, T. & Spalart, P.R. 1992 Linear and nonlinear stability of the Blasius boundary layer. J. Fluid Mech. 242, 441–474.CrossRefGoogle Scholar
Biau, D. & Bottaro, A. 2008 An optimal path to transition in a duct. Phil. Trans. R. Soc. Lond. A 367 (1888), 529–544.Google Scholar
Biau, D. & Bottaro, A. 2009 An optimal path to transition in a duct. Phil. Trans. R. Soc. Lond. A 367 (1888), 529–544.Google Scholar
Blackburn, H.M., Barkley, D. & Sherwin, S.J. 2008 Convective instability and transient growth in flow over a backward-facing step. J. Fluid Mech. 603, 271–304.CrossRefGoogle Scholar
Brandt, L. 2007 Numerical studies of the instability and breakdown of a boundary-layer low-speed streak. Eur. J. Mech. B/Fluids 26 (1), 64–82.CrossRefGoogle Scholar
Brandt, L. & Henningson, D.S. 2002 Transition of streamwise streaks in zero-pressure-gradient boundary layers. J. Fluid Mech. 472, 229–261.CrossRefGoogle Scholar
Brandt, L., Sipp, D., Pralits, J.O. & Marquet, O. 2011 Effect of base-flow variation in noise amplifiers: the flat-plate boundary layer. J. Fluid Mech. 687, 503–528.CrossRefGoogle Scholar
Breuer, K.S., Cohen, J. & Haritonidis, J.H. 1997 The late stages of transition induced by a low-amplitude wavepacket in a laminar boundary layer. J. Fluid Mech. 340, 395–411.CrossRefGoogle Scholar
Butler, K.M. & Farrell, B.F. 1992 Three–dimensional optimal perturbations in viscous shear flow. Phys. Fluids A 4 (8), 1637–1650.CrossRefGoogle Scholar
Chang, C.-L. & Malik, M.R. 1994 Oblique-mode breakdown and secondary instability in supersonic boundary layers. J. Fluid Mech. 273, 323–360.CrossRefGoogle Scholar
Cherubini, S., De Palma, P., Robinet, J.-C. & Bottaro, A. 2010 Rapid path to transition via nonlinear localized optimal perturbations in a boundary-layer flow. Phys. Rev. E 82 (6), 066302.CrossRefGoogle Scholar
Cherubini, S., De Palma, P., Robinet, J.-C. & Bottaro, A. 2011 The minimal seed of turbulent transition in the boundary layer. J. Fluid Mech. 689, 221–253.CrossRefGoogle Scholar
Duguet, Y., Brandt, L. & Larsson, B.R.J. 2010 Towards minimal perturbations in transitional plane Couette flow. Phys. Rev. E 82 (2), 026316.CrossRefGoogle ScholarPubMed
Duguet, Y., Monokrousos, A., Brandt, L. & Henningson, D.S 2013 Minimal transition thresholds in plane Couette flow. Phys. Fluids 25 (8), 084103.CrossRefGoogle Scholar
Duguet, Y., Schlatter, P., Henningson, D.S. & Eckhardt, B. 2012 Self-sustained localized structures in a boundary-layer flow. Phys. Rev. Lett. 108 (4), 044501.CrossRefGoogle Scholar
Fabre, D., Citro, V., Ferreira-Sabino, D., Bonnefis, P., Sierra, J., Giannetti, F. & Pigou, M. 2018 A practical review on linear and nonlinear global approaches to flow instabilities. Appl. Mech. Rev. 70 (6).CrossRefGoogle Scholar
Farrell, B.F., Gayme, D.F. & Ioannou, P.J. 2017 A statistical state dynamics approach to wall turbulence. Phil. Trans. R. Soc. Lond. A 375 (2089), 20160081.Google ScholarPubMed
Farrell, B.F. & Ioannou, P.J. 2012 Dynamics of streamwise rolls and streaks in turbulent wall-bounded shear flow. J. Fluid Mech. 708, 149–196.CrossRefGoogle Scholar
Gopinath, A., Van Der Weide, E., Alonso, J., Jameson, A., Ekici, K. & Hall, K. 2007 Three-dimensional unsteady multi-stage turbomachinery simulations using the harmonic balance technique. In 45th AIAA Aerospace Sciences Meeting and Exhibit, p. 892.Google Scholar
Hack, M.J.P. & Moin, P. 2018 Coherent instability in wall-bounded shear. J. Fluid Mech. 844, 917–955.CrossRefGoogle Scholar
Hack, M.J.P. & Zaki, T.A. 2014 Streak instabilities in boundary layers beneath free-stream turbulence. J. Fluid Mech. 741, 280–315.CrossRefGoogle Scholar
Hall, K.C., Thomas, J.P. & Clark, W.S. 2002 Computation of unsteady nonlinear flows in cascades using a harmonic balance technique. AIAA J. 40 (5), 879–886.CrossRefGoogle Scholar
Hecht, F. 2012 New development in FreeFem$++$. J. Numer. Maths 20 (3–4), 251–265.Google Scholar
Herbert, T. 1988 Secondary instability of boundary layers. Annu. Rev. Fluid Mech. 20 (1), 487–526.CrossRefGoogle Scholar
Huang, Z. & Hack, M.J.P. 2020 A variational framework for computing nonlinear optimal disturbances in compressible flows. J. Fluid Mech. 894, A5.CrossRefGoogle Scholar
Jahanbakhshi, R. & Zaki, T.A. 2019 Nonlinearly most dangerous disturbance for high-speed boundary-layer transition. J. Fluid Mech. 876, 87–121.CrossRefGoogle Scholar
Jovanović, M.R. & Bamieh, B. 2005 Componentwise energy amplification in channel flows. J. Fluid Mech. 534, 145–183.CrossRefGoogle Scholar
Juniper, M.P. 2011 Triggering in the horizontal Rijke tube: non-normality, transient growth and bypass transition. J. Fluid Mech. 667, 272.CrossRefGoogle Scholar
Kachanov, Y.S., Kozlov, V.V. & Levchenko, V.Y. 1977 Nonlinear development of a wave in a boundary layer. Fluid Dyn. 12 (3), 383–390.CrossRefGoogle Scholar
Kachanov, Y.S. & Levchenko, V.Y. 1984 The resonant interaction of disturbances at laminar-turbulent transition in a boundary layer. J. Fluid Mech. 138, 209–247.CrossRefGoogle Scholar
Karp, M. & Cohen, J. 2017 On the secondary instabilities of transient growth in Couette flow. J. Fluid Mech. 813, 528–557.CrossRefGoogle Scholar
Kerswell, R.R. 2018 Nonlinear nonmodal stability theory. Ann. Rev. Fluid Mech. 50, 319–345.CrossRefGoogle Scholar
Khalil, H.K. & Grizzle, J.W. 2002 Nonlinear Systems, vol. 3. Prentice Hall.Google Scholar
Klebanoff, P.S., Tidstrom, K.D. & Sargent, L.M. 1962 The three-dimensional nature of boundary-layer instability. J. Fluid Mech. 12 (1), 1–34.CrossRefGoogle Scholar
Landahl, M.T. 1980 A note on an algebraic instability of inviscid parallel shear flows. J. Fluid Mech. 98 (2), 243–251.CrossRefGoogle Scholar
Lazarus, A. & Thomas, O. 2010 A harmonic-based method for computing the stability of periodic solutions of dynamical systems. C. R. Méc 338 (9), 510–517.CrossRefGoogle Scholar
Mantič-Lugo, V. & Gallaire, F. 2016 Self-consistent model for the saturation mechanism of the response to harmonic forcing in the backward-facing step flow. J. Fluid Mech. 793, 777–797.CrossRefGoogle Scholar
Mantič-Lugo, V., Arratia, C. & Gallaire, F. 2014 Self-consistent mean flow description of the nonlinear saturation of the vortex shedding in the cylinder wake. Phys. Rev. Lett. 113, 084501.CrossRefGoogle ScholarPubMed
Monokrousos, A., Åkervik, E., Brandt, L. & Henningson, D.S. 2010 Global three-dimensional optimal disturbances in the blasius boundary-layer flow using time-steppers. J. Fluid Mech. 650, 181–214.CrossRefGoogle Scholar
Monokrousos, A., Bottaro, A., Brandt, L., Di Vita, A. & Henningson, D.S. 2011 Nonequilibrium thermodynamics and the optimal path to turbulence in shear flows. Phys. Rev. Lett. 106 (13), 134502.CrossRefGoogle ScholarPubMed
Moulin, J., Jolivet, P. & Marquet, O. 2019 Augmented Lagrangian preconditioner for large-scale hydrodynamic stability analysis. Comput. Meth. Appl. Mech. Engng 351, 718–743.CrossRefGoogle Scholar
Peixinho, J. & Mullin, T. 2007 Finite-amplitude thresholds for transition in pipe flow. J. Fluid Mech. 582, 169.CrossRefGoogle Scholar
Pickering, E., Rigas, G., Nogueira, P.A.S., Cavalieri, A.V.G., Schmidt, O.T. & Colonius, T. 2020 Lift-up, Kelvin–Helmholtz and Orr mechanisms in turbulent jets. J. Fluid Mech. 896, A2.CrossRefGoogle Scholar
Pringle, C.C.T., Willis, A.P & Kerswell, R.R. 2012 Minimal seeds for shear flow turbulence: using nonlinear transient growth to touch the edge of chaos. J. Fluid Mech. 702, 415–443.CrossRefGoogle Scholar
Reddy, S.C., Schmid, P.J., Baggett, J.S. & Henningson, D.S. 1998 On stability of streamwise streaks and transition thresholds in plane channel flows. J. Fluid Mech. 365, 269–303.CrossRefGoogle Scholar
Rigas, G., Schmidt, O.T., Colonius, T. & Bres, G.A. 2017 One way Navier-Stokes and resolvent analysis for modeling coherent structures in a supersonic turbulent jet. In 23rd AIAA/CEAS Aeroacoustics Conference, p. 4046.Google Scholar
Rist, U. & Fasel, H. 1995 Direct numerical simulation of controlled transition in a flat-plate boundary layer. J. Fluid Mech. 298, 211–248.CrossRefGoogle Scholar
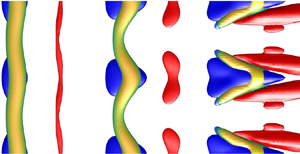
Sayadi, T., Hamman, C.W. & Moin, P. 2013 Direct numerical simulation of complete $H$-type and $K$-type transitions with implications for the dynamics of turbulent boundary layers. J. Fluid Mech. 724, 480–509.CrossRefGoogle Scholar
Schmid, P.J. 2007 Nonmodal stability theory. Annu. Rev. Fluid Mech. 39, 129–162.CrossRefGoogle Scholar
Schmid, P.J. & Henningson, D.S. 1992 A new mechanism for rapid transition involving a pair of oblique waves. Phys. Fluids A 4 (9), 1986–1989.CrossRefGoogle Scholar
Schmid, P.J. & Henningson, D.S. 2001 Stability and Transition in Shear Flows. Springer.CrossRefGoogle Scholar
Schmidt, O.T., Towne, A., Rigas, G., Colonius, T. & Brès, G.A. 2018 Spectral analysis of jet turbulence. J. Fluid Mech. 855, 953–982.CrossRefGoogle Scholar
Sicot, F., Dufour, G. & Gourdain, N. 2012 A time-domain harmonic balance method for rotor/stator interactions. Trans. ASME: J. Turbomach. 134 (1), 011001.Google Scholar
Sipp, D. & Marquet, O. 2013 Characterization of noise amplifiers with global singular modes: the case of the leading-edge flat-plate boundary layer. Theor. Comput. Fluid Dyn. 27 (5), 617–635.CrossRefGoogle Scholar
Sipp, D., Marquet, O., Meliga, P. & Barbagallo, A. 2010 Dynamics and control of global instabilities in open-flows: a linearized approach. Appl. Mech. Rev. 63 (3), 030801.CrossRefGoogle Scholar
Theofilis, V. 2011 Global linear instability. Annu. Rev. Fluid Mech. 43, 319–352.CrossRefGoogle Scholar
Thomas, V.L., Farrell, B.F., Ioannou, P.J. & Gayme, D.F. 2015 A minimal model of self-sustaining turbulence. Phys. Fluids 27 (10), 105104.CrossRefGoogle Scholar
Towne, A., Rigas, G. & Colonius, T. 2019 A critical assessment of the parabolized stability equations. In Theoretical and Computational Fluid Dynamics, pp. 1–24.Google Scholar
Trefethen, L.N., Trefethen, A.E., Reddy, S.C. & Driscoll, T.A. 1993 Hydrodynamic stability without eigenvalues. Science 261 (5121), 578–584.CrossRefGoogle ScholarPubMed
Vavaliaris, C., Beneitez, M. & Henningson, D.S. 2020 Optimal perturbations and transition energy thresholds in boundary layer shear flows. Phys. Rev. Fluids 5 (6), 062401.CrossRefGoogle Scholar
Waleffe, F. 1997 On a self-sustaining process in shear flows. Phys. Fluids 9 (4), 883–900.CrossRefGoogle Scholar
Waleffe, F. & Kim, J. 1997 How streamwise rolls and streaks self-sustain in a shear flow. In Self-Sustaining Mechanisms of Wall Turbulence (ed. R.L. Panton), Advances in Fluid Mechanics, vol. 15, pp. 309–332.Google Scholar
White, F.M. 1991 Viscous Fluid Flow. McGraw-Hill.Google Scholar
Yeo, K.S., Zhao, X., Wang, Z.Y. & Ng, K.C. 2010 DNS of wavepacket evolution in a Blasius boundary layer. J. Fluid Mech. 652, 333–372.CrossRefGoogle Scholar