Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-14T06:24:47.327Z Has data issue: false hasContentIssue false
  • English
  • Français

Numerical investigation of starting turbulent buoyant plumes released in neutral atmosphere

Published online by Cambridge University Press:  13 August 2020

Kiran Bhaganagar Open the ORCID record for Kiran Bhaganagar [Opens in a new window]  and
Sudheer R. Bhimireddy
Kiran Bhaganagar*
Affiliation:
Department of Mechanical Engineering, University of Texas, San Antonio, TX78249, USA
Sudheer R. Bhimireddy
Affiliation:
Department of Mechanical Engineering, University of Texas, San Antonio, TX78249, USA
*
†Email address for correspondence: kiran.bhaganagar@utsa.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

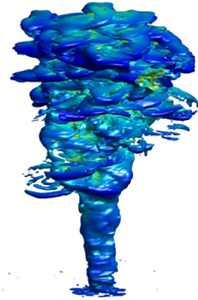
A fundamental study has been conducted to understand the mean and turbulence characteristics and their role in mixing processes of starting turbulent plumes initiated by a heated area source and released into a neutral atmosphere. Large eddy simulation has been performed in a domain of size 7 km (vertical) and $4~\text {km} \times 4~\text {km}$ (horizontal) for five different cases with surface heat flux varying from 0.5–2.5 km s$^{-1}$. With increasing buoyancy flux, the plume Reynolds number $Re$ (based on the maximum centreline velocity) increases from $6 \times 10^{7}\text {--}10^{8}$; however, the Froude number $Fr$ does not increase monotonically with increasing $B_o$, it varies from 1.091–1.149. Key physics that contributes to mixing and entrainment of ambient fluid into the plume has been investigated. The buoyancy- and shear-production of turbulence kinetic energy contributes to the mixing processes within the plume. The characteristic features of a starting plume are: the presence of a spiralling columnar neck region surrounding the central core that extends to $5D$ ($D$ is the source diameter), and which is capped with an unsteady head region that actively entrains fluid due to instabilities at the plume interface. Formation of vortex rings and instability mechanisms are linked to the entrainment process. Unsteady entrainment has been calculated using first principles, and the results revealed that entrainment, though negligible initially, initiates with the growth of the instabilities in the head region of the plume, and it correlates with the local Reynolds number in the head region.

JFM classification

Convection: Plumes/thermals Turbulent Flows: Turbulence simulation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 900 , 10 October 2020 , A32
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Arakawa, A. & Lamb, V. R. 1977 Computational design of the basic dynamical processes of the UCLA general circulation model. In General Circulation Models of the Atmosphere (ed. Chang, J.), Methods in Computational Physics: Advances in Research and Applications, vol. 17, pp. 173–265. Elsevier.CrossRefGoogle Scholar
Baines, P. G. 2001 Mixing in flows down gentle slopes into stratified environments. J. Fluid Mech. 443, 237–270.CrossRefGoogle Scholar
Batchelor, G. K. 1954 Heat convection and buoyancy effects in fluids. Q. J. R. Meteorolog. Soc. 80 (345), 339–358.CrossRefGoogle Scholar
Batchelor, G. K. 2000 An Introduction to Fluid Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Bhaganagar, K. 2017 Role of head of turbulent 3-d density currents in mixing during slumping regime. Phys. Fluids 29 (2), 020703.CrossRefGoogle Scholar
Bhaganagar, K. & Bhimireddy, S. R. 2017 Assessment of the plume dispersion due to chemical attack on April 4, 2017, in Syria. Nat. Hazards 88 (3), 1893–1901.CrossRefGoogle Scholar
Bhaganagar, K. & Pillalamarri, N. R. 2017 Lock-exchange release density currents over three-dimensional regular roughness elements. J. Fluid Mech. 832, 793–824.CrossRefGoogle Scholar
Bhamidipati, N. & Woods, A. W. 2017 On the dynamics of starting plumes. J. Fluid Mech. 833.CrossRefGoogle Scholar
Briggs, G. A. 1972 Chimney plumes in neutral and stable surroundings. Atmos. Environ. 6 (7), 507–510.CrossRefGoogle Scholar
Buckingham, S., Koloszar, L., Bartosiewicz, Y. & Winckelmans, G. 2017 Optimized temperature perturbation method to generate turbulent inflow conditions for LES/DNS simulations. Comput. Fluids 154, 44–59.CrossRefGoogle Scholar
Carazzo, G., Kaminski, E. & Tait, S. 2006 The route to self-similarity in turbulent jets and plumes. J. Fluid Mech. 547, 137–148.CrossRefGoogle Scholar
Carazzo, G., Kaminski, E. & Tait, S. 2008 On the rise of turbulent plumes: quantitative effects of variable entrainment for submarine hydrothermal vents, terrestrial and extra terrestrial explosive volcanism. J. Geophys. Res. Solid Earth 113, 94–103.CrossRefGoogle Scholar
Deardorff, J. W. 1980 Stratocumulus-capped mixed layers derived from a three-dimensional model. Boundary-Layer Meteorol. 18 (4), 495–527.CrossRefGoogle Scholar
Devenish, B. J., Rooney, G. G. & Thomson, D. J. 2010 Large-eddy simulation of a buoyant plume in uniform and stably stratified environments. J. Fluid Mech. 652, 75–103.CrossRefGoogle Scholar
Epstein, M. & Burelbach, J. P. 2001 Vertical mixing above a steady circular source of buoyancy. Int. J. Heat Mass Transfer 44 (3), 525–536.CrossRefGoogle Scholar
Ernst, G. G. J., Sparks, R. S. J., Carey, S. N. & Bursik, M. I. 1996 Sedimentation from turbulent jets and plumes. J. Geophys. Res. Solid Earth 101 (B3), 5575–5589.CrossRefGoogle Scholar
Ezzamel, A., Salizzoni, P. & Hunt, G. R. 2015 Dynamical variability of axisymmetric buoyant plumes. J. Fluid Mech. 765, 576–611.CrossRefGoogle Scholar
Fanneløp, T. K., Hirschberg, S. & Küffer, J. 1991 Surface current and recirculating cells generated by bubble curtains and jets. J. Fluid Mech. 229, 629–657.CrossRefGoogle Scholar
Fanneløp, T. K. & Webber, D. M. 2003 On buoyant plumes rising from area sources in a calm environment. J. Fluid Mech. 497, 319–334.CrossRefGoogle Scholar
Fischer, H. B., List, J. E., Koh, C. R., Imberger, J. & Brooks, N. H. 2013 Mixing in Inland and Coastal Waters. Elsevier.Google Scholar
Forthofer, J. M. & Goodrick, S. L. 2011 Review of vortices in wildland fire. J. Combust. 2011, 984363.CrossRefGoogle Scholar
Fric, T. F. & Roshko, A. 1994 Vortical structure in the wake of a transverse jet. J. Fluid Mech. 279, 1–47.CrossRefGoogle Scholar
Friedl, M. J., Härtel, C. & Fannelop, T. K. 1999 An experimental study of starting plumes over area sources. Il Nuovo Cimento 22 (6), 835–846.Google Scholar
Fromm, M. D. & Servranckx, R. 2003 Transport of forest fire smoke above the tropopause by supercell convection. Geophys. Res. Lett. 30 (10), L016820.CrossRefGoogle Scholar
Garratt, J. R. 1994 The atmospheric boundary layer. Earth-Sci. Rev. 37 (1–2), 89–134.CrossRefGoogle Scholar
George, W. K., Alpert, R. L. & Tamanini, F. 1977 Turbulence measurements in an axisymmetric buoyant plume. Int. J. Heat Mass Transfer 20 (11), 1145–1154.CrossRefGoogle Scholar
Holt, T. & Raman, S. 1988 A review and comparative evaluation of multilevel boundary layer parameterizations for first-order and turbulent kinetic energy closure schemes. Rev. Geophys. 26 (4), 761–780.CrossRefGoogle Scholar
Hunt, G. R. & Kaye, N. G. 2001 Virtual origin correction for lazy turbulent plumes. J. Fluid Mech. 435, 377–396.CrossRefGoogle Scholar
Hunt, G. R. & Kaye, N. B. 2005 Lazy plumes. J. Fluid Mech. 533, 329–338.CrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Kahn, R. A., Chen, Y., Nelson, D. L., Leung, F., Li, Q., Diner, D. J. & Logan, J. A. 2008 Wildfire smoke injection heights: two perspectives from space. Geophys. Res. Lett. 35 (4), L04809.CrossRefGoogle Scholar
Kaminski, E., Tait, S. & Carazzo, G. 2005 Turbulent entrainment in jets with arbitrary buoyancy. J. Fluid Mech. 526, 361–376.CrossRefGoogle Scholar
Kaye, N. B. & Hunt, G. R. 2009 An experimental study of large area source turbulent plumes. Int. J. Heat Fluid Flow 30 (6), 1099–1105.CrossRefGoogle Scholar
Kotsovinos, N. E. & List, E. J. 1977 Plane turbulent buoyant jets. Part 1. Integral properties. J. Fluid Mech. 81 (1), 25–44.CrossRefGoogle Scholar
Laprise, R. 1992 The Euler equations of motion with hydrostatic pressure as an independent variable. Mon. Weath. Rev. 120 (1), 197–207.2.0.CO;2>CrossRefGoogle Scholar
Linden, P. F., Batchelor, G. K., Moffatt, H. K. & Worster, M. G. 2000 Convection in the environment. In Perspectives in Fluid Dynamics: A Collective Introduction to Current Research (ed. Batchelor, G. K., Moffatt, H. K. & Worster, M. G.), pp. 289–345. Cambridge University Press.Google Scholar
Marjanovic, G., Taub, G. N. & Balachandar, S. 2019 On the effects of buoyancy on higher order moments in lazy plumes. J. Turbul. 20 (2), 121–146.CrossRefGoogle Scholar
Moeng, C. H. 1984 A large-eddy-simulation model for the study of planetary boundary-layer turbulence. J. Atmos. Sci. 41 (13), 2052–2062.2.0.CO;2>CrossRefGoogle Scholar
Morton, B. R. 1957 Buoyant plumes in a moist atmosphere. J. Fluid Mech. 2 (2), 127–144.CrossRefGoogle Scholar
Morton, B. R. 1959 Forced plumes. J. Fluid Mech. 5 (1), 151–163.CrossRefGoogle Scholar
Morton, B. R., Taylor, G. I. & Turner, J. S. 1956 Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. Lond. A 234 (1196), 1–23.Google Scholar
O'hern, T. J., Weckman, E. J., Gerhart, A. L., Tieszen, S. R. & Schefer, R. W. 2005 Experimental study of a turbulent buoyant helium plume. J. Fluid Mech. 544, 143–171.CrossRefGoogle Scholar
Özgökmen, T. M., Johns, W. E., Peters, H. & Matt, S. 2003 Turbulent mixing in the red sea outflow plume from a high-resolution nonhydrostatic model. J. Phys. Oceanogr. 33 (8), 1846–1869.CrossRefGoogle Scholar
Panchapakesan, N. R. & Lumley, J. L. 1993 Turbulence measurements in axisymmetric jets of air and helium. Part 2. Helium jet. J. Fluid Mech. 246, 225–247.CrossRefGoogle Scholar
Papanicolau, P. N. & List, E. J. 1988 Investigations of round vertical turbulent buoyant jets. J. Fluid Mech. 195 (341–391), 168.Google Scholar
Pham, M. V., Plourde, F. & Doan, K. S. 2005 Three-dimensional characterization of a pure thermal plume. Trans. ASME: J. Heat Transfer 127 (6), 624–636.CrossRefGoogle Scholar
Pham, M. V., Plourde, F. & Doan, K. S. 2007 Direct and large-eddy simulations of a pure thermal plume. Phys. Fluids 19 (12), 125103.CrossRefGoogle Scholar
Plourde, F., Pham, M. V., Doan, K. S. & Balachandar, S. 2008 Direct numerical simulations of a rapidly expanding thermal plume: structure and entrainment interaction. J. Fluid Mech. 604, 99–123.CrossRefGoogle Scholar
Ramaprian, B. R. & Chandrasekhara, M. S. 1989 Measurements in vertical plane turbulent plumes. Trans. ASME: J. Fluids Engng 111 (1), 69–77.Google Scholar
Schlüter, J. U., Pitsch, H. & Moin, P. 2004 Large-eddy simulation inflow conditions for coupling with Reynolds-averaged flow solvers. AIAA J. 42 (3), 478–484.CrossRefGoogle Scholar
Schultz, A., Delaney, J. R. & McDuff, R. E. 1992 On the partitioning of heat flux between diffuse and point source seafloor venting. J. Geophys. Res. Solid Earth 97 (B9), 12299–12314.CrossRefGoogle Scholar
Shabbir, A. & George, W. K. 1994 Experiments on a round turbulent buoyant plume. J. Fluid Mech. 275, 1–32.CrossRefGoogle Scholar
Skamarock, W. C., Klemp, J. B., Dudhia, J., Gill, D. O., Barker, D. M., Wang, W. & Powers, J. G. 2008 A description of the advanced research WRF version 3. NCAR Technical Note-475+ STR. National Center for Atmospheric Research.Google Scholar
Skyllingstad, E. D. & Denbo, D. W. 2001 Turbulence beneath sea ice and leads: a coupled sea ice/large-eddy simulation study. J. Geophys. Res. Oceans 106 (C2), 2477–2497.CrossRefGoogle Scholar
Taub, G. N., Lee, H., Balachandar, S. & Sherif, S. A. 2015 An examination of the high-order statistics of developing jets, lazy and forced plumes at various axial distances from their source. J. Turbul. 16 (10), 950–978.CrossRefGoogle Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. MIT.CrossRefGoogle Scholar
Turner, J. S. 1962 The ‘starting plume’ in neutral surroundings. J. Fluid Mech. 13 (3), 356–368.CrossRefGoogle Scholar
Turner, J. S. 1969 Buoyant plumes and thermals. Annu. Rev. Fluid Mech. 1 (1), 29–44.CrossRefGoogle Scholar
Van Reeuwijk, M, Salizzoni, P., Hunt, G. R. & Craske, J. 2016 Turbulent transport and entrainment in jets and plumes: a DNS study. Phys. Rev. Fluids 1 (7), 074301.CrossRefGoogle Scholar
Wang, H. & Law, A. W. 2002 Second-order integral model for a round turbulent buoyant jet. J. Fluid Mech. 459, 397–428.CrossRefGoogle Scholar
Wicker, L. J. & Skamarock, W. C. 2002 Time-splitting methods for elastic models using forward time schemes. Mon. Weath. Rev. 130 (8), 2088–2097.2.0.CO;2>CrossRefGoogle Scholar
Widell, K., Fer, I. & Haugan, P. M. 2006 Salt release from warming sea ice. Geophys. Res. Lett. 33 (12), L12501.CrossRefGoogle Scholar
Woods, A. W. 2010 Turbulent plumes in nature. Annu. Rev. Fluid Mech. 42, 391–412.CrossRefGoogle Scholar
Wu, Xiaohua 2017 Inflow turbulence generation methods. Annu. Rev. Fluid Mech. 49, 23–49.CrossRefGoogle Scholar
Yang, C. & Cai, X. 2014 A scalable fully implicit compressible Euler solver for mesoscale nonhydrostatic simulation of atmospheric flows. SIAM J. Sci. Comput. 36 (5), S23–S47.CrossRefGoogle Scholar