Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Rosti, Marco E.
Izbassarov, Daulet
Tammisola, Outi
Hormozi, Sarah
and
Brandt, Luca
2018.
Turbulent channel flow of an elastoviscoplastic fluid.
Journal of Fluid Mechanics,
Vol. 853,
Issue. ,
p.
488.
Rosti, Marco E.
Brandt, Luca
and
Mitra, Dhrubaditya
2018.
Rheology of suspensions of viscoelastic spheres: Deformability as an effective volume fraction.
Physical Review Fluids,
Vol. 3,
Issue. 1,
Rosti, Marco E.
Brandt, Luca
and
Pinelli, Alfredo
2018.
Turbulent channel flow over an anisotropic porous wall – drag increase and reduction.
Journal of Fluid Mechanics,
Vol. 842,
Issue. ,
p.
381.
Ahmadi, Somayeh
Roccon, Alessio
Zonta, Francesco
and
Soldati, Alfredo
2018.
Turbulent Drag Reduction by a Near Wall Surface Tension Active Interface.
Flow, Turbulence and Combustion,
Vol. 100,
Issue. 4,
p.
979.
Rosti, Marco Edoardo
Banaei, Arash Alizad
Brandt, Luca
and
Mazzino, Andrea
2018.
Flexible Fiber Reveals the Two-Point Statistical Properties of Turbulence.
Physical Review Letters,
Vol. 121,
Issue. 4,
Izbassarov, Daulet
Rosti, Marco E.
Ardekani, M. Niazi
Sarabian, Mohammad
Hormozi, Sarah
Brandt, Luca
and
Tammisola, Outi
2018.
Computational modeling of multiphase viscoelastic and elastoviscoplastic flows.
International Journal for Numerical Methods in Fluids,
Vol. 88,
Issue. 12,
p.
521.
Wood, J.N.
Breuer, M.
and
De Nayer, G.
2018.
Experimental studies on the instantaneous fluid–structure interaction of an air-inflated flexible membrane in turbulent flow.
Journal of Fluids and Structures,
Vol. 80,
Issue. ,
p.
405.
Rosti, Marco E.
and
Brandt, Luca
2018.
Suspensions of deformable particles in a Couette flow.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 262,
Issue. ,
p.
3.
De Vita, F.
Rosti, M.E.
Izbassarov, D.
Duffo, L.
Tammisola, O.
Hormozi, S.
and
Brandt, L.
2018.
Elastoviscoplastic flows in porous media.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 258,
Issue. ,
p.
10.
Ardekani, M. N.
Rosti, M. E.
and
Brandt, L.
2019.
Turbulent flow of finite-size spherical particles in channels with viscous hyper-elastic walls.
Journal of Fluid Mechanics,
Vol. 873,
Issue. ,
p.
410.
Benschop, H. O. G.
Greidanus, A. J.
Delfos, R.
Westerweel, J.
and
Breugem, W.-P.
2019.
Deformation of a linear viscoelastic compliant coating in a turbulent flow.
Journal of Fluid Mechanics,
Vol. 859,
Issue. ,
p.
613.
Shahmardi, Armin
Zade, Sagar
Ardekani, Mehdi N.
Poole, Rob J.
Lundell, Fredrik
Rosti, Marco E.
and
Brandt, Luca
2019.
Turbulent duct flow with polymers.
Journal of Fluid Mechanics,
Vol. 859,
Issue. ,
p.
1057.
Jain, Suhas S.
Kamrin, Ken
and
Mani, Ali
2019.
A conservative and non-dissipative Eulerian formulation for the simulation of soft solids in fluids.
Journal of Computational Physics,
Vol. 399,
Issue. ,
p.
108922.
Xia, Qian-Jin
Huang, Wei-Xi
and
Xu, Chun-Xiao
2019.
Direct numerical simulation of a turbulent boundary layer over an anisotropic compliant wall.
Acta Mechanica Sinica,
Vol. 35,
Issue. 2,
p.
384.
Chattopadhyay, Geetanjali
Sahu, Kirti Chandra
and
Usha, R.
2019.
Spatio-temporal instability of two superposed fluids in a channel with boundary slip.
International Journal of Multiphase Flow,
Vol. 113,
Issue. ,
p.
264.
Rosti, Marco E.
De Vita, Francesco
and
Brandt, Luca
2019.
Numerical simulations of emulsions in shear flows.
Acta Mechanica,
Vol. 230,
Issue. 2,
p.
667.
Józsa, T. I.
Balaras, E.
Kashtalyan, M.
Borthwick, A. G. L.
and
Viola, I. M.
2019.
Active and passive in-plane wall fluctuations in turbulent channel flows.
Journal of Fluid Mechanics,
Vol. 866,
Issue. ,
p.
689.
Rosti, Marco E.
Ge, Zhouyang
Jain, Suhas S.
Dodd, Michael S.
and
Brandt, Luca
2019.
Droplets in homogeneous shear turbulence.
Journal of Fluid Mechanics,
Vol. 876,
Issue. ,
p.
962.
Ghosh, Souvik
Loiseau, Jean-Christophe
Breugem, Wim-Paul
and
Brandt, Luca
2019.
Modal and non-modal linear stability of Poiseuille flow through a channel with a porous substrate.
European Journal of Mechanics - B/Fluids,
Vol. 75,
Issue. ,
p.
29.
Alghalibi, Dhiya
Rosti, Marco E.
and
Brandt, Luca
2019.
Inertial migration of a deformable particle in pipe flow.
Physical Review Fluids,
Vol. 4,
Issue. 10,
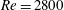 $Re=2800$ and examine the effect of different elasticity and viscosity of the deformable wall. We show that the skin friction increases monotonically with the material elastic modulus. The turbulent flow in the channel is affected by the moving wall even at low values of elasticity since non-zero fluctuations of vertical velocity at the interface influence the flow dynamics. The near-wall streaks and the associated quasi-streamwise vortices are strongly reduced near a highly elastic wall while the flow becomes more correlated in the spanwise direction, similarly to what happens for flows over rough and porous walls. As a consequence, the mean velocity profile in wall units is shifted downwards when shown in logarithmic scale, and the slope of the inertial range increases in comparison to that for the flow over a rigid wall. We propose a correlation between the downward shift of the inertial range, its slope and the wall-normal velocity fluctuations at the wall, extending results for the flow over rough walls. We finally show that the interface deformation is determined by the fluid fluctuations when the viscosity of the elastic layer is low, while when this is high the deformation is limited by the solid properties.
$Re=2800$ and examine the effect of different elasticity and viscosity of the deformable wall. We show that the skin friction increases monotonically with the material elastic modulus. The turbulent flow in the channel is affected by the moving wall even at low values of elasticity since non-zero fluctuations of vertical velocity at the interface influence the flow dynamics. The near-wall streaks and the associated quasi-streamwise vortices are strongly reduced near a highly elastic wall while the flow becomes more correlated in the spanwise direction, similarly to what happens for flows over rough and porous walls. As a consequence, the mean velocity profile in wall units is shifted downwards when shown in logarithmic scale, and the slope of the inertial range increases in comparison to that for the flow over a rigid wall. We propose a correlation between the downward shift of the inertial range, its slope and the wall-normal velocity fluctuations at the wall, extending results for the flow over rough walls. We finally show that the interface deformation is determined by the fluid fluctuations when the viscosity of the elastic layer is low, while when this is high the deformation is limited by the solid properties.