Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Dechamps, Xavier
Rasquin, Michel
Degrez, Gérard
and
Jansen, Kenneth
2013.
Study of Incompressible MHD Flow in a Circular Pipe with Transverse Magnetic Field using a Spectral/Finite Element Solver.
Li, Yongqi
and
Zikanov, Oleg
2013.
Laminar pipe flow at the entrance into transverse magnetic field.
Fusion Engineering and Design,
Vol. 88,
Issue. 4,
p.
195.
Krasnov, Dmitry
Thess, André
Boeck, Thomas
Zhao, Yurong
and
Zikanov, Oleg
2013.
Patterned Turbulence in Liquid Metal Flow: Computational Reconstruction of the Hartmann Experiment.
Physical Review Letters,
Vol. 110,
Issue. 8,
Tympel, Saskia
Boeck, Thomas
and
Schumacher, Jörg
2013.
Laminar and transitional liquid metal duct flow near a magnetic point dipole.
Journal of Fluid Mechanics,
Vol. 735,
Issue. ,
p.
553.
Hussam, Wisam K.
and
Sheard, Gregory J.
2013.
Heat transfer in a high Hartmann number MHD duct flow with a circular cylinder placed near the heated side-wall.
International Journal of Heat and Mass Transfer,
Vol. 67,
Issue. ,
p.
944.
Zikanov, Oleg
Listratov, Yaroslav I.
and
Sviridov, Valentin G.
2013.
Natural convection in horizontal pipe flow with a strong transverse magnetic field.
Journal of Fluid Mechanics,
Vol. 720,
Issue. ,
p.
486.
Boeck, Thomas
and
Krasnov, Dmitry
2014.
A mixing-length model for side layers of magnetohydrodynamic channel and duct flows with insulating walls.
Physics of Fluids,
Vol. 26,
Issue. 2,
Zhao, Yurong
Tao, Jianjun
and
Zikanov, Oleg
2014.
Transition to two-dimensionality in magnetohydrodynamic turbulent Taylor-Couette flow.
Physical Review E,
Vol. 89,
Issue. 3,
Zikanov, Oleg
Krasnov, Dmitry
Li, Yongqi
Boeck, Thomas
and
Thess, André
2014.
Patterned turbulence in spatially evolving magnetohydrodynamic duct and pipe flows.
Theoretical and Computational Fluid Dynamics,
Vol. 28,
Issue. 3,
p.
319.
Lv, Xinyuan
and
Zikanov, Oleg
2014.
Mixed convection in horizontal duct flow with transverse magnetic field and heating of side wall.
Physics of Fluids,
Vol. 26,
Issue. 9,
p.
097106.
Zikanov, Oleg
Krasnov, Dmitry
Boeck, Thomas
Thess, Andre
and
Rossi, Maurice
2014.
Laminar-Turbulent Transition in Magnetohydrodynamic Duct, Pipe, and Channel Flows.
Applied Mechanics Reviews,
Vol. 66,
Issue. 3,
Boeck, Thomas
and
Krasnov, Dmitry
2014.
A mixing‐length model for magnetohydrodynamic flows in channels and ducts with wall‐parallel magnetic field.
PAMM,
Vol. 14,
Issue. 1,
p.
661.
Zhang, Xuan
and
Zikanov, Oleg
2014.
Mixed convection in a horizontal duct with bottom heating and strong transverse magnetic field.
Journal of Fluid Mechanics,
Vol. 757,
Issue. ,
p.
33.
Hamid, Ahmad H. A.
Hussam, Wisam K.
Pothérat, Alban
and
Sheard, Gregory J.
2015.
Spatial evolution of a quasi-two-dimensional Kármán vortex street subjected to a strong uniform magnetic field.
Physics of Fluids,
Vol. 27,
Issue. 5,
Zhang, Xuan
and
Zikanov, Oleg
2015.
Two-dimensional turbulent convection in a toroidal duct of a liquid metal blanket of a fusion reactor.
Journal of Fluid Mechanics,
Vol. 779,
Issue. ,
p.
36.
Stelzer, Zacharias
Miralles, Sophie
Cébron, David
Noir, Jérôme
Vantieghem, Stijn
and
Jackson, Andrew
2015.
Experimental and numerical study of electrically driven magnetohydrodynamic flow in a modified cylindrical annulus. II. Instabilities.
Physics of Fluids,
Vol. 27,
Issue. 8,
Smolentsev, S.
Badia, S.
Bhattacharyay, R.
Bühler, L.
Chen, L.
Huang, Q.
Jin, H.-G.
Krasnov, D.
Lee, D.-W.
de les Valls, E. Mas
Mistrangelo, C.
Munipalli, R.
Ni, M.-J.
Pashkevich, D.
Patel, A.
Pulugundla, G.
Satyamurthy, P.
Snegirev, A.
Sviridov, V.
Swain, P.
Zhou, T.
and
Zikanov, O.
2015.
An approach to verification and validation of MHD codes for fusion applications.
Fusion Engineering and Design,
Vol. 100,
Issue. ,
p.
65.
Shen, Yuxin
and
Zikanov, Oleg
2016.
Thermal convection in a liquid metal battery.
Theoretical and Computational Fluid Dynamics,
Vol. 30,
Issue. 4,
p.
275.
Bandaru, Vinodh
Boeck, Thomas
and
Schumacher, Jörg
2016.
Hartmann duct flow at moderate magnetic Reynolds numbers.
PAMM,
Vol. 16,
Issue. 1,
p.
577.
Cassells, Oliver G.W.
Hussam, Wisam K.
and
Sheard, Gregory J.
2016.
Heat transfer enhancement using rectangular vortex promoters in confined quasi-two-dimensional magnetohydrodynamic flows.
International Journal of Heat and Mass Transfer,
Vol. 93,
Issue. ,
p.
186.
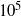 and the Hartmann number varies from
and the Hartmann number varies from 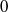 to
to  . It is found that there is a broad range of Hartmann numbers in which the flow is neither laminar nor fully turbulent, but has laminar core, Hartmann boundary layers and turbulent zones near the walls parallel to the magnetic field. Analysis of turbulent fluctuations shows that each zone consists of two layers: the boundary layer near the wall characterized by small-scale turbulence and the outer layer dominated by large-scale vortical structures strongly elongated in the direction of the magnetic field. We also find a peculiar scaling of the mean velocity, according to which the reciprocal von Kármán coefficient grows nearly linearly with the distance to the wall.
. It is found that there is a broad range of Hartmann numbers in which the flow is neither laminar nor fully turbulent, but has laminar core, Hartmann boundary layers and turbulent zones near the walls parallel to the magnetic field. Analysis of turbulent fluctuations shows that each zone consists of two layers: the boundary layer near the wall characterized by small-scale turbulence and the outer layer dominated by large-scale vortical structures strongly elongated in the direction of the magnetic field. We also find a peculiar scaling of the mean velocity, according to which the reciprocal von Kármán coefficient grows nearly linearly with the distance to the wall.

