Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-27T08:55:13.240Z Has data issue: false hasContentIssue false
  • English
  • Français

On boundary-layer receptivity to entropy waves

Published online by Cambridge University Press:  25 October 2021

A.I. Ruban Open the ORCID record for A.I. Ruban [Opens in a new window] ,
S.K. Keshari  and
M.A. Kravtsova
A.I. Ruban*
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
S.K. Keshari
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
M.A. Kravtsova
Affiliation:
Department of Mathematics, Imperial College London, 180 Queen's Gate, LondonSW7 2AZ, UK
*
†Email address for correspondence: a.ruban@imperial.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

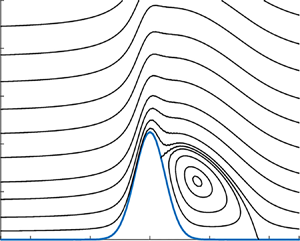
In this paper, we consider the generation of the Tollmien–Schlichting waves in the boundary layer on the surface of a wing exposed to entropy waves. It is well known that the free-stream turbulence is composed of two perturbation modes: the vorticity waves and the entropy waves. The receptivity of the boundary layer to the vorticity waves has been studied extensively by various authors. The entropy waves have not attracted such attention. We show that, in high speed subsonic flows, the entropy waves are as important for the receptivity as the vorticity waves. Methodologically, our work relies on the asymptotic analysis of the Navier–Stokes equations at large values of the Reynolds number, which results in the formulation of a suitably modified triple-deck theory. The entropy waves produce oscillations of the gas temperature and density, but the velocity and the pressure remain unperturbed to the leading order. This precludes the entropy waves from penetrating the boundary layer, as happens, for example, with the acoustic waves. Our analysis reveals that the entropy waves decay rapidly in the transition layer that forms near the outer edge of the boundary layer. We find that an entropy wave alone cannot generate the Tollmien–Schlichting waves. However, when the boundary layer encounters a wall roughness, the flow near the roughness appears to be perturbed not only inside the boundary layer but also in the inviscid region outside the boundary layer. The latter comes into the interaction with the density perturbations in the entropy wave. As a result, a localised ‘forcing’ is created that produces the Tollmien–Schlichting waves. In this paper we present the results of a linear and nonlinear receptivity analysis. We find that the nonlinearity enhances the receptivity significantly, especially when a local separation region forms on the roughness.

JFM classification

Aerodynamics: High-speed flow Boundary Layers: Boundary layer receptivity
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 929 , 25 December 2021 , A17
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Brennan, G.S., Gajjar, J.S.B. & Hewitt, R.E. 2021 Tollmien–Schlichting wave cancellation via localised heating elements in boundary layers. J. Fluid Mech. 909, A16.CrossRefGoogle Scholar
De Tullio, N. & Ruban, A.I. 2015 A numerical evaluation of the asymptotic theory of receptivity for subsonic compressible boundary layers. J. Fluid Mech. 771, 520–546.CrossRefGoogle Scholar
Denier, J.P., Hall, P. & Seddougui, S.O. 1991 On the receptivity problem for Görtler vortices: vortex motion induced by wall roughness. Phil. Trans. R. Soc. A 335, 51–85.Google Scholar
Dong, M., Liu, Y. & Wu, X. 2020 Receptivity of inviscid modes in supersonic boundary layers due to scattering of free-stream sound by localised wall roughness. J. Fluid Mech. 896, A23.CrossRefGoogle Scholar
Duck, P.W., Ruban, A.I. & Zhikharev, C.N. 1996 The generation of Tollmien–Schlichting waves by free-stream turbulence. J. Fluid Mech. 312, 341–371.CrossRefGoogle Scholar
Goldstein, M.E. 1985 Scattering of acoustic waves into Tollmien–Schlichting waves by small streamwise variation in surface geometry. J. Fluid Mech. 154, 509–529.CrossRefGoogle Scholar
Gulyaev, A.N., Kozlov, V.E., Kuznetsov, V.R., Mineev, B.I. & Sekundov, A.N. 1989 Interaction of a laminar boundary layer with external disturbances. Izv. Akad. Nauk SSSR, Mekh. Zhidk. Gaza 5, 55–65.Google Scholar
Kachanov, Y.S., Kozlov, V.V. & Levchenko, V.Y. 1982 The Apearence of Turbulence in the Boundary Layer. Nauka.Google Scholar
Kerimbekov, R.M. & Ruban, A.I. 2005 Receptivity of boundary layers with to distributed wall vibrations. Phil. Trans. R. Soc. A 363, 1145–1155.CrossRefGoogle ScholarPubMed
Kovasznay, L.S.J. 1953 Turbulence in supersonic flow. J. Aero. Sci. 20 (10), 657–682.CrossRefGoogle Scholar
Kravtsova, M.A., Zametaev, V.B. & Ruban, A.I. 2005 An effective numerical method for solving viscous-inviscid interaction problems. Phil. Trans. R. Soc. A 363 (1830), 1157–1167.CrossRefGoogle ScholarPubMed
Lin, C.C. 1946 On the stability of two-dimensional parallel flows. Part 3. Stabilty in a viscous fluid. Q. Appl. Maths 3, 277–301.CrossRefGoogle Scholar
Messiter, A.F. 1970 Boundary-layer flow near the trailing edge of a flat plate. SIAM J. Appl. Maths 18 (1), 241–257.CrossRefGoogle Scholar
Neiland, V.Y. 1969 Theory of laminar boundary layer separation in supersonic flow. Izv. Akad. Nauk SSSR, Mech. Zhidk. Gaza 4, 53–57.Google Scholar
Prandtl, L. 1904 Über flüssigkeitsbewegung bei sehr kleiner Reibung. In Verh. III. Intern. Math. Kongr., Heidelberg, pp. 484–491. Teubner, Leipzig, 1905.Google Scholar
Ruban, A.I. 1984 On Tollmien–Schlichting wave generation by sound. Izv. Akad. Nauk SSSR, Mekh. Zhid. Gaza, 44–52.Google Scholar
Ruban, A.I. 2018 Fluid Dynamics. Part 3. Boundary Layers. Oxford University Press.Google Scholar
Ruban, A.I., Bernots, T. & Kravtsova, M.A. 2016 Linear and nonlinear receptivity of the boundary layer in transonic flows. J. Fluid Mech. 786, 154–189.CrossRefGoogle Scholar
Ruban, A.I., Bernots, T. & Pryce, D. 2013 Receptivity of the boundary layer to vibrations of the wing surface. J. Fluid Mech. 723, 480–528.CrossRefGoogle Scholar
Schubauer, G.B. & Skramstad, H.K. 1948 Laminar-boundary-layer oscillations and transition on a flat plate. Tech. Rep. NACA TR 909.Google Scholar
Smith, F.T. 1979 On the non-parallel flow stability of the Blasius boundary layer. Proc. R. Soc. Lond. A 366, 91–109.Google Scholar
Stewartson, K. 1969 On the flow near the trailing edge of a flat plate. Mathematika 16 (1), 106–121.CrossRefGoogle Scholar
Stewartson, K. 1970 On laminar boundary layers near corners. Q. J. Mech. Appl. Maths 23 (2), 137–152.CrossRefGoogle Scholar
Stewartson, K. & Williams, P.G. 1969 Self-induced separation. Proc. R. Soc. Lond. A 312, 181–206.Google Scholar
Terent'ev, E.D. 1981 Linear problem for a vibrator in subsonic boundary layer. Prikl. Mat. Mech. 45, 1049–1055.Google Scholar
Wu, X. 1999 Generation of Tollmien–Schlichting waves by convecting gusts interacting with sound. J. Fluid Mech. 397, 285–316.CrossRefGoogle Scholar
Wu, X. 2001 Receptivity of boundary layers with distributed roughness to vortical and acoustic disturbances; a second order asymptotic theory and comparison with experiments. J. Fluid Mech. 431, 91–133.CrossRefGoogle Scholar
Wu, X., Zhao, D. & Luo, J. 2011 Excitation of steady and unsteady Görtler vortices by free-stream vortical disturbances. J. Fluid Mech. 682, 66–100.CrossRefGoogle Scholar
Zhuk, V.I. & Ryzhov, O.S. 1980 Free interaction and stability of boundary layer in incompressible fluid flow. Proc. Akad. Nauk SSSR 253, 1326–1329.Google Scholar