Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Wang, Bo-Fu
Zhou, Lin
Wan, Zhen-Hua
Ma, Dong-Jun
and
Sun, De-Jun
2016.
Stability analysis of Rayleigh-Bénard convection in a cylinder with internal heat generation.
Physical Review E,
Vol. 94,
Issue. 1,
Renaudière de Vaux, Sébastien
Zamansky, Rémi
Bergez, Wladimir
Tordjeman, Philippe
and
Haquet, Jean-François
2017.
Destabilization of a liquid metal by nonuniform Joule heating.
Physical Review E,
Vol. 96,
Issue. 3,
Köllner, Thomas
Boeck, Thomas
and
Schumacher, Jörg
2017.
Thermal Rayleigh-Marangoni convection in a three-layer liquid-metal-battery model.
Physical Review E,
Vol. 95,
Issue. 5,
Xiang, Linyan
and
Zikanov, Oleg
2017.
Subcritical convection in an internally heated layer.
Physical Review Fluids,
Vol. 2,
Issue. 6,
Kim, Joo‐Hong
Moon, Woosok
Wells, Andrew J.
Wilkinson, Jeremy P.
Langton, Tom
Hwang, Byongjun
Granskog, Mats A.
and
Rees Jones, David W.
2018.
Salinity Control of Thermal Evolution of Late Summer Melt Ponds on Arctic Sea Ice.
Geophysical Research Letters,
Vol. 45,
Issue. 16,
p.
8304.
Mahajan, Amit
Ram, Paras
and
Makinde, Oluwole Daniel
2018.
Penetrative Internally Heated Convection in Magnetic Fluids.
Defect and Diffusion Forum,
Vol. 387,
Issue. ,
p.
373.
Toppaladoddi, Srikanth
and
Wettlaufer, John S.
2018.
Penetrative convection at high Rayleigh numbers.
Physical Review Fluids,
Vol. 3,
Issue. 4,
Vjatkin, A.A.
Kozlov, V.G.
and
Sabirov, R.R.
2019.
Convection of a heat-generating fluid in a rotating cylindrical cavity subject to transverse vibrations.
International Journal of Thermal Sciences,
Vol. 137,
Issue. ,
p.
560.
Nandal, Reena
and
Mahajan, Amit
2019.
Nonlinear stability analysis of a fluid saturated anisotropic Darcy–Brinkman medium with internal heat source.
Applied Mathematics and Computation,
Vol. 358,
Issue. ,
p.
216.
Volkov, Sergey
Bogdanov, Sergey
Zdorovennov, Roman
Zdorovennova, Galina
Terzhevik, Arkady
Palshin, Nicolay
Bouffard, Damien
and
Kirillin, Georgiy
2019.
Fine scale structure of convective mixed layer in ice-covered lake.
Environmental Fluid Mechanics,
Vol. 19,
Issue. 3,
p.
751.
Волков, С. Ю.
Богданов, С. Р.
ЗДОРОВЕННОВА, Г. Э.
Здоровеннов, Р.Э.
Пальшин, Н.И.
and
ТЕРЖЕВИК, А. Ю.
2019.
Крупномасштабная структура конвективного перемешанного слоя в мелководном озере подо льдом, "Фундаментальная и прикладная гидрофизика".
Фундаментальная и прикладная гидрофизика,
p.
30.
Wang, Qi
Zhou, Quan
Wan, Zhen-Hua
and
Sun, De-Jun
2019.
Penetrative turbulent Rayleigh–Bénard convection in two and three dimensions.
Journal of Fluid Mechanics,
Vol. 870,
Issue. ,
p.
718.
Pratt, J.
Baraffe, I.
Goffrey, T.
Geroux, C.
Constantino, T.
Folini, D.
and
Walder, R.
2020.
Comparison of 2D and 3D compressible convection in a pre-main sequence star.
Astronomy & Astrophysics,
Vol. 638,
Issue. ,
p.
A15.
Miquel, Benjamin
Bouillaut, Vincent
Aumaître, Sébastien
and
Gallet, Basile
2020.
On the role of the Prandtl number in convection driven by heat sources and sinks.
Journal of Fluid Mechanics,
Vol. 900,
Issue. ,
Yoshikawa, Harunori N.
Kang, Changwoo
Mutabazi, Inoccent
Zaussinger, Florian
Haun, Peter
and
Egbers, Christoph
2020.
Thermoelectrohydrodynamic convection in parallel plate capacitors under dielectric heating conditions.
Physical Review Fluids,
Vol. 5,
Issue. 11,
Creyssels, M.
2020.
Model for classical and ultimate regimes of radiatively driven turbulent convection.
Journal of Fluid Mechanics,
Vol. 900,
Issue. ,
Wang, Qi
Lohse, Detlef
and
Shishkina, Olga
2021.
Scaling in Internally Heated Convection: A Unifying Theory.
Geophysical Research Letters,
Vol. 48,
Issue. 4,
Ding, Zijing
and
Wu, Jian
2021.
Coherent heat transport in two-dimensional penetrative Rayleigh–Bénard convection.
Journal of Fluid Mechanics,
Vol. 920,
Issue. ,
Smirnov, Sergei
Smirnovsky, Alexander
and
Bogdanov, Sergey
2021.
The Emergence and Identification of Large-Scale Coherent Structures in Free Convective Flows of the Rayleigh-Bénard Type.
Fluids,
Vol. 6,
Issue. 12,
p.
431.
Du, Yuhang
Zhang, Mengqi
and
Yang, Yantao
2021.
Two-component convection flow driven by a heat-releasing concentration field.
Journal of Fluid Mechanics,
Vol. 929,
Issue. ,
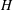 $H$ ) without convection, grows proportionally to
$H$ ) without convection, grows proportionally to  $H^{4/5}$ when convection is strong in both two and three dimensions. The ratio of the heating rate to the fluid temperature is likened to the Nusselt number of Rayleigh–Bénard convection. Simulations are reported for Prandtl numbers between 0.1 and 10 and for Rayleigh numbers (defined in terms of the heating rate) up to
$H^{4/5}$ when convection is strong in both two and three dimensions. The ratio of the heating rate to the fluid temperature is likened to the Nusselt number of Rayleigh–Bénard convection. Simulations are reported for Prandtl numbers between 0.1 and 10 and for Rayleigh numbers (defined in terms of the heating rate) up to 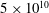 $5\times 10^{10}$ .
$5\times 10^{10}$ .

