Article contents
Pressure–strain terms in Langmuir turbulence
Published online by Cambridge University Press: 07 October 2019
Abstract
This study investigates the pressure–strain tensor ( $\unicode[STIX]{x1D72B}$) in Langmuir turbulence. The pressure–strain tensor is determined from large-eddy simulations (LES), and is partitioned into components associated with the mean current shear (rapid), the Stokes shear and the turbulent–turbulent (slow) interactions. The rapid component can be parameterized using existing closure models, although the coefficients in the closure models are particular to Langmuir turbulence. A closure model for the Stokes component is proposed, and it is shown to agree with results from the LES. The slow component of
$\unicode[STIX]{x1D72B}$) in Langmuir turbulence. The pressure–strain tensor is determined from large-eddy simulations (LES), and is partitioned into components associated with the mean current shear (rapid), the Stokes shear and the turbulent–turbulent (slow) interactions. The rapid component can be parameterized using existing closure models, although the coefficients in the closure models are particular to Langmuir turbulence. A closure model for the Stokes component is proposed, and it is shown to agree with results from the LES. The slow component of 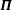 $\unicode[STIX]{x1D72B}$ does not agree with existing ‘return-to-isotropy’ closure models for five of the six components of the Reynolds stress tensor, and a new closure model is proposed that accounts for these deviations which vary systematically with Langmuir number,
$\unicode[STIX]{x1D72B}$ does not agree with existing ‘return-to-isotropy’ closure models for five of the six components of the Reynolds stress tensor, and a new closure model is proposed that accounts for these deviations which vary systematically with Langmuir number,  $La_{t}$, and depth. The implications of these results for second- and first-order closures of Langmuir turbulence are discussed.
$La_{t}$, and depth. The implications of these results for second- and first-order closures of Langmuir turbulence are discussed.
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 6
- Cited by


