Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-28T20:43:44.376Z Has data issue: false hasContentIssue false
  • English
  • Français

Reconnection of skewed vortices

Published online by Cambridge University Press:  20 June 2014

Y. Kimura  and
H. K. Moffatt
Y. Kimura
Affiliation:
Graduate School of Mathematics, Nagoya University, Chikusa-ku, Nagoya 464-8602, Japan
H. K. Moffatt*
Affiliation:
Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Wilberforce Road, Cambridge CB3 0WA, UK
*
†Email address for correspondence: hkm2@damtp.cam.ac.uk
Get access Rights & Permissions [Opens in a new window]

Abstract

Based on experimental evidence that vortex reconnection commences with the approach of nearly antiparallel segments of vorticity, a linearised model is developed in which two Burgers-type vortices are driven together and stretched by an ambient irrotational strain field induced by more remote vorticity. When these Burgers vortices are exactly antiparallel, they are annihilated on the strain time-scale, independent of kinematic viscosity $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\nu $ in the limit 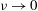$\nu \rightarrow 0$. When the vortices are skew to each other, they are annihilated under this action over a local extent that increases exponentially in the stretching direction, with clear evidence of reconnection on the same strain time-scale. The initial helicity associated with the skewed geometry is eliminated during the process of reconnection. The model applies equally to the reconnection of weak magnetic flux tubes under the action of a strain field, when Lorentz forces are negligible.

JFM classification

Vortex Flows: Vortex Flows Vortex Flows: Vortex interactions
Type
Papers
Information
Journal of Fluid Mechanics , Volume 751 , 25 July 2014 , pp. 329 - 345
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Boratav, O. N., Pelz, R. B. & Zabusky, N. J. 1992 Reconnection in orthogonally interacting vortex tubes: direct numerical simulations and quantifications. Phys. Fluids A 4, 581–605.CrossRefGoogle Scholar
Buntine, J. D. & Pullin, D. I. 1989 Merger and cancellation of strained vortices. J. Fluid Mech. 205, 263–295.CrossRefGoogle Scholar
Burgers, J. M. 1948 A mathematical model illustrating the theory of turbulence. Adv. Appl. Mech. 1, 171–199.CrossRefGoogle Scholar
Douady, S., Couder, Y. & Brachet, M. E. 1991 Direct observation of the intermittency of intense vorticity filaments in turbulence. Phys. Rev. Lett. 67, 983–986.CrossRefGoogle ScholarPubMed
Hattori, Y. & Moffatt, H. K. 2005 Reconnexion of vortex and magnetic tubes subject to an imposed strain: an approach by perturbation expansion. Fluid Dyn. Res. 26, 333–356.CrossRefGoogle Scholar
Hunt, J. C. R. & Carruthers, D. J. 1990 Rapid distortion theory and the ‘problems’ of turbulence. J. Fluid Mech. 212, 497–532.CrossRefGoogle Scholar
Ishihara, T., Kaneda, Y., Yokokawa, M., Itakura, K. & Uno, A. 2007 Small-scale statistics in high-resolution direct numerical simulation of turbulence: Reynolds number dependence of one-point velocity gradient statistics. J. Fluid Mech. 592, 335–366.CrossRefGoogle Scholar
Kerr, R. M. 2013 Swirling, turbulent vortex rings formed from a chain reaction of reconnection events. Phys. Fluids 25, 065101.CrossRefGoogle Scholar
Kida, S. & Takaoka, M. 1987 Bridging in vortex reconnection. Phys. Fluids 30, 2911–2914.CrossRefGoogle Scholar
Kida, S. & Takaoka, M. 1994 Vortex reconnection. Annu. Rev. Fluid Mech. 26, 169–189.CrossRefGoogle Scholar
Kimura, Y. & Koikari, S. 2004 Particle transport by a vortex soliton. J. Fluid Mech. 510, 201–218.CrossRefGoogle Scholar
Kleckner, D. & Irvine, W. T. M. 2013 Creation and dynamics of knotted vortices. Nat. Phys. 9, 253–258; see also http://www.newton.ac.uk/programmes/TOD/seminars/2012091111302.html.CrossRefGoogle Scholar
Kudela, H. & Kosior, A. 2013 Parallel computation of vortex tube reconnection using a graphics card and the 3D vortex-in-cell method. Proc. IUTAM 7, 59–66.CrossRefGoogle Scholar
Lundgren, T. S. 1982 Strained spiral vortex model for turbulent fine structure. Phys. Fluids 25, 2192–2203.CrossRefGoogle Scholar
Melander, M. V. & Hussain, F. 1989 Cross-linking of two antiparallel vortex tubes. Phys. Fluids A 1, 633–636.CrossRefGoogle Scholar
Moffatt, H. K. 1969 The degree of knottedness of tangled vortex lines. J. Fluid Mech. 35, 117–129.CrossRefGoogle Scholar
Moffatt, H. K. & Hunt, R. E. 2002 A model for magnetic reconnection. In Tubes, Sheets and Singularities in Fluid Dynamics (ed. Moffatt, H. K. & Bajer, K.), pp. 125–138. Kluwer.Google Scholar
Moffatt, H. K., Kida, S. & Ohkitani, K. 1994 Stretched vortices – the sinews of turbulence; large-Reynolds-number asymptotics. J. Fluid Mech. 259, 241–264.CrossRefGoogle Scholar
Moffatt, H. K. & Ricca, R. L. 1992 Helicity and the Călugăreanu invariant. Proc. R. Soc. Lond. A 439, 411–429.Google Scholar
Priest, E. R. & Forbes, T. 2000 Magnetic Reconnection. Cambridge University Press.CrossRefGoogle Scholar
Pullin, D. I. & Saffman, P. G. 1998 Vortex dynamics in turbulence. Annu. Rev. Fluid Mech. 30, 31–51.CrossRefGoogle Scholar
van Rees, W. M., Hussain, F. & Koumoutsakos, P. 2012 Vortex tube reconnection at $Re = 104$ . Phys. Fluids 24, 075105.CrossRefGoogle Scholar
Rott, N. 1958 On the viscous core of a line vortex. Z. Angew. Math. Phys. 9, 543–553.CrossRefGoogle Scholar
Taylor, J. B. 1974 Relaxation of toroidal plasma and generation of reverse magnetic fields. Phys. Rev. Lett. 33, 1139–1141.CrossRefGoogle Scholar
Saffman, P. G. 1990 A model of vortex reconnection. J. Fluid Mech. 212, 395–402.CrossRefGoogle Scholar
Townsend, A. A. 1951 On the fine-scale structure of turbulence. Proc. R. Soc. Lond. A 208, 534–542.Google Scholar
Vincent, A. & Meneguzzi, M. 1991 The spatal structure and statistical properties of homogeneous turbulence. J. Fluid Mech. 225, 1–25.CrossRefGoogle Scholar
Wright, A. N. & Berger, M. A. 1989 The effect of reconnection upon the linkage and interior structure of magnetic flux tubes. J. Geophys. Res. Space Phys. 94, 1295–1302.CrossRefGoogle Scholar