Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-28T05:55:15.410Z Has data issue: false hasContentIssue false
  • English
  • Français

Reynolds number and aspect ratio effects on the leading-edge vortex for rotating insect wing planforms

Published online by Cambridge University Press:  01 February 2013

R. R. Harbig ,
J. Sheridan  and
M. C. Thompson
R. R. Harbig*
Affiliation:
Fluids Laboratory for Aeronautical and Industrial Research (FLAIR), Department of Mechanical and Aerospace Engineering, Monash University, Clayton, VIC 3800, Australia
J. Sheridan
Affiliation:
Fluids Laboratory for Aeronautical and Industrial Research (FLAIR), Department of Mechanical and Aerospace Engineering, Monash University, Clayton, VIC 3800, Australia
M. C. Thompson
Affiliation:
Fluids Laboratory for Aeronautical and Industrial Research (FLAIR), Department of Mechanical and Aerospace Engineering, Monash University, Clayton, VIC 3800, Australia
*
†Email address for correspondence: robert.harbig@monash.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

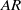Previous studies investigating the effect of aspect ratio ($\mathit{AR}$) for insect-like regimes have reported seemingly different trends in aerodynamic forces, however no detailed flow observations have been made. In this study, the effect of 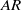$\mathit{AR}$ and Reynolds number on the flow structures over insect-like wings is explored using a numerical model of an altered fruit fly wing revolving at a constant angular velocity. Increasing the Reynolds number for an 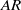$\mathit{AR}$ of 2.91 resulted in the development of a dual leading-edge vortex (LEV) structure, however increasing 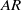$\mathit{AR}$ at a fixed Reynolds number generated the same flow structures. This result shows that the effects of Reynolds number and $\mathit{AR}$ are linked. We present an alternative scaling using wing span as the characteristic length to decouple the effects of Reynolds number from those of 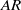$\mathit{AR}$. This results in a span-based Reynolds number, which can be used to independently describe the development of the LEV. Indeed, universal behaviour was found for various parameters using this scaling. The effect of 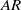$\mathit{AR}$ on the vortex structures and aerodynamic forces was then assessed at different span-based Reynolds numbers. Scaling the flow using the wing span was found to apply when a strong spanwise velocity is present on the leeward side of the wing and therefore may prove to be useful for similar studies involving flapping or rotating wings at high angles of attack.

JFM classification

Biological Fluid Dynamics: Biological Fluid Dynamics Biological Fluid Dynamics: Swimming/flying
Type
Papers
Information
Journal of Fluid Mechanics , Volume 717 , 25 February 2013 , pp. 166 - 192
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ansari, S. A., Knowles, K. & Zbikowski, R. 2008 Insectlike flapping wings in the hover part 2: effect of wing geometry. J. Aircraft 45 (6), 1976–1990.CrossRefGoogle Scholar
Aono, H., Liang, F. & Liu, H. 2008 Near- and far-field aerodynamics in insect hovering flight: an integrated computational study. J. Expl Biol. 211, 239–257.CrossRefGoogle ScholarPubMed
Billant, P., Chomaz, J. M. & Huerre, P. 1998 Experimental study of vortex breakdown in swirling jets. J. Fluid Mech. 376, 183–219.CrossRefGoogle Scholar
Birch, J. M. & Dickinson, M. H. 2001 Spanwise flow and the attachment of the leading-edge vortex on insect wings. Nature 412, 729–733.CrossRefGoogle Scholar
Birch, J. M., Dickson, W. B. & Dickinson, M. H. 2004 Force production and flow structure of the leading edge vortex on flapping wings at high and low Reynolds numbers. J. Expl Biol. 207 (7), 1063–1072.CrossRefGoogle Scholar
Dickinson, M. H. & Gotz, K. G. 1993 Unsteady aerodynamic performance of model wings at low Reynolds numbers. J. Expl Biol. 174 (1), 45–65.CrossRefGoogle Scholar
Dickinson, M. H., Lehmann, F.-O. & Sane, S. P. 1999 Wing rotation and the aerodynamic basis of insect flight. Science 284, 1954–1960.CrossRefGoogle ScholarPubMed
Ellington, C. P. 1984 The aerodynamics of hovering insect flight. II. Morphological parameters. Phil. Trans. R. Soc. B: Biol. Sci. 305 (1122), 17–40.Google Scholar
Ellington, C. P., van den Berg, C., Willmott, A. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384 (19), 626–630.CrossRefGoogle Scholar
Graftieaux, L., Michard, M. & Grosjean, N. 2001 Combining PIV, POD and vortex identification algorithms for the study of unsteady turbulent swirling flows. Meas. Sci. Technol. 12, 1422–1429.CrossRefGoogle Scholar
Hall, M. G. 1972 Vortex breakdown. Annu. Rev. Fluid Mech. 4 (1), 195–218.CrossRefGoogle Scholar
Hunt, J. C. R., Wray, A. A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. Tech. Rep. CTR-S88. Center for Turbulence Research.Google Scholar
Jones, A. R. & Babinsky, H. 2010 Unsteady lift generation on rotating wings at low Reynolds numbers. J. Aircraft 47 (3), 1013–1021.CrossRefGoogle Scholar
Jones, A. R. & Babinsky, H. 2011 Reynolds number effects on leading edge vortex development on a waving wing. Exp. Fluids 51 (1), 197–210.CrossRefGoogle Scholar
Kweon, J. & Choi, H. 2010 Sectional lift coefficient of a flapping wing in hovering motion. Phys. Fluids 22 (7).CrossRefGoogle Scholar
Leibovich, S. 1978 The structure of vortex breakdown. Annu. Rev. Fluid Mech. 10, 221–246.CrossRefGoogle Scholar
Lentink, D. & Dickinson, M. H. 2009a Biofluiddynamic scaling of flapping, spinning and translating fins and wings. J. Expl Biol. 212 (16), 2691–2704.CrossRefGoogle ScholarPubMed
Lentink, D. & Dickinson, M. H. 2009b Rotational accelerations stabilize leading edge vortices on revolving fly wings. J. Expl Biol. 212 (16), 2705–2719.CrossRefGoogle ScholarPubMed
Liu, H. & Aono, H. 2009 Size effects on insect hovering aerodynamics: an integrated computational study. Bioinspir. Biomimet. 4.Google ScholarPubMed
Lu, Y., Shen, G. X. & Lai, G. J. 2006 Dual leading-edge vortices on flapping wings. J. Expl Biol. 209, 5005–5016.CrossRefGoogle ScholarPubMed
Luo, G. & Sun, M. 2005 The effects of corrugation and wing planform on the aerodynamic force production of sweeping model insect wings. Acta Mechanica Sin. 21 (6), 531–541.CrossRefGoogle Scholar
Maxworthy, T. 1979 Experiments on the Weis–Fogh mechanism of lift generation by insects in hovering flight. Part 1. Dynamics of the fling. J. Fluid Mech. 93 (01), 47–63.CrossRefGoogle Scholar
Miller, L. A. & Peskin, C. S. 2004 When vortices stick: an aerodynamic transition in tiny insect flight. J. Expl Biol. 207, 3073–3088.CrossRefGoogle ScholarPubMed
Ozen, C. A. & Rockwell, D. 2011 Flow structure on a rotating plate. Exp. Fluids 52, 207–223.CrossRefGoogle Scholar
Phillips, N., Knowles, K. & Lawson, N. J. 2010 Effect of wing planform shape on the flow structures of an insect-like flapping wing in Hover. In 27th International Congress of the Aeronautical Sciences. ICAS.Google Scholar
Pines, D. J. & Bohorquez, F. 2006 Challenges facing future micro-air-vehicle development. J. Aircraft 43 (2), 290–305.CrossRefGoogle Scholar
Poelma, C., Dickson, W. B. & Dickinson, M. H. 2006 Time-resolved reconstruction of the full velocity field around a dynamically-scaled flapping wing. Exp. Fluids 41 (2), 213–225.CrossRefGoogle Scholar
Roache, P. J. 1998 Verification of codes and calculations. AIAA J. 36 (5), 696–702.CrossRefGoogle Scholar
Shyy, W., Aono, H., Chimakurthi, S. K., Trizila, P., Kang, C.-K., Cesnik, C. E. S. & Liu, H. 2010 Recent progress in flapping wing aerodynamics and aeroelasticity. Prog. Aeronaut. Sci. 46 (7), 284–327.CrossRefGoogle Scholar
Srygley, R. B. & Thomas, A. L. R. 2002 Unconventional lift-generating mechanisms in free-flying butterflies. Nature 420, 660–664.CrossRefGoogle ScholarPubMed
Taira, K. & Colonius, T. 2009 Three-dimensional flows around low-aspect-ratio flat-plate wings at low Reynolds numbers. J. Fluid Mech. 623, 187–207.CrossRefGoogle Scholar
Tsuzuki, N., Sato, S. & Abe, T. 2007 Design guidelines of rotary wings in hover for insect-scale micro air vehicle applications. J. Aircraft 44 (1), 252–263.CrossRefGoogle Scholar
Usherwood, J. R. & Ellington, C. P. 2002a The aerodynamics of revolving wings I. Model hawkmoth wings. J. Expl Biol. 205 (11), 1547–1564.CrossRefGoogle ScholarPubMed
Usherwood, J. R. & Ellington, C. P. 2002b The aerodynamics of revolving wings II. Propeller force coefficients from mayfly to quail. J. Expl Biol. 205 (11), 1565–1576.CrossRefGoogle ScholarPubMed
Walker, J. D. A., Smith, C. R., Cerra, A. W. & Doligalski, T. L. 1987 The impact of a vortex ring on a wall. J. Fluid Mech. 181, 99–140.CrossRefGoogle Scholar
Zanker, J. M. & Gotz, K. G. 1990 The wing beat of drosophila melanogaster. II. Dynamics. Phil. Trans. R. Soc. B: Biol. Sci. 327, 19–44.Google Scholar