Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-30T22:15:34.910Z Has data issue: false hasContentIssue false
  • English
  • Français

The role of gravity in the prediction of the circular hydraulic jump radius for high-viscosity liquids

Published online by Cambridge University Press:  07 January 2019

Yunpeng Wang  and
Roger E. Khayat Open the ORCID record for Roger E. Khayat [Opens in a new window]
Yunpeng Wang
Affiliation:
Department of Mechanical and Materials Engineering, University of Western Ontario, London, Ontario, Canada N6A 5B9
Roger E. Khayat*
Affiliation:
Department of Mechanical and Materials Engineering, University of Western Ontario, London, Ontario, Canada N6A 5B9
*
†Email address for correspondence: rkhayat@uwo.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

The free-surface flow formed by a circular jet impinging on a stationary disk is analysed theoretically. We develop a simple and coherent model to predict the location and height of the jump for high-viscosity liquids. The study explores the effect of gravity in the supercritical flow. The formulation reduces to a problem, involving only one parameter: 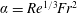$\unicode[STIX]{x1D6FC}=Re^{1/3}Fr^{2}$, where $Re$ and 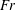$Fr$ are the Reynolds and Froude numbers based on the flow rate and the jet radius. We show that the jump location coincides with the singularity in the thin-film equation when gravity is included, suggesting that the jump location can be determined without the knowledge of downstream flow conditions such as the jump height, the radius of the disk, which corroborates earlier observations in the case of type I circular hydraulic jumps. Consequently, there is no need for a boundary condition downstream to determine the jump radius. Our results corroborate well existing measurements and numerical simulation. Our predictions also confirm the constancy of the Froude number 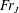$Fr_{J}$ based on the jump radius and height as suggested by the measurements of Duchesne et al. (Europhys. Lett., vol. 107, 2014, 54002). We establish theoretically the conditions for 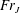$Fr_{J}$ to remain independent of the flow rate. The subcritical flow and the height of the hydraulic jump are sought subject to the thickness at the edge of the disk, comprising contributions based on the capillary length and minimum flow energy. The thickness at the edge of the disk appears to be negligibly small for high-viscosity liquids.

JFM classification

Boundary Layers: Boundary Layers Interfacial Flows (free surface): Interfacial Flows (free surface) Interfacial Flows (free surface): Thin films
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 862 , 10 March 2019 , pp. 128 - 161
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Avedisian, C. T. & Zhao, Z. 2000 The circular hydraulic jump in low gravity. Proc. R. Soc. Lond. A 456, 2127–2151.Google Scholar
Baonga, J. B., Gualous, H. L. & Imbert, M. 2006 Experimental study of hydrodynamic and heat transfer of free liquid jet impinging a flat circular heated disk. Appl. Therm. Engng 26, 1125–1138.Google Scholar
Benilov, E. S. 2015 Hydraulic jumps in a shallow flow down a slightly inclined substrate. J. Fluid Mech. 782, 5–24.Google Scholar
Bhagat, R. K., Jha, N. K., Linden, P. F. & Wilson, D. I. 2018 On the origin of the circular hydraulic jump in a thin liquid film. J. Fluid Mech. 851, R5.Google Scholar
Bohr, T., Dimon, P. & Putzkaradze, V. 1993 Shallow-water approach to the circular hydraulic jump. J. Fluid Mech. 254, 635–648.Google Scholar
Bohr, T., Ellegaard, C., Hansen, A. E. & Haaning, A. 1996 Hydraulic jumps, flow separation and wave breaking: an experimental study. Physica B 228, 1–10.Google Scholar
Bohr, T., Putkaradze, V. & Watanabe, S. 1997 Averaging theory for the structure of hydraulic jumps and separation in laminar free-surface flows. Phys. Rev. Lett. 79, 1038–1041.Google Scholar
Brechet, Y. & Neda, Z. 1999 On the circular hydraulic jump. Am. J. Phys. 67, 723–731.Google Scholar
Bush, J. W. M. & Aristoff, J. M. 2003 The influence of surface tension on the circular hydraulic jump. J. Fluid Mech. 489, 229–238.Google Scholar
Bush, J. W. M., Aristoff, J. M. & Hosoi, A. E. 2006 An experimental investigation of the stability of the circular hydraulic jump. J. Fluid Mech. 558, 33–52.Google Scholar
Craik, A., Latham, R., Fawkes, M. & Gibbon, P. 1981 The circular hydraulic jump. J. Fluid Mech. 112, 347–362.Google Scholar
Diversified Enterprises 2009 Surface Energy Data for PDMS (Polydimethylsiloxane) http://www.accudynetest.com/polymer_surface_data/polydimethylsiloxane.pdf.Google Scholar
Dressaire, E., Courbin, L., Crest, J. & Stone, H. A. 2010 Inertia dominated thin-film flows over microdecorated surfaces. Phys. Fluids 22, 073602.Google Scholar
Duchesne, A., Lebon, L. & Limat, L. 2014 Constant Froude number in a circular hydraulic jump and its implication on the jump radius selection. Europhys. Lett. 107, 54002.Google Scholar
Ellegaard, C., Hansen, A., Haaning, A., Hansen, K. & Bohr, T. 1996 Experimental results on flow separation and transitions in the circular hydraulic jump. Phys. Scr. T67, 105–110.Google Scholar
Ellegaard, C., Hansen, A. E., Haaning, A., Marcussen, A., Bohr, T., Hansen, J. L. & Watanabe, S. 1998 Creating corners in kitchen sink flows. Nature 392, 767–768.Google Scholar
Ellegaard, C, Hansen, A. E., Haaning, A., Hansen, K., Marcussen, A., Bohr, T., Hansen, J. L. & Watanabe, S. 1999 Polygonal hydraulic jumps. Nonlinearity 12, 1–7.Google Scholar
Hansen, S. H., Horluck, S., Zauner, D., Dimon, P., Ellegaard, C. & Creagh, S. C. 1997 Geometric orbits of surface waves from a circular hydraulic jump. Phys. Rev. E 55, 7048–7061.Google Scholar
Higuera, F. J. 1994 The hydraulic jump in a viscous laminar flow. J. Fluid Mech. 274, 69–92.Google Scholar
Kasimov, A. R. 2008 A stationary circular hydraulic jump, the limits of its existence and its gasdynamic analogue. J. Fluid Mech. 601, 189–198.Google Scholar
Kate, R. P., Das, P. K. & Chakraborty, S. 2007 Hydraulic jumps due to oblique impingement of circular liquid jets on a flat horizontal surface. J. Fluid Mech. 573, 247–263.Google Scholar
Khayat, R. E. 2016 Impinging planar jet flow and hydraulic jump on a horizontal surface with slip. J. Fluid Mech. 808, 258–289.Google Scholar
Khayat, R. E. & Kim, K. 2006 Thin-film flow of a viscoelastic fluid on an axisymmetric substrate of arbitrary shape. J. Fluid Mech. 552, 37–71.Google Scholar
Lienhard, J. 2006 Heat transfer by impingement of circular free-surface liquid jets. In 18th National & 7th ISHMT-ASME Heat and Mass Transfer Conference, pp. 1–17. IIT.Google Scholar
Liu, X. & Lienhard, J. 1993 The hydraulic jump in circular jet impingement and in other thin liquid films. Exp. Fluids 15, 108–116.Google Scholar
Liu, X., Gabour, L. A. & Lienhard, J. 1993 Stagnation-point heat transfer during impingement of laminar liquid jets: analysis including surface tension. Trans. ASME J. Heat Transfer 115, 99–105.Google Scholar
Lubarda, V. & Talke, K. A. 2011 Analysis of the equilibrium droplet shape based on an ellipsoidal droplet Model. Langmuir 27, 10705–10713.Google Scholar
Mohajer, B. & Li, R. 2015 Circular hydraulic jump on finite surfaces with capillary limit. Phys. Fluids 27, 117102.Google Scholar
Ozar, B., Cetegen, B. M. & Faghri, A. 2003 Experiments on the flow of a thin liquid film over a horizontal stationary and rotating disk surface. Exp. Fluids 34, 556–565.Google Scholar
Passandideh-Fard, M., Teymourtash, A. R. & Khavari, M. 2011 Numerical study of circular hydraulic jump using volume-of-fluid method. Trans. ASME J. Fluids Engng 133 (1), 011401.Google Scholar
Prince, J. F., Maynes, D. & Crockett, J. 2012 Analysis of laminar jet impingement and hydraulic jump on a horizontal surface with slip. Phys. Fluids 24, 102103.Google Scholar
Rao, A. & Arakeri, J. H. 1998 Integral analysis applied to radial film flows. Intl J. Heat Mass Transfer 41, 275–2767.Google Scholar
Rayleigh, Lord 1914 On the theory of long waves and bores. Proc. R. Soc. Lond. A 90 (619), 324–328.Google Scholar
Rojas, N., Argentina, M. & Tirapegui, E. 2010 Inertial lubrication theory. Phys. Rev. Lett. 104, 187801-4.Google Scholar
Rojas, N., Argentina, M. & Tirapegui, E. 2013 A progressive correction to the circular hydraulic jump scaling. Phys. Fluids 25, 042105.Google Scholar
Rojas, N. & Tirapegui, E. 2015 Harmonic solutions for polygonal hydraulic jumps in thin fluid films. J. Fluid Mech. 780, 99–119.Google Scholar
Stevens, J. & Webb, B. W. 1992 Measurements of the free surface flow structure under an impinging, free liquid jet. Trans. ASME J. Heat Transfer 114, 79–84.Google Scholar
Schlichtling, H. 2000 Boundary-layer Theory, 8th edn. Springer.Google Scholar
Tani, I. 1949 Water Jump in the Boundary Layer. J. Phys. Soc. Japan 4, 212–215.Google Scholar
Vicente, C. M. S., Andre, P. S. & Ferreira, R. A. S. 2012 Simple measurement of surface free energy using a web cam. Rev. Brasil. Ens. Fisica 34, 3312,1–5.Google Scholar
Wang, Y. & Khayat, R. E. 2018 Impinging jet flow and hydraulic jump on a rotating disk. J. Fluid Mech. 839, 525–560.Google Scholar
Watanabe, S., Putkaradze, V. & Bohr, T. 2003 Integral methods for shallow free-surface flows with separation. J. Fluid Mech. 480, 233–265.Google Scholar
Watson, E. 1964 The spread of a liquid jet over a horizontal plane. J. Fluid Mech. 20, 481–499.Google Scholar
White, F. 2006 Fundamentals of Fluid Mechanics. McGraw-Hill.Google Scholar
Yang, S. & Chen, C. 1992 Laminar film condensation on a finite-size horizontal plate with suction at the wall. Appl. Math. Model. 16, 325–329.Google Scholar
Yang, Y., Chen, C. & Hsu, P. 1997 Laminar film condensation on a finite-size wavy disk. Appl. Math. Model. 21, 139–144.Google Scholar
Zhao, J. & Khayat, R. E. 2008 Spread of a non-Newtonian liquid jet over a horizontal plate. J. Fluid Mech. 613, 411–443.Google Scholar