Published online by Cambridge University Press: 22 August 2017
We experimentally investigate scale-to-scale anisotropy from the integral to the dissipative scales in homogeneous turbulence. We employ an apparatus in which two facing arrays of randomly actuated air jets generate turbulence with negligible mean flow and shear, over a volume several times larger than the energy-containing eddy size. The Reynolds number based on the Taylor microscale is varied in the range 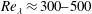 $Re_{\unicode[STIX]{x1D706}}\approx 300{-}500$, while the axial-to-radial ratio of the root mean square velocity fluctuations ranges between 1.38 and 1.72. Two velocity components are measured by particle image velocimetry at varying resolutions, capturing from the integral to the Kolmogorov scales and yielding statistics up to sixth order. Over the inertial range, the scaling exponents of the velocity structure functions are found to differ not only between the longitudinal and transverse components, but also between the axial and radial directions of separation. At the dissipative scales, the moments of the velocity gradients indicate that departure from isotropy is, at the present Reynolds numbers, significant and more pronounced for stronger large-scale anisotropy. The generalized flatness factors of the longitudinal velocity differences tend towards isotropy as the separation is reduced from the inertial to the near-dissipative scales (down to about
$Re_{\unicode[STIX]{x1D706}}\approx 300{-}500$, while the axial-to-radial ratio of the root mean square velocity fluctuations ranges between 1.38 and 1.72. Two velocity components are measured by particle image velocimetry at varying resolutions, capturing from the integral to the Kolmogorov scales and yielding statistics up to sixth order. Over the inertial range, the scaling exponents of the velocity structure functions are found to differ not only between the longitudinal and transverse components, but also between the axial and radial directions of separation. At the dissipative scales, the moments of the velocity gradients indicate that departure from isotropy is, at the present Reynolds numbers, significant and more pronounced for stronger large-scale anisotropy. The generalized flatness factors of the longitudinal velocity differences tend towards isotropy as the separation is reduced from the inertial to the near-dissipative scales (down to about 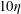 $10\unicode[STIX]{x1D702}$,
$10\unicode[STIX]{x1D702}$, 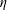 $\unicode[STIX]{x1D702}$ being the Kolmogorov length), but become more anisotropic for even smaller scales which are characterized by high intermittency. At the large scales, the direction of turbulence forcing is associated with a larger integral length, defined as the distance over which the velocity component in a given direction is spatially correlated. Because of anisotropy, the definition of the integral length is not trivial and may lead to dissimilar conclusions on the qualitative behaviour of the large scales and on the quantitative values of the normalized dissipation. Alternative definitions of these quantities are proposed to account for the anisotropy. Overall, these results highlight the importance of evaluating both the different velocity components and the different spatial directions across all scales of the flow.
$\unicode[STIX]{x1D702}$ being the Kolmogorov length), but become more anisotropic for even smaller scales which are characterized by high intermittency. At the large scales, the direction of turbulence forcing is associated with a larger integral length, defined as the distance over which the velocity component in a given direction is spatially correlated. Because of anisotropy, the definition of the integral length is not trivial and may lead to dissimilar conclusions on the qualitative behaviour of the large scales and on the quantitative values of the normalized dissipation. Alternative definitions of these quantities are proposed to account for the anisotropy. Overall, these results highlight the importance of evaluating both the different velocity components and the different spatial directions across all scales of the flow.