Hostname: page-component-745bb68f8f-b6zl4 Total loading time: 0 Render date: 2025-02-05T01:44:09.070Z Has data issue: false hasContentIssue false
  • English
  • Français

Shear-thickening suspensions down inclines: from Kapitza to Oobleck waves

Published online by Cambridge University Press:  22 March 2023

Baptiste Darbois Texier ,
Henri Lhuissier Open the ORCID record for Henri Lhuissier [Opens in a new window] ,
Bloen Metzger Open the ORCID record for Bloen Metzger [Opens in a new window]  and
Yoël Forterre Open the ORCID record for Yoël Forterre [Opens in a new window]
Baptiste Darbois Texier
Affiliation:
Aix-Marseille University, CNRS, IUSTI, 13453 Marseille, France Université Paris-Saclay, CNRS, FAST, 91405 Orsay, France
Henri Lhuissier
Affiliation:
Aix-Marseille University, CNRS, IUSTI, 13453 Marseille, France
Bloen Metzger
Affiliation:
Aix-Marseille University, CNRS, IUSTI, 13453 Marseille, France
Yoël Forterre*
Affiliation:
Aix-Marseille University, CNRS, IUSTI, 13453 Marseille, France
*
†Email address for correspondence: yoel.forterre@univ-amu.fr.
Get access Rights & Permissions [Opens in a new window]

Abstract

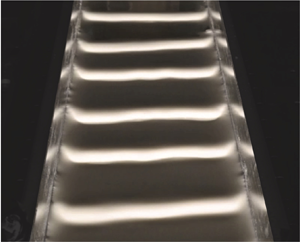
We investigate experimentally and theoretically the stability of a shear-thickening suspension flowing down an inclined plane. In a previous paper (Darbois Texier et al., Commun. Phys., vol. 3, 2020), we have shown that for particle volume fractions $\phi$ above the discontinuous shear-thickening fraction $\phi _{DST}$, long surface waves grow spontaneously at a flow Reynolds number much below 1. This motivated a simplified analysis based on a purely inertialess mechanism, called the ‘Oobleck waves’ mechanism, which couples the negatively sloped rheology of the suspension with the free-surface deflection and captures well the experimental instability threshold and the wave speed, for $\phi >\phi _{DST}$. However, neglecting inertia does not allow us to describe the inertial Kapitza regime observed for $\phi <\phi _{DST}$, nor does it allow us to discriminate between Oobleck waves and other inertial instabilities expected above $\phi _{DST}$. This paper fills this gap by extending our previous analysis, based on a depth-averaged approach and the Wyart–Cates constitutive shear-thickening rheology, to account for inertia. The extended analysis recovers quantitatively the experimental instability threshold in the Kapitza regime, below $\phi _{DST}$, and in the Oobleck waves regime, above $\phi _{DST}$. By providing additional measurements of the wave growth rate and investigating theoretically the effect of a strain delay in the rheology, it also confirms that the instability observed above $\phi _{DST}$ stems from the non-inertial Oobleck wave mechanism, which is specific to free-surface flows and dominates modes of inertial origin. These results emphasize the variety of instability mechanisms for shear-thickening suspensions and might be relevant to free-surface flows of other complex fluids displaying velocity-weakening rheology.

JFM classification

Complex Fluids: Suspensions Interfacial Flows (free surface): Thin films
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 959 , 25 March 2023 , A27
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Abdesselam, Y., Agassant, J.-F., Castellani, R., Valette, R., Demay, Y., Gourdin, D. & Peres, R. 2017 Rheology of plastisol formulations for coating applications. Polym. Engng Sci. 57 (9), 982–988.CrossRefGoogle Scholar
Allouche, M.H., Botton, V., Millet, S., Henry, D., Dagois-Bohy, S., Güzel, B. & Hadid, H.B. 2017 Primary instability of a shear-thinning film flow down an incline: experimental study. J. Fluid Mech. 821, R1.CrossRefGoogle Scholar
Balmforth, N.J., Bush, J.W.M. & Craster, R.V. 2005 Roll waves on flowing cornstarch suspensions. Phys. Lett. A 338 (6), 479–484.CrossRefGoogle Scholar
Balmforth, N.J. & Liu, J.J. 2004 Roll waves in mud. J. Fluid Mech. 519, 33–54.CrossRefGoogle Scholar
Benjamin, T.B. 1957 Wave formation in laminar flow down an inclined plane. J. Fluid Mech. 2 (6), 554–573.CrossRefGoogle Scholar
Blanco, E., Hodgson, D.J.M., Hermes, M., Besseling, R., Hunter, G.L., Chaikin, P.M., Cates, M.E., Van Damme, I. & Poon, W.C.K. 2019 Conching chocolate is a prototypical transition from frictionally jammed solid to flowable suspension with maximal solid content. Proc. Natl Acad. Sci. 116 (21), 10303–10308.CrossRefGoogle ScholarPubMed
Boersma, W.H., Baets, P.J.M., Lavèn, J. & Stein, H.N.J. 1991 Time-dependent behavior and wall slip in concentrated shear thickening dispersions. J. Rheol. 35 (6), 1093–1120.CrossRefGoogle Scholar
Bonnoit, C., Darnige, T., Clement, E. & Lindner, A. 2010 Inclined plane rheometry of a dense granular suspension. J. Rheol. 54 (1), 65–79.CrossRefGoogle Scholar
Carpen, I.C. & Brady, J.F. 2002 Gravitational instability in suspension flow. J. Fluid Mech. 472, 201–210.CrossRefGoogle Scholar
Chacko, R.N., Mari, R., Cates, M.E. & Fielding, S.M. 2018 Dynamic vorticity banding in discontinuously shear thickening suspensions. Phys. Rev. Lett. 121 (10), 108003.CrossRefGoogle ScholarPubMed
Clavaud, C., Bérut, A., Metzger, B. & Forterre, Y. 2017 Revealing the frictional transition in shear-thickening suspensions. Proc. Natl Acad. Sci. 114 (20), 5147–5152.CrossRefGoogle ScholarPubMed
Clavaud, C., Metzger, B. & Forterre, Y. 2020 The Darcytron: a pressure-imposed device to probe the frictional transition in shear-thickening suspensions. J. Rheol. 64, 395–403.CrossRefGoogle Scholar
Comtet, J., Chatté, G., Niguès, A., Bocquet, L., Siria, A. & Colin, A. 2017 Pairwise frictional profile between particles determines discontinuous shear thickening transition in non-colloidal suspensions. Nat. Commun. 8, 15633.CrossRefGoogle ScholarPubMed
Darbois Texier, B., Lhuissier, H., Forterre, Y. & Metzger, B. 2020 Surface-wave instability without inertia in shear-thickening suspensions. Commun. Phys. 3, 232.CrossRefGoogle Scholar
Dhas, D.J. & Roy, A. 2022 Stability of gravity-driven particle-laden flows – roles of shear-induced migration and normal stresses. J. Fluid Mech. 938, A29.CrossRefGoogle Scholar
Divoux, T., Fardin, M.A., Manneville, S. & Lerouge, S. 2016 Shear banding of complex fluids. Annu. Rev. Fluid Mech. 48, 81–103.CrossRefGoogle Scholar
Dong, J. & Trulsson, M. 2017 Analog of discontinuous shear thickening flows under confining pressure. Phys. Rev. Fluids 2 (8), 081301.CrossRefGoogle Scholar
Forterre, Y. 2006 Kapiza waves as a test for three-dimensional granular flow rheology. J. Fluid Mech. 563, 123–132.CrossRefGoogle Scholar
Forterre, Y. & Pouliquen, O. 2003 Long-surface-wave instability in dense granular flows. J. Fluid Mech. 486, 21–50.CrossRefGoogle Scholar
Freundlich, H. & Röder, H.L. 1938 Dilatancy and its relation to thixotropy. Trans. Faraday Soc. 34, 308–316.CrossRefGoogle Scholar
Gauthier, A., Pruvost, M., Gamache, O. & Colin, A. 2021 A new pressure sensor array for normal stress measurement in complex fluids. J. Rheol. 65 (4), 583–594.CrossRefGoogle Scholar
Goddard, J.D. 2003 Material instability in complex fluids. Annu. Rev. Fluid Mech. 35 (1), 113–133.CrossRefGoogle Scholar
Guazzelli, É. & Pouliquen, O. 2018 Rheology of dense granular suspensions. J. Fluid Mech. 852, P1.CrossRefGoogle Scholar
Guy, B.M., Hermes, M. & Poon, W.C.K. 2015 Towards a unified description of the rheology of hard-particle suspensions. Phys. Rev. Lett. 115 (8), 088304.CrossRefGoogle ScholarPubMed
Guy, B.M., Ness, C., Hermes, M., Sawiak, L.J., Sun, J. & Poon, W.C.K. 2020 Testing the Wyart–Cates model for non-Brownian shear thickening using bidisperse suspensions. Soft Matt. 16 (1), 229–237.CrossRefGoogle ScholarPubMed
Han, E., Wyart, M., Peters, I.R. & Jaeger, H.M. 2018 Shear fronts in shear-thickening suspensions. Phys. Rev. Fluids 3 (7), 073301.CrossRefGoogle Scholar
Hermes, M., Guy, B.M., Poon, W.C.K., Poy, G., Cates, M.E. & Wyart, M. 2016 Unsteady flow and particle migration in dense, non-Brownian suspensions. J. Rheol. 60 (5), 905–916.CrossRefGoogle Scholar
Hsu, C.-P., Ramakrishna, S.N., Zanini, M., Spencer, N.D. & Isa, L. 2018 Roughness-dependent tribology effects on discontinuous shear thickening. Proc. Natl Acad. Sci. 115 (20), 5117–5122.CrossRefGoogle ScholarPubMed
Hwang, C.-C., Chen, J.-L., Wang, J.-S. & Lin, J.-S. 1994 Linear stability of power law liquid film flows down an inclined plane. J. Phys. D: Appl. Phys. 27 (11), 2297–2301.CrossRefGoogle Scholar
Jeffreys, H. 1925 The flow of water in an inclined channel of rectangular section. Phil. Mag. 49 (293), 793–807.CrossRefGoogle Scholar
von Kann, S., Snoeijer, J.H., Lohse, D. & van der Meer, D. 2011 Nonmonotonic settling of a sphere in a cornstarch suspension. Phys. Rev. E 84 (6), 060401.CrossRefGoogle Scholar
von Kann, S., Snoeijer, J.H. & van der Meer, D. 2013 Velocity oscillations and stop–go cycles: the trajectory of an object settling in a cornstarch suspension. Phys. Rev. E 87 (4), 042301.CrossRefGoogle Scholar
Kapitza, P.L. & Kapitza, S.P. 1948 Wave flow of thin viscous fluid layers. Zh. Eksp. Teor. Fiz. 18 (1), 3–28.Google Scholar
LaFarge 2013 Superplasticizers: the wonder of fluid concrete. https://www.youtube.com/watch?v=CSZxjQwDKF0 38.Google Scholar
Landau, L.D. & Lifshitz, E.M. 2013 Fluid Mechanics: Landau and Lifshitz: Course of Theoretical Physics, vol. 6. Elsevier.Google Scholar
Lin, N.Y.C., Guy, B.M., Hermes, M., Ness, C., Sun, J., Poon, W.C.K. & Cohen, I. 2015 Hydrodynamic and contact contributions to continuous shear thickening in colloidal suspensions. Phys. Rev. Lett. 115 (22), 228304.CrossRefGoogle ScholarPubMed
Liu, K. & Mei, C.C. 1994 Roll waves on a layer of a muddy fluid flowing down a gentle slope – a Bingham model. Phys. Fluids 6 (8), 2577–2590.CrossRefGoogle Scholar
Lootens, D., Van Damme, H. & Hébraud, P. 2003 Giant stress fluctuations at the jamming transition. Phys. Rev. Lett. 90 (17), 178301.CrossRefGoogle ScholarPubMed
Mari, R., Seto, R., Morris, J.F. & Denn, M.M. 2014 Shear thickening, frictionless and frictional rheologies in non-Brownian suspensions. J. Rheol. 58 (6), 1693–1724.CrossRefGoogle Scholar
Mari, R., Seto, R., Morris, J.F. & Denn, M.M. 2015 a Discontinuous shear thickening in Brownian suspensions by dynamic simulation. Proc. Natl Acad. Sci. 112 (50), 15326–15330.CrossRefGoogle ScholarPubMed
Mari, R., Seto, R., Morris, J.F. & Denn, M.M. 2015 b Nonmonotonic flow curves of shear thickening suspensions. Phys. Rev. E 91 (5), 052302.CrossRefGoogle ScholarPubMed
Morris, J.F. 2018 Lubricated-to-frictional shear thickening scenario in dense suspensions. Phys. Rev. Fluids 3 (11), 110508.CrossRefGoogle Scholar
Nagahiro, S.-I., Nakanishi, H. & Mitarai, N. 2013 Experimental observation of shear thickening oscillation. Europhys. Lett. 104 (2), 28002.CrossRefGoogle Scholar
Nakanishi, H. & Mitarai, N. 2011 Shear thickening oscillation in a dilatant fluid. J. Phys. Soc. Japan 80 (3), 033801.CrossRefGoogle Scholar
Ng, C.-O. & Mei, C.C. 1994 Roll waves on a shallow layer of mud modelled as a power-law fluid. J. Fluid Mech. 263, 151–184.CrossRefGoogle Scholar
Olmsted, P.D. 1999 Two-state shear diagrams for complex fluids in shear flow. Europhys. Lett. 48 (3), 339–345.CrossRefGoogle Scholar
Olmsted, P.D. 2008 Perspectives on shear banding in complex fluids. Rheol. Acta 47 (3), 283–300.CrossRefGoogle Scholar
Ovarlez, G., Le, A.V.N., Smit, W.J., Fall, A., Mari, R., Chatté, G. & Colin, A. 2020 Density waves in shear-thickening suspensions. Sci. Adv. 6 (16), eaay5589.CrossRefGoogle ScholarPubMed
Ramaswamy, M., Griniasty, I., Liarte, D.B., Shetty, A., Katifori, E., Del Gado, E., Sethna, J.P., Chakraborty, B. & Cohen, I. 2021 Universal scaling of shear thickening transitions. arXiv:2107.13338v2.Google Scholar
Rathee, V., Blair, D.L. & Urbach, J.S. 2017 Localized stress fluctuations drive shear thickening in dense suspensions. Proc. Natl Acad. Sci. 114 (33), 8740–8745.CrossRefGoogle ScholarPubMed
Richards, J.A., Royer, J.R., Liebchen, B., Guy, B.M. & Poon, W.C.K. 2019 Competing timescales lead to oscillations in shear-thickening suspensions. Phys. Rev. Lett. 123 (3), 038004.CrossRefGoogle ScholarPubMed
Saint-Michel, B., Gibaud, T. & Manneville, S. 2018 Uncovering instabilities in the spatiotemporal dynamics of a shear-thickening cornstarch suspension. Phys. Rev. X 8 (3), 031006.Google Scholar
Sedes, O., Singh, A. & Morris, J.F. 2020 Fluctuations at the onset of discontinuous shear thickening in a suspension. J. Rheol. 64 (2), 309–319.CrossRefGoogle Scholar
Seto, R., Mari, R., Morris, J.F. & Denn, M.M. 2013 Discontinuous shear thickening of frictional hard-sphere suspensions. Phys. Rev. Lett. 111 (21), 218301.CrossRefGoogle ScholarPubMed
Singh, A., Mari, R., Denn, M.M. & Morris, J.F. 2018 A constitutive model for simple shear of dense frictional suspensions. J. Rheol. 62 (2), 457–468.CrossRefGoogle Scholar
Spenley, N.A., Yuan, X.F. & Cates, M.E. 1996 Nonmonotonic constitutive laws and the formation of shear-banded flows. J. Phys. II 6 (4), 551–571.Google Scholar
Trowbridge, J.H. 1987 Instability of concentrated free surface flows. J. Geophys. Res.: Oceans 92 (C9), 9523–9530.CrossRefGoogle Scholar
Whitham, G.B. 2011 Linear and Nonlinear Waves, vol. 42. John Wiley & Sons.Google Scholar
Wyart, M. & Cates, M.E. 2014 Discontinuous shear thickening without inertia in dense non-Brownian suspensions. Phys. Rev. Lett. 112 (9), 098302.CrossRefGoogle ScholarPubMed
Yerushalmi, J., Katz, S. & Shinnar, R. 1970 The stability of steady shear flows of some viscoelastic fluids. Chem. Engng Sci. 25 (12), 1891–1902.CrossRefGoogle Scholar
Yih, C.-S. 1963 Stability of liquid flow down an inclined plane. Phys. Fluids 6 (3), 321–334.CrossRefGoogle Scholar
Zarei, M. & Aalaie, J. 2020 Application of shear thickening fluids in material development. J. Mater. Res. Technol. 9 (5), 10411–10433.CrossRefGoogle Scholar