Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T07:16:17.588Z Has data issue: false hasContentIssue false
  • English
  • Français

Simulation of convection at a vertical ice face dissolving into saline water

Published online by Cambridge University Press:  31 May 2016

Bishakhdatta Gayen ,
Ross W. Griffiths  and
Ross C. Kerr
Bishakhdatta Gayen*
Affiliation:
Research School of Earth Sciences, Australian National University, Canberra, ACT 2601, Australia
Ross W. Griffiths
Affiliation:
Research School of Earth Sciences, Australian National University, Canberra, ACT 2601, Australia
Ross C. Kerr
Affiliation:
Research School of Earth Sciences, Australian National University, Canberra, ACT 2601, Australia
*
†Email address for correspondence: bishakhdatta.gayen@anu.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the convection and dissolution rate generated when a wall of ice dissolves into seawater under Antarctic Ocean conditions. In direct numerical simulations three coupled interface equations are used to solve for interface temperature, salinity and ablation velocity, along with the boundary layer flow and transport. The main focus is on ambient water temperatures between $-1\,^{\circ }\text{C}$ and 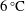$6\,^{\circ }\text{C}$ and salinities around 35 ‰, where diffusion of salt to the ice–water interface depresses the freezing point and enhances heat diffusion to the ice. We show that fluxes of both heat and salt to the interface are significant in governing the dissolution of ice, and the ablation velocity agrees well with experiments and a recent theoretical prediction. The same turbulent flow dynamics and ablation rate are expected to apply at any depth in a deeper ocean water column (after choosing the relevant pressure coefficient for the liquidus temperature). At Grashof numbers currently accessible by direct numerical simulation, turbulence is generated both directly from buoyancy flux and from shear production in the buoyancy-driven boundary layer flow, whereas shear production by the convective flow is expected to be more important at geophysical scales. The momentum balance in the boundary layer is dominated by buoyancy forcing and wall stress, with the latter characterised by a large drag coefficient.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 798 , 10 July 2016 , pp. 284 - 298
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Carey, V. P. & Gebhart, B. 1982 Transport near a vertical ice surface melting in saline water: experiments at low salinities. J. Fluid Mech. 117, 403–423.CrossRefGoogle Scholar
Cooper, P. & Hunt, G. R. 2010 The ventilated filing box containing a vertically distributed source of buoyancy. J. Fluid Mech. 646, 39–58.Google Scholar
Galton-Fenzi, B. K., Hunter, J. R., Coleman, R., Marsland, S. J. & Warner, R. C. 2012 Modeling the basal melting and marine ice accretion of the Amery Ice Shelf. J. Geophys. Res. 117, C09031.Google Scholar
Gayen, B., Griffiths, R. W., Hughes, G. O. & Saenz, J. A. 2013 Energetics of horizontal convection. J. Fluid Mech. 716, R10.CrossRefGoogle Scholar
George, W. K. & Capp, S. P. 1979 A theory for natural convection turbulent boundary layers next to heated vertical surfaces. Intl J. Heat Mass Transfer 22, 813–826.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Holland, D. M. & Jenkins, A. 1999 Modeling thermodynamic ice–ocean interactions at the base of an ice shelf. J. Phys. Oceanogr. 29, 1787–1800.Google Scholar
Holland, P. R., Jenkins, A. & Holland, D. M. 2008 The response of ice shelf basal melting to variations in ocean temperature. J. Clim. 21, 2558–2572.CrossRefGoogle Scholar
Huppert, H. E. & Josberger, E. G. 1980 The melting of ice in cold stratified water. J. Phys. Oceanogr. 10, 953–960.2.0.CO;2>CrossRefGoogle Scholar
Huppert, H. E. & Turner, J. S. 1978 On melting icebergs. Nature 271, 46–48.Google Scholar
Huppert, H. E. & Turner, S. J. 1980 Ice blocks melting into a salinity gradient. J. Fluid Mech. 100, 367–384.Google Scholar
Jenkins, A., Dutrieux, P., Jacobs, S. S., Mcphail, S. D., Perrett, J. R., Webb, A. T & White, D. 2010 Observations beneath Pine Island Glacier in West Antarctica and implications for its retreat. Nature Geosci. 3, 468–472.CrossRefGoogle Scholar
Johnson, R. S. & Mollendrof, J. C. 1984 Transport from a vertical ice surface in saline water. Intl J. Heat Mass Transfer 27, 1928–1932.Google Scholar
Josberger, E. G. & Martin, S. 1981 A laboratory and theoretical study of the boundary layer adjacent to a vertical melting ice wall in salt water. J. Fluid Mech. 111, 439–473.CrossRefGoogle Scholar
Kerr, R. C. 1994a Melting driven by vigorous compositional convection. J. Fluid Mech. 280, 255–285.Google Scholar
Kerr, R. C. 1994b Dissolving driven by vigorous compositional convection. J. Fluid Mech. 280, 287–302.Google Scholar
Kerr, R. C. & Mcconnochie, C. D. 2015 Dissolution of a vertical solid surface by turbulent compositional convection. J. Fluid Mech. 765, 211–228.Google Scholar
Klemp, J. B. & Durran, D. R. 1983 An upper boundary condition permitting internal gravity wave radiation in numerical mesoscale models. Mon. Weath. Rev. 111, 430–444.Google Scholar
Mcconnochie, C. D. & Kerr, R. C. 2016 The turbulent wall plume from a vertically distributed source of buoyancy. J. Fluid Mech. 787, 224–236.Google Scholar
Nilson, R. H. 1985 Countercurrent convection in a double-diffusive boundary layer. J. Fluid Mech. 160, 181–210.Google Scholar
Oerter, H., Kipfstuhl, J., Determann, J., Miller, H., Wagenbach, D., Minikin, A. & Graf, W. 1992 Evidence for basal marine ice in the Filchner–Ronne ice shelf. Nature 358, 399–401.Google Scholar
Payne, A. J., Vieli, A., Shepherd, A. P., Wingham, D. J. & Rignot, E. 2004 Recent dramatic thinning of largest West Antarctic ice stream triggered by oceans. Geophys. Res. Lett. 31, L23401.Google Scholar
Rignot, E., Bamber, J. L., Van Den Broeke, M. R., Davis, C., Li, Y. H., Van De Berg, W. J. & Van Meijgaard, E. 2008 Recent Antarctic ice mass loss from radar interferometry and regional climate modelling. Nature Geo. Sci. 1 (2), 106–110.CrossRefGoogle Scholar
Rignot, E. & Jacobs, S. S. 2002 Rapid bottom melting widespread near Antarctic ice sheet grounding lines. Science 296, 2020–2023.Google Scholar
Tsuji, T. & Nagano, Y. 1989 Velocity and temperature measurements in a natural convection boundary layer along a vertical flat plate. Exp. Therm. Fluid Sci. 2 (2), 208–215.Google Scholar
Turner, J. S. 1973 Buoyancy Effects on Fluids. Cambridge University Press.Google Scholar
Wells, A. J. & Worster, M. G. 2008 A geophysical-scale model of vertical natural convection boundary layers. J. Fluid Mech. 609, 111–137.Google Scholar
Wells, J. A. & Worster, G. M. 2011 Melting and dissolving of a vertical solid surface with laminar compositional convection. J. Fluid Mech. 687, 118–140.CrossRefGoogle Scholar
Woods, A. W. 1992 Melting and dissolving. J. Fluid Mech. 239, 429–448.Google Scholar