Hostname: page-component-6bf8c574d5-k2jvg Total loading time: 0 Render date: 2025-03-07T03:09:10.420Z Has data issue: false hasContentIssue false
  • English
  • Français

Smoothed particle hydrodynamics modelling of particle-size segregation in granular flows

Published online by Cambridge University Press:  24 January 2024

Chengwei Zhu Open the ORCID record for Chengwei Zhu [Opens in a new window] ,
Chong Peng  and
Wei Wu Open the ORCID record for Wei Wu [Opens in a new window]
Chengwei Zhu
Affiliation:
Research Center of Coastal and Urban Geotechnical Engineering, Zhejiang University, 310058 Hangzhou, PR China Engineering Research Center of Urban Underground Space Development of Zhejiang Province, 310058 Hangzhou, PR China
Chong Peng
Affiliation:
ESS Engineering Software Steyr GmbH, Berggasse 35, 4400 Steyr, Austria
Wei Wu*
Affiliation:
Institut für Geotechnik, Universität für Bodenkultur, Feistmantelstraße 4, 1180 Vienna, Austria
*
†Email address for correspondence: wei.wu@boku.ac.at
Get access Rights & Permissions [Opens in a new window]

Abstract

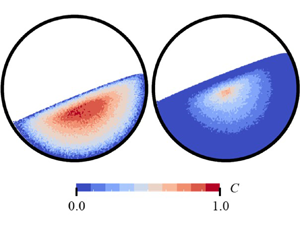
In this work, smoothed particle hydrodynamics (SPH) is employed to investigate the segregation evolution in granular flows. We first provide the Lagrangian description-based governing equations, including the linear momentum conservation and the segregation–diffusion equation. Then the hybrid continuum surface reaction scheme is introduced to formulate the concentration-related inhomogeneous Neumann boundary condition on the free and wall surfaces. We follow a two-stage strategy to advance boundary particle searching and normal direction identification. Moreover, $C^1$ consistency is considered based on the Taylor series to obtain accurate segregation flux gradient along the boundary. Our SPH model is validated with a shear box experiment. The model is then applied to investigate the segregation mechanism in bidisperse-sized granular flows in a rotating drum.

JFM classification

Granular media: Dry granular material Mass Transport: Coupled diffusion and flow Mathematical Foundations: Computational methods
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 977 , 25 December 2023 , A47
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adami, S., Hu, X.Y. & Adams, N.A. 2012 A generalized wall boundary condition for smoothed particle hydrodynamics. J. Comput. Phys. 231, 7057–7075.CrossRefGoogle Scholar
Bancroft, R. & Johnson, C.G. 2021 Drag, diffusion and segregation in inertial granular flows. J. Fluid Mech. 924, A3.CrossRefGoogle Scholar
Barker, T., Rauter, M., Maguire, E.S.F., Johnson, C.G. & Gray, J.M.N.T. 2021 Coupling rheology and segregation in granular flows. J. Fluid Mech. 909, A22.Google Scholar
Barker, T., Schaeffer, D.G., Bohorquez, P. & Gray, J.M.N.T. 2015 Well-posed and ill-posed behaviour of the $\mu (I)$-rheology for granular flow. J. Fluid Mech. 779, 794–818.Google Scholar
Bartelt, P. & McArdell, B.W. 2009 Granulometric investigations of snow avalanches. J. Glaciol. 55, 829–833.Google Scholar
de Borst, R. 1991 Simulation of strain localization: a reappraisal of the Cosserat continuum. Engng Comput. 8, 317–332.Google Scholar
de Borst, R. 1993 A generalisation of J$_2$-flow theory for polar continua. Comput. Meth. Appl. Mech. Engng 103, 347–362.Google Scholar
Brandao, R.J., Lima, R.M., Santos, R.L., Duarte, C.R. & Barrozo, M.A.S. 2020 Experimental study and DEM analysis of granular segregation in a rotating drum. Powder Technol. 364, 1–12.Google Scholar
Bridgwater, J. 1980 Self-diffusion coefficients in deforming powders. Powder Technol. 25, 129–131.Google Scholar
Bui, H.H., Fukagawa, R., Sako, K. & Ohno, S. 2008 Lagrangian meshfree particles method (SPH) for large deformation and failure flows of geomaterial using elastic-plastic soil constitutive model. Intl J. Numer. Anal. Meth. Geomech. 32, 1537–1570.Google Scholar
Colagrossi, A., Antuono, M. & Le Touzé, D. 2009 Theoretical considerations on the free-surface role in the smoothed-particle-hydrodynamics model. Phys. Rev. E 79, 056701.Google Scholar
Dehnen, W. & Hossam, A. 2012 Improving convergence in smoothed particle hydrodynamics simulations without pairing instability. Mon. Not. R. Astron. Soc. 425, 1068–1082.Google Scholar
Dolgunin, V.N. & Ukolov, A.A. 1995 Segregation modeling of particle rapid gravity flow. Powder Technol. 83, 95–103.CrossRefGoogle Scholar
Drahun, J.A. & Bridgwater, J. 1983 The mechanisms of free surface segregation. Powder Technol. 36, 39–53.Google Scholar
Dsouza, P.V. & Nott, P.R. 2020 A non-local constitutive model for slow granular flow that incorporates dilatancy. J. Fluid Mech. 888, R3.Google Scholar
Ehrichs, E.E., Jaeger, H.M., Karczmar, G.S., Knight, J.B., Kuperman, V.Y. & Nagel, S.R. 1995 Granular convection observed by magnetic resonance imaging. Science 267, 1632–1634.Google Scholar
Fan, Y., Schlick, C.P., Umbanhowar, P.B., Ottino, J.M. & Lueptow, R.M. 2014 Modelling size segregation of granular materials: the roles of segregation. J. Fluid Mech. 741, 252–279.Google Scholar
Fourtakas, G. & Rogers, B.D. 2016 Modelling multi-phase liquid-sediment scour and resuspension induced by rapid flows using smoothed particle hydrodynamics (SPH) accelerated with a graphics processing unit (GPU). Adv. Water Resour. 92, 186–199.Google Scholar
Fry, A.M., Umbanhowar, P.B., Ottino, J.M. & Lueptow, R.M. 2018 Effect of pressure on segregation in granular shear flows. Phys. Rev. E 97, 062906.CrossRefGoogle ScholarPubMed
Fry, A.M., Umbanhowar, P.B., Ottino, J.M. & Lueptow, R.M. 2019 Diffusion, mixing, and segregation in confined granular flows. AIChE J. 65, 875–881.Google Scholar
Gajjar, P. & Gray, J.M.N.T. 2014 Asymmetric flux models for particle-size segregation in granular avalanches. J. Fluid Mech. 757, 297–329.Google Scholar
Gajjar, P., Johnson, C.G., Carr, J., Chrispeels, K., Gray, J.M.N.T. & Withers, P.J. 2021 Size segregation of irregular granular materials captured by time-resolved 3D imaging. Sci. Rep. 11, 1–6.Google Scholar
Gilberg, D. & Steiner, K. 2020 Size segregation in compressible granular shear flows of binary particle systems. Granul. Matt. 22, 1–15.Google Scholar
Gingold, R.A. & Monaghan, J.J. 1977 Smoothed particle hydrodynamics: theory and application to non-spherical stars. Mon. Not. R. Astron. Soc. 181, 375–389.Google Scholar
Golick, L.A. & Daniels, K.E. 2009 Mixing and segregation rates in sheared granular materials. Phys. Rev. E 80, 042301.Google Scholar
Gray, J.M.N.T. 2018 Particle segregation in dense granular flows. Annu. Rev. Fluid Mech. 50, 407–433.Google Scholar
Gray, J.M.N.T. & Chugunov, V.A. 2006 Particle-size segregation and diffusive remixing in shallow granular avalanches. J. Fluid Mech. 569, 365–398.Google Scholar
Gray, J.M.N.T. & Thornton, A.R. 2005 A theory for particle size segregation in shallow granular free-surface flows. Proc. R. Soc. A: Math. Phys. Engng Sci. 461, 1447–1473.Google Scholar
Green, S. 2010 Particle simulation using CUDA. NVIDIA Whitepaper 6, 121–128.Google Scholar
Hajra, S.K. & Khakhar, D.V. 2002 Radial mixing of granular materials in a rotating cylinder: experimental determination of particle self-diffusivity. Phys. Fluids 17, 013101.Google Scholar
Harrison, S.M., Eyres, G.E., Cleary, P.W., Sinnott, M.D., Delahunty, C. & Lundin, L. 2014 Computational modeling of food oral breakdown using smoothed particle hydrodynamics. J. Texture Stud. 45, 97–109.Google Scholar
Hsiau, S.S. & Shieh, Y.M. 1999 Fluctuations and self-diffusion of sheared granular material flows. J. Rheol. 43, 1049–1066.Google Scholar
Huang, A.N., Kao, W.-C. & Kuo, H.-P. 2013 Numerical studies of particle segregation in a rotating drum based on Eulerian continuum approach. Adv. Powder Technol. 24, 364–372.Google Scholar
Iverson, R.M. 1997 The physics of debris flows. Rev. Geophys. 35, 245–296.Google Scholar
Jiang, M., Wu, P., Hu, L., Fu, H., Chen, B. & Wang, L. 2021 Experimental study on the size segregation of binary particles in a moving granular bed. Powder Technol. 388, 82–89.Google Scholar
Jop, P., Forterre, Y. & Pouliquen, O. 2006 A constitutive law for dense granular flows. Nature 441, 727–730.Google Scholar
Khakhar, D.V., McCarthy, J.J. & Ottino, J.M. 1997 Radial segregation of granular mixtures in rotating cylinders. Phys. Fluids 9, 3600–3614.Google Scholar
Liu, G.-R. & Liu, M.B. 2003 Smoothed Particle Hydrodynamics: a Meshfree Particle Method. World Scientific.Google Scholar
Liu, M.B., Liu, G.L., Zong, Z. & Lam, K.Y. 2003 Computer simulation of high explosive explosion using smoothed particle hydrodynamics methodology. Comput. Fluids 32, 305–322.CrossRefGoogle Scholar
Liu, M.B. & Liu, G.R. 2010 Smoothed particle hydrodynamics (SPH): an overview and recent developments. Arch. Comput. Meth. Engng 17, 25–76.Google Scholar
Liu, M.B. & Liu, G.R. 2011 Restoring particle consistency in smoothed particle hydrodynamics. Appl. Numer. Maths 56, 19–36.CrossRefGoogle Scholar
Liu, Y., Gonzalez, M. & Wassgren, C. 2018 Modeling granular material blending in a rotating drum using a finite element method and advection–diffusion equation multiscale model. AIChE J. 24, 364–372.Google Scholar
Lucy, L.B. 1977 A numerical approach to the testing of the fission hypothesis. Astron. J. 82, 1013–1024.Google Scholar
Lyu, H.G. & Sun, P.N. 2022 Further enhancement of the particle shifting technique: towards better volume conservation and particle distribution in SPH simulations of violent free-surface flows. Appl. Math. Model. 101, 214–238.Google Scholar
Macia, F., Antuono, M., González, L.M. & Colagrossi, A. 2011 Theoretical analysis of the no-slip boundary condition enforcement in SPH methods. Prog. Theor. Phys. 125, 1091–1121.Google Scholar
Marrone, S., Colagrossi, A., Le Touzé, D. & Graziani, G. 2010 Fast free-surface detection and level-set function definition in SPH solvers. J. Comput. Phys. 229, 3652–3663.Google Scholar
May, L.B.H., Golick, L.A., Phillips, K.C., Shearer, M. & Daniels, K.E. 2010 Shear-driven size segregation of granular materials: modeling and experiment. Phys. Rev. E 550, 1–25.Google Scholar
Monaghan, J.J. 1992 Smoothed particle hydrodynamics. Annu. Rev. Astron. Astrophys. 30, 543–574.Google Scholar
Monaghan, J.J. 2012 Smoothed particle hydrodynamics and its diverse applications. Annu. Rev. Fluid Mech. 44, 323–346.Google Scholar
Monaghan, J.J. & Lattanzio, J.C. 1985 A refined particle method for astrophysical problems. Astron. Astrophys. 149, 135–143.Google Scholar
Morris, J.P. 1996 A study of the stability properties of smooth particle hydrodynamics. Publ. Astron. Soc. Aust. 13, 97–102.Google Scholar
Mullin, T. 2000 Coarsening of self-organized clusters in binary mixtures of particles. Phys. Rev. Lett. 84, 4741.CrossRefGoogle ScholarPubMed
Nguyen, C.T., Nguyen, C.T., Bui, H.H., Nguyen, G.D. & Fukagawa, R. 2000 A new SPH-based approach to simulation of granular flows using viscous damping and stress regularisation. Landslides 14, 69–81.Google Scholar
Ottino, J.M. & Khakhar, D.V. 2000 Mixing and segregation of granular materials. Annu. Rev. Fluid Mech. 32, 55–91.Google Scholar
Pan, W., Kim, K., Perego, M., Tartakovsky, A.M. & Parks, M.L. 2017 Modeling electrokinetic flows by consistent implicit incompressible smoothed particle hydrodynamics. J. Comput. Phys. 334, 125–144.Google Scholar
Peng, C., Wang, S., Wu, W., Yu, H., Wang, C. & Chen, J. 2019 An open-source GPU-accelerated SPH solver for geotechnical modeling. Acta Geotech. 14, 1269–1287.Google Scholar
Peng, C., Xu, G., Wu, W., Yu, H.-S. & Wang, C. 2017 Multiphase SPH modeling of free surface flow in porous media with variable porosity. Comput. Geotech. 81, 239–248.Google Scholar
Rosato, A., Strandburg, K.J., Prinz, F. & Swendsen, R.H. 1987 Why the Brazil nuts are on top: size segregation of particulate matter by shaking. Phys. Rev. Lett. 58, 1038.Google Scholar
Ryan, E.M., Tartakovsky, A.M. & Amon, C. 2010 A novel method for modeling Neumann and Robin boundary conditions in smoothed particle hydrodynamics. Comput. Phys. Commun. 181, 2008–2023.Google Scholar
Savage, S.B. & Dai, R. 1993 Studies of granular shear flows wall slip velocities, ‘layering’ and self-diffusion. Mech. Mater. 16, 225–238.Google Scholar
Savage, S.B. & Lun, C.K.K. 1988 Particle size segregation in inclined chute flow of dry cohesionless granular solids. J. Fluid Mech. 189, 311–335.CrossRefGoogle Scholar
Schlick, C.P., Fan, Y., Isner, A.B., Umbanhowar, P.B., Ottino, J.M. & Lueptow, R.M. 2015 a Modeling segregation of bidisperse granular materials using physical control parameters in the quasi-2D bounded heap. J. Fluid Mech. 765, 632–652.Google Scholar
Schlick, C.P., Fan, Y., Umbanhowar, P.B., Ottino, J.M. & Lueptow, R.M. 2015 b Granular segregation in circular tumblers: theoretical model and scaling laws. J. Fluid Mech. 765, 632–652.Google Scholar
Schröter, M., Ulrich, S., Kreft, J., Swift, J.B. & Swinney, H.L. 2006 Mechanisms in the size segregation of a binary granular mixture. Phys. Rev. E 74, 011307.Google Scholar
Thornton, A., Weinhart, T., Luding, S. & Bokhove, O. 2012 Modeling of particle size segregation: calibration using the discrete particle method. Intl J. Mod. Phys. C 23, 1240014.Google Scholar
Thornton, A.R., Gray, J.M.N.T. & Hogg, A.J. 2006 A three-phase mixture theory for particle size segregation in shallow granular free-surface flows. J. Fluid Mech. 550, 1–25.Google Scholar
Trewhela, T., Ancey, C. & Gray, J.M.N.T. 2021 a An experimental scaling law for particle-size segregation in dense granular flows. J. Fluid Mech. 916, A55.CrossRefGoogle Scholar
Trewhela, T., Gray, J.M.N.T. & Ancey, C. 2021 b Large particle segregation in two-dimensional sheared granular flows. Phys. Rev. Fluids 6, 054302.Google Scholar
Tunuguntla, D.R., Weinhart, T. & Thornton, A.R. 2017 Comparing and contrasting size-based particle segregation models. Comput. Part. Mech. 4, 387–405.Google Scholar
Vacondio, R., Altomare, C., De Leffe, M., Hu, X., Le Touzé, D., Lind, S., Marongiu, J.C., Marrone, S., Rogers, B.D. & Souto-Iglesias, A. 2021 Grand challenges for smoothed particle hydrodynamics numerical schemes. Comput. Part. Mech. 8, 575–588.Google Scholar
Valizadeh, A. & Monaghan, J.J. 2015 A study of solid wall models for weakly compressible SPH. J. Comput. Phys. 300, 5–19.CrossRefGoogle Scholar
Van der Vaart, K., Gajjar, P., Épely-Chauvin, G., Andreini, N., Gray, J.M.N.T. & Ancey, C. 2015 Underlying asymmetry within particle size segregation. Phys. Rev. Lett. 114, 238001.Google Scholar
Viccione, G., Bovolin, V. & Carratelli, E.P. 2008 Defining and optimizing algorithms for neighbouring particle identification in SPH fluid simulations. Intl J. Numer. Meth. Fluids 58, 625–638.Google Scholar
Wang, J., Hu, W., Zhang, X. & Pan, W. 2019 Modeling heat transfer subject to inhomogeneous Neumann boundary conditions by smoothed particle hydrodynamics and peridynamics. Intl J. Heat Mass Transfer 139, 948–962.Google Scholar
Wendland, H. 1995 Piecewise polynomial, positive definite and compactly supported radial functions of minimal degree. Adv. Comput. Math. 4, 389–396.Google Scholar
Wiederseiner, S., Andreini, N., Épely-Chauvin, G., Moser, G., Monnereau, M., Gray, J.M.N.T. & Ancey, C. 2011 Experimental investigation into segregating granular flows down chutes. Phys. Fluids 23, 013301.Google Scholar
Xu, Z., Yoshinaga, S., Tsunazawa, Y. & Tokoro, C. 2021 Numerical investigation of segregation behavior of multi-sized particles during pharmaceutical mini-tablet die filling. J. Drug Deliv. Sci. Technol. 61, 102301.Google Scholar
Yang, L.Y.M., Zheng, Q.J., Bai, L. & Yu, A.B. 2021 Continuum modelling of granular segregation by coupling flow rheology and transport equation. Powder Technol. 378, 371–387.Google Scholar
Yu, H.S. 2007 Plasticity and Geotechnics. Springer Science & Business Media.Google Scholar
Zhan, L., Peng, C., Zhang, B. & Wu, W. 2019 Three-dimensional modeling of granular flow impact on rigid and deformable structures. Comput. Geotech. 112, 257–271.Google Scholar
Zhang, C., Wang, J., Rezavand, M., Wu, D. & Hu, X. 2021 An integrative smoothed particle hydrodynamics method for modeling cardiac function. Comput. Meth. Appl. Mech. Engng 381, 113847.Google Scholar
Zhu, C., Peng, C. & Wu, W. 2021 Applications of micropolar SPH in geomechanics. Acta Geotech. 16, 2355–2369.Google Scholar
Zhu, C., Peng, C. & Wu, W. 2022 a Lagrangian meshfree particle method (SPH) based simulation for granular flow in a rotating drum with regularized $\mu (I)$ elastoplastic model. Powder Technol. 408, 117699.Google Scholar
Zhu, C., Peng, C., Wu, W. & Wang, C. 2022 b A multi-layer SPH method for generic water–soil dynamic coupling problems. Part I. Revisit, theory, and validation. Comput. Meth. Appl. Mech. Engng 396, 115106.Google Scholar