Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Baratian, Davood
Ruiz-Gutiérrez, Élfego
Mugele, Frieder
and
Ledesma-Aguilar, Rodrigo
2019.
Slippery when wet: mobility regimes of confined drops in electrowetting.
Soft Matter,
Vol. 15,
Issue. 35,
p.
7063.
Yang, Yanjie
Chen, Xiaoqian
and
Huang, Yiyong
2019.
Spreading Dynamics of Droplet Impact on a Wedge-Patterned Biphilic Surface.
Applied Sciences,
Vol. 9,
Issue. 11,
p.
2214.
McHale, Glen
Orme, Bethany V.
Wells, Gary G.
and
Ledesma-Aguilar, Rodrigo
2019.
Apparent Contact Angles on Lubricant-Impregnated Surfaces/SLIPS: From Superhydrophobicity to Electrowetting.
Langmuir,
Vol. 35,
Issue. 11,
p.
4197.
Won, Dong‐Joon
Huh, Myoung
Lee, Sangmin
Park, Usung
Yoo, Dongwoo
and
Kim, Joonwon
2020.
Capacitive‐Type Two‐Axis Accelerometer with Liquid‐Type Proof Mass.
Advanced Electronic Materials,
Vol. 6,
Issue. 6,
McHale, Glen
Ledesma-Aguilar, Rodrigo
and
Wells, Gary George
2020.
Interfacial Strategies for Smart Slippery Surfaces.
Journal of Bionic Engineering,
Vol. 17,
Issue. 4,
p.
633.
Ruiz-Gutiérrez, Élfego
and
Ledesma-Aguilar, Rodrigo
2020.
Lattice-Boltzmann simulations of the dynamics of liquid barrels.
Journal of Physics: Condensed Matter,
Vol. 32,
Issue. 21,
p.
214007.
Butler, Matthew D
and
Vella, Dominic
2020.
Detachment in capillary adhesion: the relative roles of tilting and separation.
IMA Journal of Applied Mathematics,
Vol. 85,
Issue. 5,
p.
673.
Torres, Logan J.
and
Weislogel, Mark M.
2021.
The ejection of large non-oscillating droplets from a hydrophobic wedge in microgravity.
npj Microgravity,
Vol. 7,
Issue. 1,
Zhuang, Kai
Lu, Yao
Wang, Xiaolei
and
Yang, Xiaolong
2021.
Architecture-Driven Fast Droplet Transport without Mass Loss.
Langmuir,
Vol. 37,
Issue. 43,
p.
12519.
Fournier, Clementine
Lee, Carmen L.
Schulman, Rafael D.
Raphaël, Élie
and
Dalnoki-Veress, Kari
2021.
Droplet migration on conical fibers.
The European Physical Journal E,
Vol. 44,
Issue. 2,
Animasaun, Isaac Lare
Al-Mdallal, Qasem M.
Khan, Umair
and
Alshomrani, Ali Saleh
2022.
Unsteady Water-Based Ternary Hybrid Nanofluids on Wedges by Bioconvection and Wall Stretching Velocity: Thermal Analysis and Scrutinization of Small and Larger Magnitudes of the Thermal Conductivity of Nanoparticles.
Mathematics,
Vol. 10,
Issue. 22,
p.
4309.
Yang, Xiaolong
Li, Yimin
Zheng, Huanxi
and
Lu, Yao
2022.
Saturated Surface Charging on Micro/Nanoporous Polytetrafluoroethylene for Droplet Manipulation.
ACS Applied Nano Materials,
Vol. 5,
Issue. 3,
p.
3342.
Wu, Yanchen
Wang, Fei
Huang, Weidong
Selzer, Michael
and
Nestler, Britta
2022.
Capillary adsorption of droplets into a funnel-like structure.
Physical Review Fluids,
Vol. 7,
Issue. 5,
Zhang, Yixin
and
Zhao, Chengxi
2024.
Effects of dynamic wetting and liquid–solid slip on self-propelled nanodrops in tapered nanochannels.
Physics of Fluids,
Vol. 36,
Issue. 7,
Boudina, Mouad
and
Elfring, Gwynn J.
2024.
Equilibrium States of a Liquid Bridge between Flexible Sheets.
Langmuir,
Vol. 40,
Issue. 44,
p.
23243.
Di Novo, Nicolò Giuseppe
Bagolini, Alvise
and
Pugno, Nicola Maria
2024.
Single Condensation Droplet Self-Ejection from Divergent Structures with Uniform Wettability.
ACS Nano,
Vol. 18,
Issue. 12,
p.
8626.
Wu, Yanchen
Wang, Fei
Zheng, Sai
and
Nestler, Britta
2024.
Evolution dynamics of thin liquid structures investigated using a phase-field model.
Soft Matter,
Vol. 20,
Issue. 7,
p.
1523.
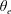 $\unicode[STIX]{x1D703}_{e}$, confined in a solid wedge geometry of opening angle
$\unicode[STIX]{x1D703}_{e}$, confined in a solid wedge geometry of opening angle  $\unicode[STIX]{x1D6FD}$. We focus on a mostly non-wetting regime, given by the condition
$\unicode[STIX]{x1D6FD}$. We focus on a mostly non-wetting regime, given by the condition 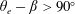 $\unicode[STIX]{x1D703}_{e}-\unicode[STIX]{x1D6FD}>90^{\circ }$, where the droplet forms a liquid barrel – a closed shape of positive mean curvature. Using a quasi-equilibrium assumption for the shape of the liquid–gas interface, we compute the changes to the surface energy and pressure distribution of the liquid upon a translation along the symmetry plane of the wedge. Our model is in good agreement with numerical calculations of the surface energy minimisation of static droplets deformed by gravity. Beyond the statics, we put forward a Lagrangian description of the droplet dynamics. We focus on the overdamped limit, where the driving capillary force is balanced by the frictional forces arising from the bulk hydrodynamics, the corner flow near the contact lines and the contact-line friction. Our results provide a theoretical framework to describe the motion of partially wetting liquids in confinement, and can be used to gain further understanding on the relative importance of dissipative processes that span from microscopic to macroscopic length scales.
$\unicode[STIX]{x1D703}_{e}-\unicode[STIX]{x1D6FD}>90^{\circ }$, where the droplet forms a liquid barrel – a closed shape of positive mean curvature. Using a quasi-equilibrium assumption for the shape of the liquid–gas interface, we compute the changes to the surface energy and pressure distribution of the liquid upon a translation along the symmetry plane of the wedge. Our model is in good agreement with numerical calculations of the surface energy minimisation of static droplets deformed by gravity. Beyond the statics, we put forward a Lagrangian description of the droplet dynamics. We focus on the overdamped limit, where the driving capillary force is balanced by the frictional forces arising from the bulk hydrodynamics, the corner flow near the contact lines and the contact-line friction. Our results provide a theoretical framework to describe the motion of partially wetting liquids in confinement, and can be used to gain further understanding on the relative importance of dissipative processes that span from microscopic to macroscopic length scales.


