Article contents
Stratified Ekman layers evolving under a finite-time stabilizing buoyancy flux
Published online by Cambridge University Press: 12 February 2018
Abstract
Stratified flow in nocturnal boundary layers is studied using direct numerical simulation (DNS) of the Ekman layer, a model problem that is useful to understand atmospheric boundary-layer (ABL) turbulence. A stabilizing buoyancy flux is applied for a finite time to a neutral Ekman layer. Based on previous studies and the simulations conducted here, the choice of 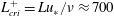 $L_{\mathit{cri}}^{+}=Lu_{\ast }/\unicode[STIX]{x1D708}\approx 700$ (
$L_{\mathit{cri}}^{+}=Lu_{\ast }/\unicode[STIX]{x1D708}\approx 700$ ( 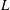 $L$ is the Obukhov length scale and
$L$ is the Obukhov length scale and 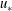 $u_{\ast }$ is the friction velocity) provides a cooling flux that is sufficiently strong to cause the initial collapse of turbulence. The turbulent kinetic energy decays over a time scale of
$u_{\ast }$ is the friction velocity) provides a cooling flux that is sufficiently strong to cause the initial collapse of turbulence. The turbulent kinetic energy decays over a time scale of 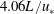 $4.06L/u_{\ast }$ during the collapse. The simulations suggest that imposing
$4.06L/u_{\ast }$ during the collapse. The simulations suggest that imposing 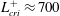 $L_{\mathit{cri}}^{+}\approx 700$ on the neutral Ekman layer results in turbulence collapse during the initial transient, independent of Reynolds number,
$L_{\mathit{cri}}^{+}\approx 700$ on the neutral Ekman layer results in turbulence collapse during the initial transient, independent of Reynolds number, 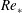 $Re_{\ast }$ . However, the long-time state of the flow, i.e. turbulent with spatial intermittency or non-turbulent, is found to depend on the initial value of
$Re_{\ast }$ . However, the long-time state of the flow, i.e. turbulent with spatial intermittency or non-turbulent, is found to depend on the initial value of  $Re_{\ast }$ since the cooling flux and resultant stratification increase with
$Re_{\ast }$ since the cooling flux and resultant stratification increase with  $Re_{\ast }$ for a given
$Re_{\ast }$ for a given 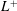 $L^{+}$ . The lower-
$L^{+}$ . The lower- 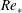 $Re_{\ast }$ cases have sustained turbulence with shear and stratification profiles that evolve in a manner such that the gradient Richardson number,
$Re_{\ast }$ cases have sustained turbulence with shear and stratification profiles that evolve in a manner such that the gradient Richardson number, 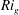 $Ri_{g}$ , in the near-surface layer, including the low-level jet, remains subcritical. The highest
$Ri_{g}$ , in the near-surface layer, including the low-level jet, remains subcritical. The highest 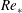 $Re_{\ast }$ case has supercritical
$Re_{\ast }$ case has supercritical 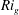 $Ri_{g}$ in the low-level jet and turbulence does not recover. A theoretical discussion is performed to infer that the bulk Richardson number,
$Ri_{g}$ in the low-level jet and turbulence does not recover. A theoretical discussion is performed to infer that the bulk Richardson number, 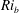 $Ri_{b}$ , is more suitable than
$Ri_{b}$ , is more suitable than 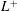 $L^{+}$ to determine the fate of stratified Ekman layers at late time. DNS results support the implications of
$L^{+}$ to determine the fate of stratified Ekman layers at late time. DNS results support the implications of 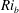 $Ri_{b}$ for the effect of initial
$Ri_{b}$ for the effect of initial 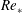 $Re_{\ast }$ and
$Re_{\ast }$ and 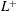 $L^{+}$ on the flow.
$L^{+}$ on the flow.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 7
- Cited by

