Article contents
Structural organization of large and very large scales in turbulent pipe flow simulation
Published online by Cambridge University Press: 27 February 2013
Abstract
The physical structures of velocity are examined from a recent direct numerical simulation of fully developed incompressible turbulent pipe flow (Wu, Baltzer & Adrian, J. Fluid Mech., vol. 698, 2012, pp. 235–281) at a Reynolds number of 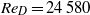 ${\mathit{Re}}_{D} = 24\hspace{0.167em} 580$ (based on bulk velocity) and a Kármán number of
${\mathit{Re}}_{D} = 24\hspace{0.167em} 580$ (based on bulk velocity) and a Kármán number of  ${R}^{+ } = 685$. In that work, the periodic domain length of
${R}^{+ } = 685$. In that work, the periodic domain length of 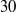 $30$ pipe radii
$30$ pipe radii 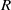 $R$ was found to be sufficient to examine long motions of negative streamwise velocity fluctuation that are commonly observed in wall-bounded turbulent flows and correspond to the large fractions of energy present at very long streamwise wavelengths (
$R$ was found to be sufficient to examine long motions of negative streamwise velocity fluctuation that are commonly observed in wall-bounded turbulent flows and correspond to the large fractions of energy present at very long streamwise wavelengths (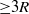 ${\geq }3R$). In this paper we study how long motions are composed of smaller motions. We characterize the spatial arrangements of very large-scale motions (VLSMs) extending through the logarithmic layer and above, and we find that they possess dominant helix angles (azimuthal inclinations relative to streamwise) that are revealed by two- and three-dimensional two-point spatial correlations of velocity. The correlations also reveal that the shorter, large-scale motions (LSMs) that concatenate to comprise the VLSMs are themselves more streamwise aligned. We show that the largest VLSMs possess a form similar to roll cells centred above the logarithmic layer and that they appear to play an important role in organizing the flow, while themselves contributing only a minor fraction of the flow turbulent kinetic energy. The roll cell motions play an important role with the smaller scales of motion that are necessary to create the strong streamwise streaks of low-velocity fluctuation that characterize the flow.
${\geq }3R$). In this paper we study how long motions are composed of smaller motions. We characterize the spatial arrangements of very large-scale motions (VLSMs) extending through the logarithmic layer and above, and we find that they possess dominant helix angles (azimuthal inclinations relative to streamwise) that are revealed by two- and three-dimensional two-point spatial correlations of velocity. The correlations also reveal that the shorter, large-scale motions (LSMs) that concatenate to comprise the VLSMs are themselves more streamwise aligned. We show that the largest VLSMs possess a form similar to roll cells centred above the logarithmic layer and that they appear to play an important role in organizing the flow, while themselves contributing only a minor fraction of the flow turbulent kinetic energy. The roll cell motions play an important role with the smaller scales of motion that are necessary to create the strong streamwise streaks of low-velocity fluctuation that characterize the flow.
JFM classification
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 96
- Cited by


