Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Balasuriya, Sanjeeva
2017.
Transport between Two Fluids across Their Mutual Flow Interface: The Streakline Approach.
SIAM Journal on Applied Dynamical Systems,
Vol. 16,
Issue. 2,
p.
1015.
Li Gao-Hua
and
Wang Fu-Xin
2018.
Evolution characteristic analysis of double-helical vortex wake of high Reynolds number flow.
Acta Physica Sinica,
Vol. 67,
Issue. 5,
p.
054701.
Singh, Surabhi
Keeler, Jason
and
Ukeiley, Lawrence S.
2018.
Application of POD to Pulse Burst PIV Data of Flow Over an Open Cavity.
Abbasi, Farshid
and
Mohammadpour Velni, Javad
2018.
Robust non‐linear control design for systems governed by Burgers' equation subjected to parameter variation.
IET Control Theory & Applications,
Vol. 12,
Issue. 5,
p.
582.
Tinney, Charles E.
and
Valdez, John
2020.
Thrust and Acoustic Performance of Small-Scale, Coaxial, Corotating Rotors in Hover.
AIAA Journal,
Vol. 58,
Issue. 4,
p.
1657.
Dghim, Marouen
Ferchichi, Mohsen
and
Fellouah, Hachimi
2020.
On the effect of active flow control on the meandering of a wing-tip vortex.
Journal of Fluid Mechanics,
Vol. 896,
Issue. ,
Singh, Surabhi
and
Ukeiley, Lawrence
2020.
Proper Orthogonal Decomposition of High-Speed Particle Image Velocimetry in an Open Cavity.
AIAA Journal,
Vol. 58,
Issue. 7,
p.
2975.
Tinney, Charles E.
Shipman, Jeremy
and
Panickar, Praveen
2020.
Proper-Orthogonal-Decomposition-Based Reduced-Order Models for Characterizing Ship Airwake Interactions.
AIAA Journal,
Vol. 58,
Issue. 2,
p.
633.
Cuppoletti, Daniel R.
and
Riley, Troy
2022.
Time-Resolved Flow Field and Acoustic Measurements of a Ducted and Unducted Rotor.
Sorensen, Peter N.
and
Cuppoletti, Daniel R.
2022.
Source Noise Mechanisms of Co-Rotating and Counter Rotating Rotors in Hover for Air Mobility Applications.
Valdez, John A.
and
Tinney, Charles E.
2022.
The unsteady wake produced by a coaxial co-rotating rotor in hover.
Valdez, John A.
and
Tinney, Charles E.
2022.
Wake of a Coaxial Corotating Rotor in Hover.
AIAA Journal,
Vol. 60,
Issue. 8,
p.
4829.
Ceglia, G.
Astarita, T.
and
De Gregorio, F.
2023.
An experimental investigation of rotor wakes by means of time-resolved PIV measurements.
Aerospace Science and Technology,
Vol. 141,
Issue. ,
p.
108556.
Tinney, Charles E
Zhao-Dubuc, Yingjun
and
Valdez, John
2023.
The space-time structure of sound produced by stacked rotors in hover using Vold-Kalman filters and proper orthogonal decomposition.
International Journal of Aeroacoustics,
Vol. 22,
Issue. 5-6,
p.
576.
Tinney, Charles E.
Valdez, John A.
and
Zhao, Yingjun
2023.
A Moving Source Model for Rotor Broadband Noise.
Cuppoletti, Daniel R.
and
Riley, Troy
2024.
Time-Resolved Flowfield and Acoustic Measurements of a Ducted and Unducted Rotor.
AIAA Journal,
p.
1.
Zaccara, Mirko
Paolillo, Gerardo
Cafiero, Gioacchino
Astarita, Tommaso
Iuso, Gaetano
Cardone, Gennaro
and
Greco, Carlo Salvatore
2024.
Near field evolution of wingtip vortices under synthetic-jet based control.
Aerospace Science and Technology,
Vol. 148,
Issue. ,
p.
109068.
Tinney, Charles E.
Valdez, John
and
Zhao-Dubuc, Yingjun
2024.
Distilling the acoustics of stacked rotors using conventional POD with Vold-Kalman filters..
Ben-Gida, Hadar
Baba, Satoshi
Stalnov, Oksana
Moreau, Stephane
and
Lavoie, Philippe
2024.
Experimental Characterization of the Side-Edge Triple-Vortex System on a Supercritical Wing Model.
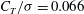 $C_{T}/{\it\sigma}=0.066$. In-plane components of the velocity field (normal to the axis of the vortex filament) are captured by way of two-dimensional particle image velocimetry with corrections for vortex wander being performed using the
$C_{T}/{\it\sigma}=0.066$. In-plane components of the velocity field (normal to the axis of the vortex filament) are captured by way of two-dimensional particle image velocimetry with corrections for vortex wander being performed using the 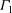 ${\it\Gamma}_{1}$ method. The first POD mode alone is found to encompass nearly 75 % of the energy for all vortex ages studied and is determined using a grid of sufficient resolution to avoid numerical integration errors in the decomposition. The findings reveal an equal balance between the axisymmetric and helical modes during vortex roll-up, which immediately transitions to helical mode dominance at all other vortex ages. This helical mode is one of the modes of the elliptic instability. The spatial eigenfunctions of the first few Fourier-azimuthal modes associated with the most energetic POD mode is shown to be sensitive to the choice of the wander correction technique used. Higher Fourier-azimuthal modes are observed in the outer portions of the vortex and appeared not to be affected by the choice of the wander correction technique used.
${\it\Gamma}_{1}$ method. The first POD mode alone is found to encompass nearly 75 % of the energy for all vortex ages studied and is determined using a grid of sufficient resolution to avoid numerical integration errors in the decomposition. The findings reveal an equal balance between the axisymmetric and helical modes during vortex roll-up, which immediately transitions to helical mode dominance at all other vortex ages. This helical mode is one of the modes of the elliptic instability. The spatial eigenfunctions of the first few Fourier-azimuthal modes associated with the most energetic POD mode is shown to be sensitive to the choice of the wander correction technique used. Higher Fourier-azimuthal modes are observed in the outer portions of the vortex and appeared not to be affected by the choice of the wander correction technique used.