Article contents
Surfactant effect on path instability of a rising bubble
Published online by Cambridge University Press: 04 December 2013
Abstract
We report results from the first systematic experiments for investigating surfactant effects on path instability of an air bubble rising in quiescent water. The addition of surfactant to a gas–water system causes a non-uniform distribution of surfactant concentration along the bubble surface, resulting in variations in the gas–water boundary condition from zero shear stress to non-zero shear stress due to the Marangoni effect. This leads to retarded surface velocity and ends up with immobilization of the bubble surface with increasing surfactant concentration, where the drag corresponds to that of a solid sphere of the same size. Using two high-speed cameras and vertical traverse systems, we measure three-dimensional trajectories, velocities and aspect ratios of a millimetre-sized bubble simultaneously for 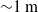 ${\sim }1~\mathrm{m} $. Experimental parameters are the diameter of the bubble and the surfactant concentration of 1-Pentanol or Triton X-100. We explore the surfactant effect on the drag and lift forces acting on the bubble in helical motion. While the drag force monotonically increases with the surfactant concentration as expected, the lift force shows a non-monotonic behaviour. Nevertheless, the direction of the lift force in a reference frame that rotates with the bubble along its trajectory is kept almost constant. We also observe the transient trajectory starting from helical motion to zigzag, which has never been reported in the case of purified water. The instantaneous amplitude and frequency of the transient motion agree with those of the motion regarded as steady. Finally the bubble motions are categorized as straight/helical/zigzag and experimentally examined in the field of two dimensionless numbers: Reynolds number
${\sim }1~\mathrm{m} $. Experimental parameters are the diameter of the bubble and the surfactant concentration of 1-Pentanol or Triton X-100. We explore the surfactant effect on the drag and lift forces acting on the bubble in helical motion. While the drag force monotonically increases with the surfactant concentration as expected, the lift force shows a non-monotonic behaviour. Nevertheless, the direction of the lift force in a reference frame that rotates with the bubble along its trajectory is kept almost constant. We also observe the transient trajectory starting from helical motion to zigzag, which has never been reported in the case of purified water. The instantaneous amplitude and frequency of the transient motion agree with those of the motion regarded as steady. Finally the bubble motions are categorized as straight/helical/zigzag and experimentally examined in the field of two dimensionless numbers: Reynolds number  $\mathit{Re}\in $ [300 900] and the normalized drag coefficient
$\mathit{Re}\in $ [300 900] and the normalized drag coefficient 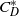 ${ C}_{D}^{\ast } $ which represents the slip condition. Remarkably it is found that the motions of a bubble with the intermediate slip conditions between free-slip and no-slip are helical for a broad range of
${ C}_{D}^{\ast } $ which represents the slip condition. Remarkably it is found that the motions of a bubble with the intermediate slip conditions between free-slip and no-slip are helical for a broad range of 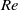 $\mathit{Re}$.
$\mathit{Re}$.
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 67
- Cited by


