Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-29T06:56:01.988Z Has data issue: false hasContentIssue false
  • English
  • Français

A tale of two airfoils: resolvent-based modelling of an oscillator versus an amplifier from an experimental mean

Published online by Cambridge University Press:  24 October 2019

Sean Symon Open the ORCID record for Sean Symon [Opens in a new window] ,
Denis Sipp Open the ORCID record for Denis Sipp [Opens in a new window]  and
Beverley J. McKeon Open the ORCID record for Beverley J. McKeon [Opens in a new window]
Sean Symon*
Affiliation:
Department of Mechanical Engineering, University of Melbourne, VIC 3010, Australia Graduate Aerospace Laboratories, California Institute of Technology, Pasadena, CA 91125, USA
Denis Sipp
Affiliation:
ONERA DAAA, 8 rue des Vertugadins, 92190 Meudon, France
Beverley J. McKeon
Affiliation:
Graduate Aerospace Laboratories, California Institute of Technology, Pasadena, CA 91125, USA
*
†Email address for correspondence: ssymon@unimelb.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

The flows around a NACA 0018 airfoil at a chord-based Reynolds number of 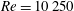$Re=10\,250$ and angles of attack of 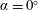$\unicode[STIX]{x1D6FC}=0^{\circ }$ and 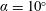$\unicode[STIX]{x1D6FC}=10^{\circ }$ are modelled using resolvent analysis and limited experimental measurements obtained from particle image velocimetry. The experimental mean velocity fields are data assimilated so that they are solutions of the incompressible Reynolds-averaged Navier–Stokes equations forced by Reynolds stress terms which are derived from experimental data. Resolvent analysis of the data-assimilated mean velocity fields reveals low-rank behaviour only in the vicinity of the shedding frequency for 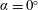$\unicode[STIX]{x1D6FC}=0^{\circ }$ and none of its harmonics. The resolvent operator for the 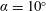$\unicode[STIX]{x1D6FC}=10^{\circ }$ case, on the other hand, identifies two linear mechanisms whose frequencies are a close match with those identified by spectral proper orthogonal decomposition. It is also shown that the second linear mechanism, corresponding to the Kelvin–Helmholtz instability in the shear layer, cannot be identified just by considering the time-averaged experimental measurements as an input for resolvent analysis due to missing data near the leading edge. For both cases, resolvent modes resemble those from spectral proper orthogonal decomposition when the resolvent operator is low rank. The $\unicode[STIX]{x1D6FC}=0^{\circ }$ case is classified as an oscillator and its harmonics, where the resolvent operator is not low rank, are modelled using parasitic modes as opposed to classical resolvent modes which are the most amplified. The $\unicode[STIX]{x1D6FC}=10^{\circ }$ case behaves more like an amplifier and its nonlinear forcing is far less structured. The two cases suggest that resolvent-based modelling can be achieved for more complex flows with limited experimental measurements.

JFM classification

Wakes/Jets: Separated flows Wakes/Jets: Vortex streets Wakes/Jets: Wakes
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 881 , 25 December 2019 , pp. 51 - 83
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Amestoy, P. R., Duff, I. S., L’Excellent, J.-Y. & Koster, J. 2001 A fully asynchronous multifrontal solver using distributed dynamic shedding. SIAM J. Matrix Anal. Applics. 23 (1), 15–41.Google Scholar
Beneddine, S., Sipp, D., Arnault, A., Dandois, J. & Lesshafft, L. 2016 Conditions for validity of mean flow stability analysis. J. Fluid Mech. 798, 485–504.Google Scholar
Beneddine, S., Yegavian, R., Sipp, D. & Leclaire, B. 2017 Unsteady flow dynamics reconstruction from mean flow and point sensors: an experimental study. J. Fluid Mech. 824, 174–201.Google Scholar
Dergham, G., Sipp, D. & Robinet, J.-Ch. 2013 Stochastic dynamics and model reduction of amplifier flows: the backward facing step flow. J. Fluid Mech. 719, 406–430.Google Scholar
Dunne, R.2016 Dynamic stall on vertical axis wind turbine blades. PhD thesis, California Institute of Technology, Pasadena, CA.Google Scholar
Foures, D. P. G., Dovetta, N., Sipp, D. & Schmid, P. J. 2014 A data-assimilation method for Reynolds-averaged Navier–Stokes-driven mean flow reconstruction. J. Fluid Mech. 759, 404–431.Google Scholar
Gómez, F., Blackburn, H. M., Rudman, M., McKeon, B. J., Luhar, M., Moarref, R. & Sharma, A. S. 2014 On the origin of frequency sparsity in direct numerical simulations of turbulent pipe flow. Phys. Fluids 26, 101703.Google Scholar
Gómez, F., Blackburn, H. M., Rudman, M., Sharma, A. S. & McKeon, B. J. 2016a A reduced-order model of three-dimensional unsteady flow in a cavity based on the resolvent operator. J. Fluid Mech. 798, R2.Google Scholar
Gómez, F., Sharma, A. S. & Blackburn, H. M. 2016b Estimation of unsteady aerodynamic forces using pointwise velocity data. J. Fluid Mech. 804, R4.Google Scholar
Hayase, T. 2015 Numerical simulation of real-world flows. Fluid Dyn. Res. 47, 051201.Google Scholar
He, C., Liu, Y., Gan, L. & Lesshafft, L. 2019 Data assimilation and resolvent analysis of turbulent flow behind a wall-proximity rib. Phys. Fluids 31, 025118.Google Scholar
Hecht, F. 2012 New development in FreeFem++. J. Numer. Math. 20, 251–265.Google Scholar
Huerre, P. & Rossi, M. 1998 Hydrodynamics and Nonlinear Instabilities, pp. 81–294. Cambridge University Press.Google Scholar
Illingworth, S. J., Monty, J. P. & Marusic, I. 2018 Estimating large-scale structures in wall turbulence using linear models. J. Fluid Mech. 842, 146–162.Google Scholar
Lehoucq, R. B. & Sorensen, D. C. 1996 Deflation techniques for an implicitly restarted Arnoldi iteration. SIAM J. Matrix Anal. Applics. 17 (4), 789–821.Google Scholar
Lesshafft, L., Semeraro, O., Jaunet, V., Cavalieri, A. V. G. & Jordan, P. 2019 Resolvent-based modeling of coherent wavepackets in a turbulent jet. Phys. Rev. Fluids 4, 063901.Google Scholar
Lumley, J. L. 1967 The structure of inhomogeneous turbulent flows. In Atmospheric Turbulence and Radio Propagation (ed. Yaglom, A. M. & Tatarski, V. I.) pp. 168–178. Nauka.Google Scholar
Lumley, J. L. 1970 Stochastic Tools in Turbulence. Academic Press.Google Scholar
McKeon, B. J. 2017 The engine behind (wall) turbulence: perspectives on scale interactions. J. Fluid Mech. 817, P1.Google Scholar
McKeon, B. J. & Sharma, A. S. 2010 A critical-layer framework for turbulent pipe flow. J. Fluid Mech. 658, 336–382.Google Scholar
McKeon, B. J., Sharma, A. S. & Jacobi, I. 2013 Experimental manipulation of wall turbulence: A systems approach. Phys. Fluids 25, 031301.Google Scholar
Moarref, R., Sharma, A. S., Tropp, J. A. & McKeon, B. J. 2013 Model-based scaling of the streamwise energy density in high-Reynolds-number turbulent channels. J. Fluid Mech. 734, 275–316.Google Scholar
Monokrousos, A., Åverik, E., Brandt, L. & Henningson, D. S. 2010 Global three-dimensional optimal disturbances in the blasius boundary-layer flow using time-steppers. J. Fluid Mech. 650, 181–214.Google Scholar
Raffel, M., Willert, C. E., Scarano, F., Kähler, C. J., Wereley, S. T. & Kompenhans, J. 2018 Particle Image Velocimetry – A Practical Guide. Springer.Google Scholar
Rosenberg, K., Symon, S. & McKeon, B. J. 2019 The role of parasitic modes in nonlinear closure via the resolvent feedback loop. Phys. Rev. Fluids 4, 052601(R).Google Scholar
Rowley, C. W., Mezić, I., Bagheri, S., Schlatter, P. & Henningson, D. S. 2009 Spectral analysis of nonlinear flows. J. Fluid Mech. 641, 115–127.Google Scholar
Sasaki, K., Piantanida, S., Cavalieri, A. V. G. & Jordan, P. 2017 Real-time modelling of wavepackets in turbulent jets. J. Fluid Mech. 821, 458–481.Google Scholar
Schmid, P. J. 2010 Dynamic mode decomposition of numerical and experimental data. J. Fluid Mech. 656, 5–28.Google Scholar
Schmidt, O. T. & Towne, A. 2019 An efficient streaming algorithm for spectral proper orthogonal decomposition. Comput. Phys. Commun. 237, 98–109.Google Scholar
Schmidt, O. T., Towne, A., Rigas, G., Colonius, T. & Brès, G. A. 2018 Spectral analysis of jet turbulence. J. Fluid Mech. 855, 953–982.Google Scholar
Sharma, A. S., Mezić, I. & McKeon, B. J. 2016 Correspondence between Koopman mode decomposition, resolvent mode decomposition, and invariant solutions of the Navier–Stokes equations. Phys. Rev. Fluids 1, 032402(R).Google Scholar
da Silva, A. F. C.2019 An EnKF-based flow estimator for aerodynamic flows. PhD thesis, California Institute of Technology, Pasadena, CA.Google Scholar
Sipp, D. & Lebedev, A. 2007 Global stability of base and mean flows: a general approach and its applications to cylinder and open cavity flows. J. Fluid Mech. 593, 333–358.Google Scholar
Sipp, D. & Marquet, O. 2013 Characterization of noise amplifiers with global singular modes: the case of the leading-edge flat-plate boundary layer. Theor. Comput. Fluid Dyn. 27, 617–635.Google Scholar
Sipp, D., Marquet, O., Meliga, P. & Barbagallo, A. 2010 Dynamics and control of instabilities in open-flows: a linearized approach. Appl. Mech. Rev. 63, 030801.Google Scholar
Symon, S.2018 Reconstruction and estimation of flows using resolvent analysis and data-assimilation. PhD thesis, California Institute of Technology, Pasadena, CA.Google Scholar
Symon, S., Dovetta, N., McKeon, B. J., Sipp, D. & Schmid, P. J. 2017 Data assimilation of mean velocity from 2D PIV measurements of flow over an idealized airfoil. Exp. Fluids 58 (5), 61.Google Scholar
Symon, S. & McKeon, B. J. 2018 Experimental flow reconstruction using resolvent analysis and data-assimilation. In 21st Australasian Fluid Mechanics Conference, Adelaide, Australia Google Scholar
Symon, S., Rosenberg, K., Dawson, S. T. M. & McKeon, B. J. 2018 Non-normality and classification of amplification mechanisms in stability and resolvent analysis. Phys. Rev. Fluids 3, 053902.Google Scholar
Symon, S., Sipp, D., Schmid, P. J. & McKeon, B. J. 2019 Mean and unsteady flow reconstruction using data-assimilation and resolvent analysis. AIAA J. (in press).Google Scholar
Thomareis, N. & Papadakis, G. 2018 Resolvent analysis of separated and attached flows around an airfoil at transitional Reynolds number. Phys. Rev. Fluids 3, 073901.Google Scholar
Towne, A.2016 Advancements in jet turbulence and noise modeling: accurate one-way solutions and empirical evaluation of the nonlinear forcing of wavepackets. PhD thesis, California Institute of Technology.Google Scholar
Towne, A., Colonius, T., Jordan, P., Cavalieri, A. V. G. & Brès, G. A. 2015 Stochastic and nonlinear forcing of wavepackets in a Mach 0.9 jet. In 21st AIAA/CEAS Aeroacustics Conference. AIAA Paper 2015-2217.Google Scholar
Towne, A., Lozano-Durán, A. & Yang, X.2019 Resolvent-based estimation of space-time flow statistics. J. Fluid Mech. (submitted) arXiv:1901.07478.Google Scholar
Towne, A., Schmidt, O. T. & Colonius, T. 2018 Spectral proper orthogonal decomposition and its relationship to dynamic mode decomposition and resolvent analysis. J. Fluid Mech. 847, 821–867.Google Scholar
Weihs, D. & Katz, J. 1983 Cellular patterns in poststall flow over unswept wings. AIAA J. 21 (12), 1757–1759.Google Scholar
Welch, P. 1967 The use of fast Fourier transform for the estimation of power spectra: a method based on time averaging over short, modified periodograms. IEEE Trans. Audio Electroacoust. 2, 70–73.Google Scholar
Westerweel, J. & Scarano, F. 2005 Universal outlier detection for PIV data. Exp. Fluids 39 (6), 1096–1100.Google Scholar
Yeh, C. A. & Taira, K. 2019 Resolvent-analysis-based design of airfoil separation control. J. Fluid Mech. 867, 572–610.Google Scholar

Symon et al. supplementary movie 1

Reduced-order model for the A0 case containing 91 resolvent modes.

Download Symon et al. supplementary movie 1(Video)
Video 6.7 MB

Symon et al. supplementary movie 2

Filtered PIV for the A0 case containing the same frequencies as the resolvent model.

Download Symon et al. supplementary movie 2(Video)
Video 8.6 MB

Symon et al. supplementary movie 3

Filtered PIV for the A10 case containing 66 frequencies in the range $3.14 < \omega < 17.7$.

Download Symon et al. supplementary movie 3(Video)
Video 9.9 MB

Symon et al. supplementary movie 4

Reduced-order model for the A10 case containing 66 resolvent modes.

Download Symon et al. supplementary movie 4(Video)
Video 8.2 MB