Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Song, Jiaxing
Wan, Zhen-Hua
Liu, Nansheng
Lu, Xi-Yun
and
Khomami, Bamin
2021.
A reverse transition route from inertial to elasticity-dominated turbulence in viscoelastic Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 927,
Issue. ,
Lacassagne, Tom
Boulafentis, Theofilos
Cagney, Neil
and
Balabani, Stavroula
2021.
Modulation of elasto-inertial transitions in Taylor–Couette flow by small particles.
Journal of Fluid Mechanics,
Vol. 929,
Issue. ,
Lacassagne, Tom
Cagney, Neil
and
Balabani, Stavroula
2021.
Shear-thinning mediation of elasto-inertial Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 915,
Issue. ,
Ohmura, Naoto
Masuda, Hayato
and
Wang, Steven
2022.
Vortex Dynamics - From Physical to Mathematical Aspects.
Lopez, Jose M.
2022.
Vortex merging and splitting events in viscoelastic Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Topayev, S.
Nouar, C.
and
Dusek, J.
2022.
Secondary instabilities in Taylor–Couette flow of shear-thinning fluids.
Journal of Fluid Mechanics,
Vol. 933,
Issue. ,
Stathoulopoulos, Antonios
Passos, Andreas
and
Balabani, Stavroula
2022.
Flows of healthy and hardened RBC suspensions through a micropillar array.
Medical Engineering & Physics,
Vol. 107,
Issue. ,
p.
103874.
Xu, Yufu
Bao, Yichen
Liu, Zhichao
Zheng, Quan
Dong, Yinhui
Song, Ruhong
and
Yang, Bingxun
2022.
Temperature-sensitive tribological performance of titanium alloy lubricated with PNIPAM microgels.
Applied Surface Science,
Vol. 572,
Issue. ,
p.
151392.
Lopez, Jose M.
and
Altmeyer, Sebastian A.
2023.
Arrow-shaped elasto-inertial rotating waves.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2246,
Moazzen, Masoud
Lacassagne, Tom
Thomy, Vincent
and
Bahrani, S. Amir
2023.
Friction dynamics of elasto-inertial turbulence in Taylor–Couette flow of viscoelastic fluids.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2246,
Pryazhnikov, Maxim
Pryazhnikov, Andrey
Skorobogatova, Angelica
Minakov, Andrey
and
Ivleva, Yulia
2023.
Microfluidic Study of Enhanced Oil Recovery during Flooding with Polyacrylamide Polymer Solutions.
Micromachines,
Vol. 14,
Issue. 6,
p.
1137.
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2023.
Experimental insights into elasto-inertial transitions in Taylor–Couette flows.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2243,
Dastbaz, Zahra
and
Ashrafizadeh, Seyed Nezameddin
2024.
Intensifying the performance of polymer suspensions to evaluate drag reduction using rotating disc apparatus.
Arabian Journal of Chemistry,
Vol. 17,
Issue. 8,
p.
105858.
Ghanbari, Reza
Pashazadeh, Sajjad
Sekar, Kesavan
Nygård, Kim
Terry, Ann
Liebi, Marianne
Matic, Aleksandar
and
Kádár, Roland
2024.
Painting Taylor vortices with cellulose nanocrystals: Suspension flow supercritical spectral dynamics.
Physics of Fluids,
Vol. 36,
Issue. 4,
Nouri, Sabrina
Mahloul, Mohamed
Hadj Arab, Belaid
Benmalek, Toufik
and
Aksouh, Mohamed
2024.
First hydrodynamic instability and flow transitions between concentric vertical cylinders.
Physics of Fluids,
Vol. 36,
Issue. 12,
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2024.
Elastically modulated wavy vortex flow.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 330,
Issue. ,
p.
105283.
Boulafentis, T.
Lacassagne, T.
Cagney, N.
and
Balabani, S.
2024.
Coherent structures of elastoinertial instabilities in Taylor–Couette flows.
Journal of Fluid Mechanics,
Vol. 986,
Issue. ,
Clarke, Andrew
and
Davoodi, Mahdi
2025.
The movement of particles in Taylor–Couette flow of complex fluids.
Journal of Non-Newtonian Fluid Mechanics,
Vol. 335,
Issue. ,
p.
105354.
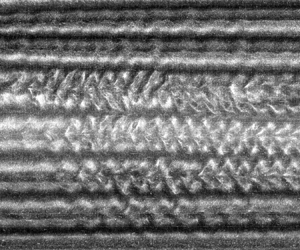
 ${\geq }1000\ \textrm {ppm}$ concentration), the flow becomes highly unsteady soon after the formation of Taylor vortices, with substantial changes in the waviness with Reynolds number, which are shown to be highly repeatable. Vortices are found to suddenly merge as the Reynolds number increases, with the number of mergers increasing with polymer concentration. These abrupt changes in wavelength are highly hysteretic and can occur in both steady and wavy regimes. Finally, the vortices in moderate and dense polymer solutions are shown to undergo a gradual drift in both their size and position, which appears to be closely linked to the splitting and merger of vortices.
${\geq }1000\ \textrm {ppm}$ concentration), the flow becomes highly unsteady soon after the formation of Taylor vortices, with substantial changes in the waviness with Reynolds number, which are shown to be highly repeatable. Vortices are found to suddenly merge as the Reynolds number increases, with the number of mergers increasing with polymer concentration. These abrupt changes in wavelength are highly hysteretic and can occur in both steady and wavy regimes. Finally, the vortices in moderate and dense polymer solutions are shown to undergo a gradual drift in both their size and position, which appears to be closely linked to the splitting and merger of vortices.