Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-13T15:52:38.590Z Has data issue: false hasContentIssue false
  • English
  • Français

The turbulent/non-turbulent interface and entrainment in a boundary layer

Published online by Cambridge University Press:  21 February 2014

Kapil Chauhan ,
Jimmy Philip ,
Charitha M. de Silva ,
Nicholas Hutchins  and
Ivan Marusic
Kapil Chauhan*
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
Jimmy Philip
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
Charitha M. de Silva
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
Nicholas Hutchins
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
Ivan Marusic
Affiliation:
Department of Mechanical Engineering, The University of Melbourne, Parkville, VIC 3010, Australia
*
†Email address for correspondence: kchauhan@unimelb.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

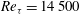The turbulent/non-turbulent interface in a zero-pressure-gradient turbulent boundary layer at high Reynolds number ($\mathit{Re}_\tau =14\, 500$) is examined using particle image velocimetry. An experimental set-up is utilized that employs multiple high-resolution cameras to capture a large field of view that extends 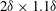$2\delta \times 1.1\delta $ in the streamwise/wall-normal plane with an unprecedented dynamic range. The interface is detected using a criteria of local turbulent kinetic energy and proves to be an effective method for boundary layers. The presence of a turbulent/non-turbulent superlayer is corroborated by the presence of a jump for the conditionally averaged streamwise velocity across the interface. The steep change in velocity is accompanied by a discontinuity in vorticity and a sharp rise in the Reynolds shear stress. The conditional statistics at the interface are in quantitative agreement with the superlayer equations outlined by Reynolds (J. Fluid Mech., vol. 54, 1972, pp. 481–488). Further analysis introduces the mass flux as a physically relevant parameter that provides a direct quantitative insight into the entrainment. Consistency of this approach is first established via the equality of mean entrainment calculations obtained using three different methods, namely, conditional, instantaneous and mean equations of motion. By means of ‘mass-flux spectra’ it is shown that the boundary-layer entrainment is characterized by two distinctive length scales which appear to be associated with a two-stage entrainment process and have a substantial scale separation.

JFM classification

Turbulent Flows: Intermittency Turbulent Flows: Turbulent boundary layers Turbulent Flows: Turbulent Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 742 , 10 March 2014 , pp. 119 - 151
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adrian, R. J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19, 041301.Google Scholar
Anand, R., Boersma, B. J. & Agrawal, A. 2009 Detection of turbulent/non-turbulent interface for an axisymmetric turbulent jet: evaluation of known criteria and proposal of a new criterion. Exp. Fluids 47 (6), 995–1007.CrossRefGoogle Scholar
Antonia, R. A. 1972 Conditionally sampled measurements near the outer edge of a turbulent boundary layer. J. Fluid Mech. 56 (1), 1–18.Google Scholar
Bisset, D. K., Hunt, J. C. R. & Rogers, M. M. 2002 The turbulent/non-turbulent interface bounding a far wake. J. Fluid Mech. 451, 383–410.Google Scholar
Brown, G. L. & Roshko, A. 1974 On density effects and large structure in turbulent mixing layers. J. Fluid Mech. 64, 775–816.Google Scholar
Cannon, S., Champagne, E. & Glezer, A. 1993 Observations of large-scale structures in wakes behind axisymmetric bodies. Exp. Fluids 14, 447–450.Google Scholar
Chauhan, K. A., Monkewitz, P. A. & Nagib, H. M. 2009 Criteria for assessing experiments in zero pressure gradient boundary layers. Fluid Dyn. Res. 41, 021404.CrossRefGoogle Scholar
Chen, C.-H. P. & Blackwelder, R. F. 1978 Large-scale motion in a turbulent boundary layer: a study using temperature contamination. J. Fluid Mech. 89, 1–31.Google Scholar
Coles, D. E. & Hirst, E. A. 1968 Computation of turbulent boundary layers. In Proceedings of AFOSR-IFP Stanford Conference, vol. 2 .Google Scholar
Corrsin, S. & Kistler, A. L.1955 Free-stream boundaries of turbulent flows. Tech. Rep. TN-1244. NACA, Washington, DC.Google Scholar
da Silva, C. B. & dos Reis, R. J. N. 2011 The role of coherent vortices near the turbulent/non-turbulent interface in a planar jet. Phil. Trans. R. Soc. Lond. A Math. Phys. Sci. 369, 738–753.Google Scholar
da Silva, C. B. & Pereira, J. C. F. 2008 Invariants of the velocity-gradient, rate-of-strain, and rate-of-rotation tensors across the turbulent/nonturbulent interface in jets. Phys. Fluids 20 (5), 055101.CrossRefGoogle Scholar
da Silva, C. B. & Taveira, R. R. 2010 The thickness of the turbulent/nonturbulent interface is equal to the radius of the large vorticity structures near the edge of the shear layer. Phys. Fluids 22, 121702.Google Scholar
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
de Silva, C. M., Chauhan, K. A., Atkinson, C. H., Buchmann, N. A., Hutchins, N., Soria, J. & Marusic, I.2012 Implementation of large-scale PIV measurements for wall bounded turbulence at high Reynolds numbers. In Proceedings of 18th Australasian Fluid Mechanics Conference, 3–7 December, Launceston, Australia.Google Scholar
de Silva, C. M., Philip, J., Chauhan, K., Meneveau, C. & Marusic, I. 2013 Multiscale geometry and scaling of the turbulent-nonturbulent interface in high Reynolds number boundary layers. Phys. Rev. Lett. 111, 044501.Google Scholar
Fackrell, J. E. & Robins, A. G. 1982 Concentration fluctuations and fluxes in plumes from point sources in a turbulent boundary layer. J. Fluid Mech. 117, 1–26.Google Scholar
Fiedler, H. & Head, M. R. 1966 Intermittency measurements in the turbulent boundary layer. J. Fluid Mech. 25, 719–735.Google Scholar
Hancock, P. E. & Bradshaw, P. 1989 Turbulence structure of a boundary layer beneath a turbulent free stream. J. Fluid Mech. 205, 45–76.Google Scholar
Hart, D. P. 2000 PIV error correction. Exp. Fluids 29 (1), 13–22.Google Scholar
Head, M. R. 1958 Entrainment in the turbulent boundary layer. Aero. Res. Counc. Rep 3152.Google Scholar
Hedley, T. B. & Keffer, J. F. 1974a Some turbulent/non-turbulent properties of the outer intermittent region of a boundary layer. J. Fluid Mech. 64, 645–678.CrossRefGoogle Scholar
Hedley, T. B. & Keffer, J. F. 1974b Turbulent/non-turbulent decisions in an intermittent flow. J. Fluid Mech. 64, 625–644.Google Scholar
Holzner, M., Liberzon, A., Nikitin, N., Kinzelbach, W. & Tsinober, A. 2007 Small-scale aspects of flows in proximity of the turbulent/nonturbulent interface. Phys. Fluids 19, 071702.Google Scholar
Holzner, M. & Lüthi, B. 2011 Laminar superlayer at the turbulence boundary. Phys. Rev. Lett. 106, 134503.Google Scholar
Hunt, J. C. R. 1994 Atmospheric jets and plumes. In Recent Research Advances in the Fluid Mechanics of Turbulent Jets and Plumes (ed. Davies, P. & Neves, M. J. V.), pp. 309–334. Kluwer.Google Scholar
Hunt, J. C. R., Rottman, J. W. & Britter, R. E. 1984 Some physical processes involved in the dispersion of dense gases. In Proceedings of IUTAM Symposium ‘Atmospheric Dispersion of Heavy Gases and Small Particles’ (ed. Ooms, G. & Tennekes, H.), pp. 361–395. Springer.Google Scholar
Hutchins, N., Nickels, T. B., Marusic, I. & Chong, M. S. 2009 Hot-wire spatial resolution issues in wall-bounded turbulence. J. Fluid Mech. 635, 103–136.Google Scholar
Ishihara, T., Hunt, J. C. R. & Kaneda, Y. 2012 Intense dissipative mechanisms of strong thin shear layers in high Reynolds number turbulence. Bull. Am. Phys. Soc. 57 (17), A23.00004.Google Scholar
Jiménez, J., Hoyas, S., Simens, M. P. & Mizuno, Y. 2010 Turbulent boundary layers and channels at moderate Reynolds number. J. Fluid Mech. 657, 335–360.Google Scholar
Khashehchi, M., Ooi, A., Soria, J. & Marusic, I. 2013 Evolution of the turbulent/non-turbulent interface of an axisymmetric turbulent jet. Exp. Fluids 54 (1), 1–12.Google Scholar
Kovasznay, L. S. G. 1967 Structure of the turbulent boundary layer. Phys. Fluids Suppl. 10, S25–S30.Google Scholar
Kovasznay, L. S. G. 1970 The turbulent boundary layer. Annu. Rev. Fluid Mech. 2, 95–112.Google Scholar
Kovasznay, L. S. G., Kibens, V. & Blackwelder, R. F. 1970 Large-scale motion in the intermittent region of a turbulent boundary layer. J. Fluid Mech. 41, 283–325.CrossRefGoogle Scholar
Kulandaivelu, V.2012 Evolution of zero pressure gradient turbulent boundary layers from different initial conditions. PhD thesis, The University of Melbourne, Melbourne, Australia.Google Scholar
Marusic, I. & Kunkel, G. J. 2003 Streamwise turbulence intensity formulation for flat-plate boundary layers. Phys. Fluids 15, 2461–2464.Google Scholar
Mathew, J. & Basu, A. J. 2002 Some characteristics of entrainment at a cylindrical turbulence boundary. Phys. Fluids 14, 2065–2072.CrossRefGoogle Scholar
Meneveau, C. & Sreenivasan, K. R. 1990 Interface dimension in intermittent turbulence. Phys. Rev. A 41 (4), 2246–2248.Google Scholar
Monkewitz, P. A., Chauhan, K. A. & Nagib, H. M. 2007 Self-consistent high-Reynolds-number asymptotics for zero-pressure-gradient turbulent boundary layers. Phys. Fluids 19, 115101.CrossRefGoogle Scholar
Nychas, S. G., Hershey, H. C. & Brodkey, R. S. 1973 A visual study of turbulent shear flow. J. Fluid Mech. 61, 513–540.Google Scholar
Paizis, S. T. & Schwarz, W. H. 1974 An investigation of the topography and motion of the turbulent interface. J. Fluid Mech. 63 (2), 315–343.Google Scholar
Philip, J. & Marusic, I. 2012 Large-scale eddies and their role in entrainment in turbulent jets and wakes. Phys. Fluids 24, 055108.Google Scholar
Philip, J., Meneveau, C., de Silva, C. M. & Marusic, I. 2014 Multiscale analysis of fluxes at the turbulent/non-turbulent interface in high Reynolds number boundary layers. Phys. Fluids 26, 015105.Google Scholar
Phillips, O. M. 1972 The entrainment interface. J. Fluid Mech. 51, 97–118.Google Scholar
Prasad, R. R. & Sreenivasan, K. R. 1989 Scalar interfaces in digital images of turbulent flows. Exp. Fluids 7 (4), 259–264.Google Scholar
Reynolds, W. C. 1972 Large-scale instabilities of turbulent wakes. J. Fluid Mech. 54, 481–488.Google Scholar
Sandham, N. D., Mungal, M. G., Broadwell, J. E. & Reynolds, W. C. 1988 Scalar entrainment in the mixing layer. In Proceedings of the Summer Program, CTR - Stanford pp. 69–76.Google Scholar
Schneider, W. 1981 Flow induced by jets and plumes. J. Fluid Mech. 108 (1), 55–65.Google Scholar
Semin, N. V., Golub, V. V., Elsinga, G. E. & Westerweel, J. 2011 Laminar superlayer in a turbulent boundary layer. Tech. Phys. Lett. 37 (12), 1154–1157.Google Scholar
Sreenivasan, K. R. 1991 Fractals and multifractals in fluid turbulence. Annu. Rev. Fluid Mech. 23 (1), 539–604.Google Scholar
Sreenivasan, K. R. & Meneveau, C. 1986 The fractal facets of turbulence. J. Fluid Mech. 173, 357–386.Google Scholar
Sreenivasan, K. R., Ramshankar, R. & Meneveau, C. 1989 Mixing, entrainment and fractal dimensions of surfaces in turbulent flows. Phil. Trans. R. Soc. Lond. A Math. Phys. Sci. 421 (1860), 79–108.Google Scholar
Taylor, G. I. 1921 Diffusion by continuous movements. Proc. Lond. Math. Soc. 20, 196–211.Google Scholar
Taylor, G. I. 1958 Flow induced by jets. J. Aerosp. Sci. 25, 464–465.Google Scholar
Thompson, B. G. J. 1964 A critical review of existing methods of calculating the turbulent boundary layer. Aero. Res. Counc. Rep 3447.Google Scholar
Townsend, A. A. 1966 The mechanism of entrainment in free turbulent flows. J. Fluid Mech. 26, 689–715.Google Scholar
Townsend, A. A. 1976 The Structure of Turbulent Shear Flow. Cambridge University Press.Google Scholar
Turner, J. S. 1986 Turbulent entrainment: the development of the entrainment assumption, and its application to geophysical flows. J. Fluid Mech. 173, 431–471.CrossRefGoogle Scholar
van Reeuwijk, M. & Holzner, M. 2014 The turbulence boundary of a temporal jet.. J. Fluid Mech. 739, 254–275.Google Scholar
Westerweel, J., Fukushima, C., Pedersen, J. M. & Hunt, J. C. R. 2005 Mechanics of the turbulent-nonturbulent interface of a jet. Phys. Rev. Lett. 95, 174501.Google ScholarPubMed
Westerweel, J., Fukushima, C., Pedersen, J. M. & Hunt, J. C. R. 2009 Momentum and scalar transport at the turbulent/non-turbulent interface of a jet. J. Fluid Mech. 631, 199–230.Google Scholar