Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-30T22:39:06.307Z Has data issue: false hasContentIssue false
  • English
  • Français

Very small insects use novel wing flapping and drag principle to generate the weight-supporting vertical force

Published online by Cambridge University Press:  19 September 2018

Xin Cheng  and
Mao Sun Open the ORCID record for Mao Sun [Opens in a new window]
Xin Cheng
Affiliation:
Institute of Fluid Mechanics, Behang University, Beijing 100191, China
Mao Sun*
Affiliation:
Institute of Fluid Mechanics, Behang University, Beijing 100191, China
*
†Email address for correspondence: m.sun@buaa.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

The effect of air viscosity on the flow around an insect wing increases as insect size decreases. For the smallest insects (wing length $R$ below 1 mm), the viscous effect is so large that lift-generation mechanisms used by their larger counterparts become ineffective. How the weight-supporting vertical force is generated is unknown. To elucidate the aerodynamic mechanisms responsible, we measure the wing kinematics of the tiny wasp Encarsia formosa (0.6 mm 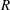$R$) in hovering or very slow ascending flight and compute and analyse the aerodynamic forces. We find that the insects perform two unusual wing motions. One is ‘rowing’: the wings move fast downward and backward, like stroking oars. The other is the previously discovered Weis-Fogh ‘fling’. The rowing produces 70 % of the required vertical force and the Weis-Fogh ‘fling’ the other 30 %. The oaring wing mainly produces an approximately up-pointing drag, resulting in the vertical force. Because each oaring produces a starting flow, the drag is unsteady in nature and much greater than that in steady motion at the same velocities and angles of attack. Furthermore, our computation shows that if the tiny wasps employed the usual wing kinematics of the larger insects (flapping back and forth in a horizontal plane), the vertical force produced would be only 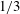$1/3$ of that by the real wing kinematics; i.e. they must use the special wing movements to overcome the problem of large viscous effects encountered by the commonly used flapping kinematics. We have observed for the first time very small insects using drag to support their weight and we explain how a net vertical force is generated when the drag principle is applied.

JFM classification

Biological Fluid Dynamics: Biological Fluid Dynamics Biological Fluid Dynamics: Swimming/flying
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 855 , 25 November 2018 , pp. 646 - 670
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arora, N., Gupta, A., Sanghi, S., Aono, H. & Shyy, W. 2014 Lift–drag and flow structures associated with the ‘clap and fling’ motion. Phys. Fluids 26, 071906.Google Scholar
Birch, J. M., Dickson, W. B. & Dickson, M. H. 2004 Force production and flow structure of the leading edge vortex on flapping wings at high and low Reynolds numbers. J. Expl Biol. 207, 1063–1072.Google Scholar
Bomphrey, R. J., Srygley, R. B., Taylor, G. K. & Thomas, A. L. R. 2002 Visualizing the flow around insect wings. Phys. Fluids 14, S4.Google Scholar
Carr, Z. R., Chen, C. & Ringuette, M. J. 2013 Finite-span rotating wings: three-dimensional vortex formation and variations with aspect ratio. Exp. Fluids 54, 1444.Google Scholar
Dickinson, M. H., Lehmann, F. O. & Sane, S. P. 1999 Wing rotation and the aerodynamic basis of insect flight. Science 284 (5422), 1954–1960.Google Scholar
Du, G. & Sun, M. 2012 Aerodynamic effects of corrugation and deformation in flapping wings of hovering hoverflies. J. Theor. Biol. 300, 19–28.Google Scholar
Dudley, R. 2002 The Biomechanics of Insect Flight: Form, Function, Evolution. Princeton University Press.Google Scholar
Ellington, C. P. 1975 Non-steady-state aerodynamic of the flight of Encarsia formosa . In Swimming and Flying in Nature (ed. Wu, T. Y., Brokaw, C. J. & Brennen, C.), vol. 2, pp. 729–762. Plenum.Google Scholar
Ellington, C. P. 1984a The aerodynamics of hovering insect flight. Part I. The quasi-steady analysis. Phil. Trans. R. Soc. Lond. B 305 (1122), 1–15.Google Scholar
Ellington, C. P. 1984b The aerodynamics of hovering insect flight. Part III. Kinematics. Phil. Trans. R. Soc. Lond. B 305 (1122), 41–78.Google Scholar
Ellington, C. P. 1984c The aerodynamics of hovering insect flight. Part IV. Aerodynamic mechanisms. Phil. Trans. R. Soc. Lond. B 305 (1122), 79–113.Google Scholar
Ellington, C. P. 1984d The aerodynamics of hovering insect flight. Part VI. Lift and power requirements. Phil. Trans. R. Soc. Lond. B 305 (1122), 145–181.Google Scholar
Ellington, C. P., van den Berg, C., Willmott, A. P., Ellington, C. P. & Thomas, A. L. R. 1996 Leading-edge vortices in insect flight. Nature 384, 626–630.Google Scholar
Garmann, D. J., Visbal, M. R. & Orkwis, P. D. 2013 Three-dimensional flow structure and aerodynamic loading on a revolving wing. Phys. Fluids 25, 034101.Google Scholar
Han, J.-S., Chang, J. W. & Han, J.-H. 2016 The advance ratio effect on the lift augmentations of an insect-like flapping wing in forward flight. J. Fluid Mech. 808, 485–510.Google Scholar
Harbig, R. R., Sheridan, J. & Thompson, M. C. 2013 Reynolds number and aspect ratio effects on the leading-edge vortex for rotating insect wing planforms. J. Fluid Mech. 717, 166–192.Google Scholar
Hilgenstock, A. 1988 A fast method for the elliptic generation of three dimensional grids with full boundary control. In Numerical Grid Generation in Computational Fluid Mechanics, Swansea, UK (ed. Sengupta, S., Hauster, J., Eiseman, P. R. & Thompson, J. F.), pp. 137–146. Pineridge Press.Google Scholar
Horridge, G. A. 1956 The flight of very small insects. Nature 178, 1334–1335.Google Scholar
Howe, M. S. 1989 On unsteady surface forces, and sound produced by the normal chopping of a rectilinear vortex. J. Fluid Mech. 206, 131–153.Google Scholar
Howe, M. S. 1995 On the force and moment on a body in an incompressible fluid, with application to rigid bodies and bubbles at high and low Reynolds numbers. Q. J. Mech. Appl. Maths 48, 401–426.Google Scholar
Jardin, T., Farcy, A. & David, L. 2012 Three-dimensional effects in hovering flapping flight. J. Fluid Mech. 702, 102–125.Google Scholar
Jones, S. K., Laurenza, R., Hedrick, T. L., Griffith, B. E. & Miller, L. A. 2015 Lift versus drag based mechanisms for vertical force production in the smallest flying insects. J. Theor. Biol. 384, 105–120.Google Scholar
Kim, D. & Gharib, M. 2010 Experimental study of three-dimensional vortex structures in translating and rotating plates. Exp. Fluids 49, 329–339.Google Scholar
Lentink, D. & Dickinson, M. H. 2009 Rotational accelerations stabilize leading edge vortices on revolving fly wings. J. Expl Biol. 212, 2705–2719.Google Scholar
Liu, H., Ellington, C. P., Kawachi, K., van den Berg, C. & Willmott, A. P. 1998 A computational fluid dynamic study of hawkmoth hovering. J. Expl Biol. 201, 461–477.Google Scholar
Liu, L. G. & Sun, M. 2018 The added mass forces in insect flapping wings. J. Theor. Biol. 437, 45–50.Google Scholar
Liu, Y. P. & Sun, M. 2008 Wing kinematics measurement and aerodynamics of hovering droneflies. J. Expl Biol. 211, 2014–2025.Google Scholar
Luo, G. Y. & Sun, M. 2005 The effects of corrugation and wing planform on the aerodynamic force production of sweeping model insect wings. Acta Mechanica Sin. 21, 531–541.Google Scholar
Manar, F. & Jones, A. R.2014 The effect of tip clearance on low Reynolds number rotating wings. AIAA Paper 2014-1452.Google Scholar
Meng, X. G. & Sun, M. 2013 Aerodynamic effects of wing corrugation at gliding flight at low Reynolds numbers. Phys. Fluids 25, 071905.Google Scholar
Miller, L. A. & Peskin, C. S. 2004 When vortices stick: an aerodynamic transition in tiny insect flight. J. Expl Biol. 207, 3073–3088.Google Scholar
Miller, L. A. & Peskin, C. S. 2005 A computational fluid dynamics of ‘clap and fling’ in the smallest insects. J. Expl Biol. 208, 195–212.Google Scholar
Mou, X. L., Liu, Y. P. & Sun, M. 2011 Wing motion measurement and aerodynamics of hovering true hoverflies. J. Expl Biol. 214, 2832–2844.Google Scholar
Ozen, C. A. & Rockwell, D. 2012 Three-dimensional vortex structure on a rotating wing. J. Fluid Mech. 707, 541–550.Google Scholar
Rogers, S. E., Kwak, D. & Kiris, C. 1991 Steady and unsteady solutions of the incompressible Navier–Stokes equations. AIAA J. 29, 603–610.Google Scholar
Rogers, S. E. & Pulliam, T. H.1994 Accuracy enhancements for overset grids using a defect correction approach. AIAA Paper 94-0523.Google Scholar
Sane, S. P. 2003 The aerodynamics of insect flight. J. Expl Biol. 206, 4191–4208.Google Scholar
Shyy, W., Aono, H., Chimakurthi, S. K., Trizila, P., Kang, C. K., Cesink, C. E. S. & Liu, H. 2010 Recent progress in flapping wing aerodynamics and aeroelasticity. Prog. Aerosp. Sci. 46, 284–327.Google Scholar
Shyy, W., Trizila, P., Kang, C. K. & Aono, H. 2009 Can tip vortices enhance lift of a flapping wing? AIAA J. 47, 289–293.Google Scholar
Spedding, G. R. & Maxworthy, T. 1986 The generation of circulation and lift in a rigid two-dimensional fling. J. Fluid Mech. 165, 247–272.Google Scholar
Sun, M. & Du, G. 2003 Lift and power requirements of hovering insect flight. Acta Mechanica Sin. 19, 458–469.Google Scholar
Sun, M. & Lan, S. L. 2004 A computational study of the aerodynamic forces and power requirements of dragonfly (Aeschna juncea) hovering. J. Expl Biol. 207, 1887–1901.Google Scholar
Sun, M. & Wu, J. H. 2004 Large aerodynamic forces on a sweeping wing at low Reynolds number. Acta Mechanica Sin. 20, 24–31.Google Scholar
Sun, M. & Yu, X. 2003 Flows around two airfoils performing fling and subsequent translation and subsequent clap. Acta Mechanica Sin. 19, 103–117.Google Scholar
Sun, M. & Yu, X. 2006 Aerodynamic force generation in hovering flight in a tiny insect. AIAA J. 44, 1532–1540.Google Scholar
Sunada, S., Takashima, H., Hattori, T., Yasuda, K. & Kawachi, K. 2002 Fluid-dynamic characteristics of a bristled wing. J. Expl Biol. 205, 2737–2744.Google Scholar
Wakeling, J. M. & Ellington, C. P. 1997 Dragonfly flight. Part II. Velocities, accelerations and kinematics of flapping flight. J. Expl Biol. 200, 557–582.Google Scholar
Wang, Z. J. 2004 The role of drag in insect hovering. J. Expl Biol. 207, 4147–4155.Google Scholar
Wang, Z. J. 2005 Dissecting insect flight. Annu. Rev. Fluid Mech. 37, 183–210.Google Scholar
Weis-Fogh, T. 1973 Quick estimates of flight fitness in hovering animals, including novel mechanisms for lift production. J. Expl Biol. 59, 169–203.Google Scholar
Weis-Fogh, T. 1975 Flapping flight and power in birds and insects, conventional and novel mechanisms. In Swimming and Flying in Nature (ed. Wu, T. Y., Brokaw, C. J. & Brennen, C.), vol. 2, pp. 729–762. Plenum.Google Scholar
White, F. M. 1991 Viscous Fluid Flow, vol. 2. McGraw-Hill Higher Education.Google Scholar
Wojcik, C. J. & Buchholz, J. H. J. 2014 Vorticity transport in the leading-edge vortex on a rotating blade. J. Fluid Mech. 743, 249–261.Google Scholar
Wolfinger, M. & Rockwell, D. 2014 Flow structure on a rotating wing: effect of radius of gyration. J. Fluid Mech. 755, 83–110.Google Scholar
Wu, J. H. & Sun, M. 2004 Unsteady aerodynamic forces of a flapping wing. J. Expl Biol. 207, 1137–1150.Google Scholar
Zhu, H. J. & Sun, M. 2017 Unsteady aerodynamic force mechanisms of a hoverfly hovering with a short stroke-amplitude. Phys. Fluids 29, 081901.Google Scholar

Cheng Supplementary Movie 1

Hover flight of EF1. The left, middle and right parts of the movie show the flight captured by the top-view camera and two side-view cameras, respectively. Playback speed is 15fps, approximately 0.15% of the actual speed of the movie.

Download Cheng Supplementary Movie 1(Video)
Video 1.6 MB

Cheng Supplementary Movie 2

Hover flight of EF2. The left, middle and right parts of the movie show the flight captured by the top-view camera and two side-view cameras, respectively. Playback speed is 15fps, approximately 0.15% of the actual speed of the movie.

Download Cheng Supplementary Movie 2(Video)
Video 1.9 MB

Cheng Supplementary Movie 3

Hover flight of EF5. The left, middle and right parts of the movie show the flight captured by the top-view camera and two side-view cameras, respectively. Playback speed is 15fps, approximately 0.15% of the actual speed of the movie.

Download Cheng Supplementary Movie 3(Video)
Video 1.4 MB