Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-28T19:23:45.406Z Has data issue: false hasContentIssue false
  • English
  • Français

Visualization of the Ludford column

Published online by Cambridge University Press:  13 March 2013

Oleg Andreev ,
Yurii Kolesnikov  and
André Thess
Oleg Andreev
Affiliation:
Institute of Thermodynamics and Fluid Mechanics, Ilmenau University of Technology, PO Box 100565, 98684 Ilmenau, Germany
Yurii Kolesnikov
Affiliation:
Institute of Thermodynamics and Fluid Mechanics, Ilmenau University of Technology, PO Box 100565, 98684 Ilmenau, Germany
André Thess*
Affiliation:
Institute of Thermodynamics and Fluid Mechanics, Ilmenau University of Technology, PO Box 100565, 98684 Ilmenau, Germany
*
†Email address for correspondence: thess@tu-ilmenau.de
Get access Rights & Permissions [Opens in a new window]

Abstract

When a liquid metal flows around a truncated cylinder in the presence of a magnetic field which is parallel to the axis of the cylinder, a stagnant region develops above the cylinder. We call this region a Ludford column. The Ludford column represents the magnetohydrodynamics (MHD) analogue of the well-known Taylor columns in rotating flows. Whereas Taylor columns can be easily visualized using dye, the visualization of Ludford columns has remained elusive up to now because liquid metals are opaque. We demonstrate that this fundamental limitation of experimental MHD can be overcome by using a superconducting 5 T magnet. This facility permits us to perform MHD experiments in which the opaque liquid metals are replaced with a transparent electrolyte while maintaining the key MHD effects. We report results of a series of flow experiments in which an aqueous solution of sulphuric acid flows around a bar with square cross-section (which for simplicity shall be referred to as a cylinder). We vary the Reynolds number in the range $5\lt Re\lt 100$ and the Hartmann number in the range 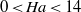$0\lt Ha\lt 14$. The experimental procedure involves flow visualizations using tracer particles as well as velocity measurements using particle image velocimetry (PIV). Our experiments provide direct access to the Ludford column for the first time and reveal the spatial structure of this basic feature of MHD flows.

JFM classification

MHD and Electrohydrodynamics: High-Hartmann-number flows MHD and Electrohydrodynamics: MHD and Electrohydrodynamics MHD and Electrohydrodynamics: MHD turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 721 , 25 April 2013 , pp. 438 - 453
Copyright
©2013 Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Present address: Institute of Fluid Dynamics, Helmholtz-Zentrum Dresden-Rossendorf, Bautzner Landstraße 400, 01328 Dresden, Germany.

References

Andreev, O. & Kolesnikov, Yu 1997 MHD instabilities at transverse flow around a circular cylinder in an axial magnetic field. In Third International Conference on Transfer Phenomena in Magnetohydrodynamic and Electroconducting Flows, pp. 205–210. Aussois.Google Scholar
Branover, G. G., Gel’fgat, Yu. M., Turuntaev, S. V. & Tsinober, A. B. 1969 Effect of a transverse magnetic field on velocity perturbations behind a circular cylinder swept by an electrolyte. Magnetohydrodynamics 5 (3), 41–46.Google Scholar
Davidson, P. A 1997 The role of angular momentum in the magnetic damping of turbulence. J. Fluid Mech. 336, 123–150.Google Scholar
Davidson, P. 1999 Magnetohydrodynamics in materials processing. Annu. Rev. Fluid Mech. 31, 273–300.CrossRefGoogle Scholar
Dousset, V. & Pothérat, A. 2008 Numerical simulations of a cylinder wake under a strong axial magnetic field. Phys. Fluids 20, 017104.Google Scholar
Dousset, V. & Pothérat, A. 2010 Formation mechanism of hairpin vortices in the wake of truncated square cylinder in a duct. J. Fluid Mech. 653, 519–536.CrossRefGoogle Scholar
Dousset, V. & Pothérat, A. 2012 Characterisation of the flow around a truncated cylinder in a duct in a spanwise magnetic field. J. Fluid Mech. 691, 341–367.Google Scholar
Frank, M., Barleon, L. & Müller, U. 2001 Visual analysis of two-dimensional magnetohydro- dynamics. Phys. Fluids 13 (8), 2287–2295.CrossRefGoogle Scholar
Greenspan, H. P. 1969 The Theory of Rotating Fluids. Cambridge University Press.Google Scholar
Hunt, J. C. R., Abell, C. J., Peterka, J. A. & Woo, H. 1978 Kinematical studies of the flows around free or surface-mounted mounted obstacles; applying topology to flow visualization. J. Fluid Mech. 86, 179–200.Google Scholar
Hunt, J. C. R. & Leibovich, S. 1967 Magnetohydrodynamic flow in channels of variable cross-section with strong transverse magnetic fields. J. Fluid Mech. 28, 241–260.CrossRefGoogle Scholar
Hunt, J. C. R. & Ludford, G. S. S. 1968 Three-dimensional MHD duct flows with strong transverse magnetic fields. Part 1. Obstacles in a constant area channel. J. Fluid Mech. 33, 693–714.CrossRefGoogle Scholar
Josserand, J., Marty, P. & Alémany, A. 1993 Pressure and drag measurements on a cylinder in a liquid metal flow with an aligned magnetic field. Fluid Dyn. Res. 11, 107–117.CrossRefGoogle Scholar
Kolesnikov, Yu. & Andreev, O. 1997 Heat transfer intensification promoted by vortical structures in a closed channel under magnetic field. J. Expl Therm. Fluid Sci. 15 (2), 82–90.Google Scholar
Kolesnikov, Yu. & Andreev, O. 2000 Two scaling flow around a cylinder in axial magnetic field. In The 4th International Conference: MHD at Dawn of 3rd Millennium, pp. 33–38. Giens.Google Scholar
Kolesnikov, Yu. B. & Tsinober, A. B. 1972 Two-dimensional turbulent flow behind a circular cylinder (experiment). Magnetohydrodynamics 8 (3), 300–307.Google Scholar
Lahjomri, J., Capéran, P. & Alémany, A. 1993 The cylinder wake in a magnetic field aligned with the velocity. J. Fluid Mech. 253, 421–448.Google Scholar
Ludford, G. S. S. 1961 The effect of a very strong magnetic cross-field on steady motion through a slightly conducting fluid. J. Fluid Mech. 10, 141–155.Google Scholar
Moreau, R. 1990 Magnetohydrodynamics. Kluwer.Google Scholar
Müller, U. & Bühler, L. 2010 Magnetofluiddynamics in Channels and Containers. Springer.Google Scholar
Mutschke, G., Gerbeth, G., Shatrov, V. & Tomboulides, A. 1997 Two- and three dimensional instabilities of the cylinder wake in an aligned magnetic field. Phys. Fluids 9 (11), 3114–3116.Google Scholar
Pothérat, A., Sommeria, J. & Moreau, R. 2000 An effective two-dimensional model for MHD flows with transverse magnetic field. J. Fluid Mech. 424, 75–100.CrossRefGoogle Scholar
Raffel, M., Willert, C. E. & Kompenhans, J. 1998 Particle Image Velocimetry. Springer.Google Scholar
Shercliff, J. A. 1953 Steady motion of conducting fluids in pipes under transverse magnetic fields. Proc. Camb. Phil. Soc. 49, 136–144.Google Scholar
Shercliff, J. A. 1962 The Theory of Electromagnetic Flow-measurement. Cambridge University Press.Google Scholar
Shercliff, J. A. 1975 Some duct flow problems at high Hartmann number. Z. Angew. Math. Phys. 26, 537–548.Google Scholar
Sommeria, J. & Moreau, R. 1982 Why, how, and when, MHD turbulence becomes two-dimensional. J. Fluid Mech. 118, 507–518.Google Scholar
Takizawa, Y, Matsuda, A., Sato, S., Abe, T. & Konigorski, D. 2006 Experimental investigation of the electromagnetic effect on a shock layer around a blunt body in a weakly ionized flow. Phys. Fluids 18, 117105.Google Scholar
Zaytsev, I. D. & Aseyev, G. G. 1992 Properties of Aqueous Solutions of Electrolytes. CRC.Google Scholar
Zdravkovich, M. M. 1997 Flow Around Circular Cylinders. Fundamentals, vol. 1, Oxford University Press.Google Scholar