Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-10T04:57:05.021Z Has data issue: false hasContentIssue false
  • English
  • Français

Water–propylene glycol sessile droplet shapes and migration: Marangoni mixing and separation of scales

Published online by Cambridge University Press:  06 January 2022

J. Charlier Open the ORCID record for J. Charlier [Opens in a new window] ,
A.Y. Rednikov Open the ORCID record for A.Y. Rednikov [Opens in a new window] ,
S. Dehaeck Open the ORCID record for S. Dehaeck [Opens in a new window] ,
P. Colinet Open the ORCID record for P. Colinet [Opens in a new window]  and
D. Terwagne Open the ORCID record for D. Terwagne [Opens in a new window]
J. Charlier
Affiliation:
Frugal Lab, Faculté des Sciences, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium FabLab ULB, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium TIPs Laboratory, Ecole polytechnique de Bruxelles, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium
A.Y. Rednikov*
Affiliation:
TIPs Laboratory, Ecole polytechnique de Bruxelles, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium
S. Dehaeck
Affiliation:
TIPs Laboratory, Ecole polytechnique de Bruxelles, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium
P. Colinet
Affiliation:
TIPs Laboratory, Ecole polytechnique de Bruxelles, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium
D. Terwagne
Affiliation:
Frugal Lab, Faculté des Sciences, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium FabLab ULB, Université libre de Bruxelles (ULB), B-1050 Brussels, Belgium
*
†Email address for correspondence: alexey.rednikov@ulb.be
Get access Rights & Permissions [Opens in a new window]

Abstract

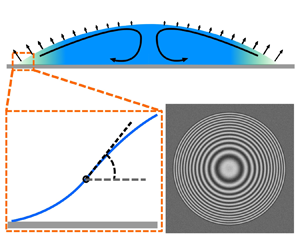
New light is shed on morphological features of water–propylene glycol sessile droplets evaporating into ambient air at not too high relative humidity. Such droplets adopt a Marangoni-contracted shape even on perfectly wetting substrates, an effect well known since Cira et al. (Nature, 519, 2015). We here highlight a strong separation of scales normally occurring for such droplets. Namely, there is a narrow high-curvature zone localized at the foot of the droplet, where the apparent contact angle is formed, while the core of the droplet merely adheres to the classical (capillary–gravity) static shape. Experimentally, we rely upon interferometry to discern such fine key details. We detect a maximum of the droplet slope profile in the foot region, which amounts to the apparent contact angle. Theoretically, a local description of the foot region is devised. We indicate a crucial role of convective mixing by the solutal Marangoni flow, here accounted for by the Taylor dispersion, which proves to underlie the separation of scales and ensure self-consistency of the local model. Migration of such droplets in a humidity gradient is also approached within the same experimental and theoretical framework. It is considered that the resulting back–front asymmetry of the apparent contact angles drives the motion similarly to a wettability gradient, although the drag (‘Cox–Voinov’) factor is here found to be different. The predictions, comparing well with the measurements (our own and from the literature), are based on rigorous models, isothermal and as reduced as possible, without any fitting parameters or microphysics effects.

JFM classification

Interfacial Flows (free surface): Contact lines Phase change: Condensation/evaporation Mass Transport: Coupled diffusion and flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 933 , 25 February 2022 , A45
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Allen, J.S. 2003 An analytical solution for determination of small contact angles from sessile drops of arbitrary size. J. Colloid Interface Sci. 261 (2), 481–489.CrossRefGoogle ScholarPubMed
Barmi, M.R. & Meinhart, C.D. 2014 Convective flows in evaporating sessile droplets. J. Phys. Chem. B 118 (9), 2414–2421.CrossRefGoogle ScholarPubMed
Benusiglio, A., Cira, N.J. & Prakash, M. 2018 Two-component Marangoni-contracted droplets: friction and shape. Soft Matt. 14 (37), 7724–7730.CrossRefGoogle ScholarPubMed
Brochard, F. 1989 Motions of droplets on solid surfaces induced by chemical or thermal gradients. Langmuir 5 (2), 432–438.CrossRefGoogle Scholar
Carles, P. & Cazabat, A.M. 1989 Spreading involving the Marangoni effect: some preliminary results. Colloids Surf. 41, 97–105.CrossRefGoogle Scholar
Charlier, J. 2020 Spreading and evaporation of drops in complex situations: lubricated rough surfaces, breaking of axi-symmetry, and multi-component droplets. PhD thesis, Université libre de Bruxelles.Google Scholar
Charlier, J., Rednikov, A., Dehaeck, S., Colinet, P. & Terwagne, D. 2019 Binary droplets walking with their feet. Bull. Am. Phys. Soc. 64 (2).Google Scholar
Cira, N.J., Benusiglio, A. & Prakash, M. 2015 Vapour-mediated sensing and motility in two-component droplets. Nature 519 (7544), 446–450.CrossRefGoogle ScholarPubMed
Colinet, P. & Rednikov, A. 2011 On integrable singularities and apparent contact angles within a classical paradigm. Partial and complete wetting regimes with or without phase change. Eur. Phys. J. Spec. Top. 197, 89–113.CrossRefGoogle Scholar
De Gennes, P.G., Brochard-Wyart, F. & Quèrè, D. 2004 Capillarity and Wetting Phenomena: Drops, Bubbles, Pearls, Waves. Springer.CrossRefGoogle Scholar
Dehaeck, S. & Colinet, P. 2016 Improving speed and precision of local frequency analysis using Gaussian ridge interpolation for wavelet and windowed Fourier ridge algorithms. Opt. Lasers Engng 77, 54–63.CrossRefGoogle Scholar
Dehaeck, S., Rednikov, A. & Colinet, P. 2014 Vapor-based interferometric measurement of local evaporation rate and interfacial temperature of evaporating droplets. Langmuir 30 (8), 2002–2008.CrossRefGoogle ScholarPubMed
Dehaeck, S., Tsoumpas, Y. & Colinet, P. 2015 Analyzing closed-fringe images using two-dimensional Fan wavelets. Appl. Opt. 54 (10), 2939–2952.CrossRefGoogle ScholarPubMed
Diddens, C., Li, Y. & Lohse, D. 2021 Competing Marangoni and Rayleigh convection in evaporating binary droplets. J. Fluid Mech. 914, A23.CrossRefGoogle Scholar
Dugas, V., Broutin, J. & Souteyrand, E. 2005 Droplet evaporation study applied to DNA chip manufacturing. Langmuir 21 (20), 9130–9136.CrossRefGoogle ScholarPubMed
Hack, M.A., Kwieciński, W., Ramírez-Soto, O., Segers, T., Karpitschka, S., Kooij, E.S. & Snoeijer, J.H. 2021 Wetting of two-component drops: Marangoni contraction versus autophobing. Langmuir 37 (12), 3605–3611.CrossRefGoogle ScholarPubMed
Hu, H. & Larson, R.G. 2005 Analysis of the effects of Marangoni stresses on the microflow in an evaporating sessile droplet. Langmuir 21 (9), 3972–3980.CrossRefGoogle Scholar
Jing, J., et al. 1998 Automated high resolution optical mapping using arrayed, fluid-fixed DNA molecules. Proc. Natl Acad. Sci. USA 95 (14), 8046–8051.CrossRefGoogle ScholarPubMed
Karpitschka, S., Liebig, F. & Riegler, H. 2017 Marangoni contraction of evaporating sessile droplets of binary mixtures. Langmuir 33 (19), 4682–4687.CrossRefGoogle ScholarPubMed
Karpitschka, S. & Riegler, H. 2010 Quantitative experimental study on the transition between fast and delayed coalescence of sessile droplets with different but completely miscible liquids. Langmuir 26 (14), 11823–11829.CrossRefGoogle Scholar
Keiser, L., Bense, H., Colinet, P., Bico, J. & Reyssat, E. 2017 Marangoni bursting: evaporation-induced emulsification of binary mixtures on a liquid layer. Phys. Rev. Lett. 118 (7), 074504.CrossRefGoogle ScholarPubMed
Khattab, I.S., Bandarkar, F., Khoubnasabjafari, M. & Jouyban, A. 2012 Density, viscosity, surface tension, and molar volume of propylene glycol + water mixtures from 293 to 323 K and correlations by the Jouyban–Acree model. Arab. J. Chem. 10, S71–S75.CrossRefGoogle Scholar
Kim, D., Jeong, S., Park, B.K. & Moon, J. 2006 Direct writing of silver conductive patterns: improvement of film morphology and conductance by controlling solvent compositions. Appl. Phys. Lett. 89 (26), 264101.CrossRefGoogle Scholar
Kim, H. & Stone, H.A. 2018 Direct measurement of selective evaporation of binary mixture droplets by dissolving materials. J. Fluid Mech. 850, 769–783.CrossRefGoogle Scholar
Kim, J. 2007 Spray cooling heat transfer: the state of the art. Intl J. Heat Fluid Flow 28 (4), 753–767.CrossRefGoogle Scholar
Lohse, D. & Zhang, X. 2020 Physicochemical hydrodynamics of droplets out of equilibrium. Nat. Rev. Phys. 2 (8), 426–443.CrossRefGoogle Scholar
Morris, S.J.S. 2014 On the contact region of a diffusion-limited evaporating drop: a local analysis. J. Fluid Mech. 739, 308–337.CrossRefGoogle Scholar
Parimalanathan, S.K., Dehaeck, S., Rednikov, A. & Colinet, P. 2021 Controlling the wetting and evaporation dynamics of non-ideal volatile binary solutions. J. Colloid Interface Sci. 592, 319–328.CrossRefGoogle ScholarPubMed
Pham, C.-T., Berteloot, G., Lequeux, F. & Limat, L. 2010 Dynamics of complete wetting liquid under evaporation. Europhys. Lett. 92, 54005.CrossRefGoogle Scholar
Popov, Y.O. 2005 Evaporative deposition patterns: spatial dimensions of the deposit. Phys. Rev. E 71, 036313.CrossRefGoogle ScholarPubMed
Poulard, C., Guéna, G., Cazabat, A.M., Boudaoud, A. & Ben Amar, M. 2005 Rescaling the dynamics of evaporating drops. Langmuir 21 (18), 8226–8233.CrossRefGoogle ScholarPubMed
Ramírez-Soto, O. & Karpitschka, S. 2021 Taylor dispersion governs the compositional evolution of Marangoni-contracted droplets. arXiv:2102.08727v2.Google Scholar
Rednikov, A.Y. & Colinet, P. 2019 Contact-line singularities resolved exclusively by the Kelvin effect: volatile liquids in air. J. Fluid Mech. 858, 881–916.CrossRefGoogle Scholar
Rednikov, A.Y. & Colinet, P. 2020 Contact angles for perfectly wetting pure liquids evaporating into air: between de Gennes-type and other classical models. Phys. Rev. Fluids 5, 114007.CrossRefGoogle Scholar
Sadafi, H., Dehaeck, S., Rednikov, A. & Colinet, P. 2019 Vapor-mediated versus substrate-mediated interactions between volatile droplets. Langmuir 35 (21), 7060–7065.CrossRefGoogle ScholarPubMed
Savino, R., Paterna, D. & Favaloro, N. 2002 Buoyancy and Marangoni effects in an evaporating drop. J. Thermophys. Heat Transfer 16 (4), 562–574.CrossRefGoogle Scholar
Singh, M., Haverinen, H.M., Dhagat, P. & Jabbour, G.E. 2010 Inkjet printing – process and its applications. Adv. Mater. 22 (6), 673–685.CrossRefGoogle ScholarPubMed
Siregar, D.P., Kuerten, J.G.M. & van der Geld, C.W.M. 2013 Numerical simulation of the drying of inkjet-printed droplets. J. Colloid Interface Sci. 392, 388–395.CrossRefGoogle ScholarPubMed
Taylor, G. 1953 Dispersion of soluble matter in solvent flowing slowly through a tube. Proc. R. Soc. Lond. A 219 (1137), 186–203.Google Scholar
Taylor, G. 1954 The dispersion of matter in turbulent flow through a pipe. Proc. R. Soc. Lond. A 223 (1155), 446–468.Google Scholar
Tekin, E., de Gans, B.J. & Schubert, U.S. 2004 Ink-jet printing of polymers–from single dots to thin film libraries. J. Mater. Chem. 14 (17), 2627–2632.CrossRefGoogle Scholar
Tsoumpas, Y., Dehaeck, S., Rednikov, A. & Colinet, P. 2015 Effect of Marangoni flows on the shape of thin sessile droplets evaporating into air. Langmuir 31 (49), 13334–13340.CrossRefGoogle ScholarPubMed
Van den Broeck, C. 1990 Taylor dispersion revisited. Physica A 168 (2), 677–696.CrossRefGoogle Scholar
Verlinde, J., Verbeeck, R. & Thun, H. 2010 Density and vapour pressure of the propylene glycol–water system from 15 to 50 $^\circ$C. Bull. Soc. Chim. Belg. 84, 1119–1130.CrossRefGoogle Scholar
Wang, M.H., Soriano, A.N., Caparanga, A.R. & Li, M.H. 2010 Binary mutual diffusion coefficient of aqueous solutions of propylene glycol and dipropylene glycol. J. Taiwan Inst. Chem. Engng 41 (3), 279–285.CrossRefGoogle Scholar
Xu, X. & Luo, J. 2007 Marangoni flow in an evaporating water droplet. Appl. Phys. Lett. 91 (12), 124102.CrossRefGoogle Scholar
Xu, Y., Zhang, N., Yang, W.J. & Vest, C.M. 1984 Optical measurements of profile and contact angle of liquids on transparent substrates. Exp. Fluids 2 (3), 142–144.CrossRefGoogle Scholar
Yakhno, T.A., Sedova, O.A., Sanin, A.G. & Pelyushenko, A.S. 2003 On the existence of regular structures in liquid human blood serum (plasma) and phase transitions in the course of its drying. Tech. Phys. 48 (4), 399–403.CrossRefGoogle Scholar