Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Johnson, F.E.A.
2011.
Infinite branching in the first syzygy.
Journal of Algebra,
Vol. 337,
Issue. 1,
p.
181.

 for any finite group
for any finite group 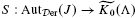 here
here 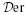 is the quotient of the category of Λ-homomorphisms obtained by setting ‘projective = 0’. We then employ it to give an exact classification of homotopy classes of extensions 0 →
is the quotient of the category of Λ-homomorphisms obtained by setting ‘projective = 0’. We then employ it to give an exact classification of homotopy classes of extensions 0 → 