Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-10T21:23:12.022Z Has data issue: false hasContentIssue false
  • English
  • Français

Dislocation mechanisms of radius effect on displacement bursts during spherical nanoindentations

Published online by Cambridge University Press:  12 June 2012

Chansun Shin  and
Sanghoon Shim
Chansun Shin*
Affiliation:
Nuclear Materials Research Division, Korea Atomic Energy Institute, Daejeon, 305-353, Korea
Sanghoon Shim
Affiliation:
Steel Structure Research Laboratory, Research Institute of Industrial Science and Technology, Gyunngi-do, 445-813, Korea
*
a)Address all correspondence to this author. e-mail: cshin@kaeri.re.kr
Get access

Abstract

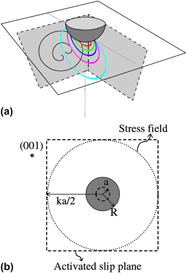
Indentation load–displacement curves for Mo (100) single crystals reveal clear displacement bursts from spherical indenters with various radii from ∼0.1 to ∼130 μm. There are two different size-dependent mechanisms for dislocation evolution involved during the displacement bursts. It has been postulated that these bursts are triggered by the nucleation of dislocations for a small indenter radius and the activation of preexisting dislocations for a large indenter radius. We present a simple model with which the displacement bursts from a larger indenter radius can be rationalized. This model relates the load and the excursion length during the first displacement burst. The correspondence between the model and experimental data indicates that the displacement bursts are initiated by the activation of preexisting dislocations and the model can accurately describe the mechanism for the displacement bursts from large indenters.

Type
Articles
Information
Journal of Materials Research , Volume 27 , Issue 16 , 28 August 2012 , pp. 2161 - 2166
Copyright
Copyright © Materials Research Society 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

1.Oliver, W.C. and Pharr, G.M.: Improved technique for determining hardness and elastic modulus using load and displacement sensing indentation experiments. J. Mater. Res. 7, 1564 (1992).CrossRefGoogle Scholar
2.Corcoran, S.G., Colton, R.J., Lilleodden, E.T., and Gerberich, W.W.: Anomalous plastic deformation at surfaces: Nanoindentation of gold single crystals. Phys. Rev. B 55, R16057 (1997).CrossRefGoogle Scholar
3.Schuh, C.A., Mason, J.K., and Lund, A.C.: Quantitative insight into dislocation nucleation from high-temperature nanoindentation experiments. Nat. Mater. 4, 617 (2005).CrossRefGoogle ScholarPubMed
4.Cha, P-R., Srolovitz, D.J., and Vanderlick, T.K.: Molecular dynamics simulation of single asperity contact. Acta Mater. 52, 3983 (2004).CrossRefGoogle Scholar
5.Van Vliet, K.J., Li, J., Zhu, T., Yip, S., and Suresh, S.: Quantifying the early stages of plasticity through nanoscale experiments and simulations. Phys. Rev. B 67, 104105 (2003).CrossRefGoogle Scholar
6.Gouldstone, A., Van Vliet, K.J., and Suresh, S.: Nanoindentation: Simulation of defect nucleation in a crystal. Nature 411, 656 (2001).CrossRefGoogle ScholarPubMed
7.Shim, S., Bei, H., George, E.P., and Pharr, G.M.: A different type of indentation size effect. Scr. Mater. 59, 1095 (2008).CrossRefGoogle Scholar
8.Gao, H., Huang, Y., Nix, W.D., and Hutchinson, J.W.: Mechanism-based strain gradient plasticity -I. J. Mech. Phys. Solids 47, 1239 (1999).CrossRefGoogle Scholar
9.Qu, S., Huang, Y., Pharr, G.M., and Hwang, K.C.: The indentation size effect in the spherical indentation of iridium: A study via the conventional theory of mechanism-based strain gradient plasticity. Int. J. Plast. 22, 1265 (2006).CrossRefGoogle Scholar
10.Gerberich, W.W., Venkataraman, S.K., Huang, H., Harvey, S.E., and Kohlstedt, D.L.: The injection of plasticity by millinewton contacts. Acta Metall. Mater. 43, 1569 (1995).CrossRefGoogle Scholar
11.Gerberich, W.W., Nelson, J.C., Lilleodden, E.T., Anderson, P., and Wyrobek, J.T.: Indentation induced dislocation nucleation: The initial yield point. Acta Mater. 44, 3585 (1996).CrossRefGoogle Scholar
12.Bahr, D.F., Kramer, D.E., and Gerberich, W.W.: Non-linear deformation mechanisms during nanoindentation. Acta Mater. 46, 4605 (1998).CrossRefGoogle Scholar
13.Gerberich, W.W., Karmer, D.E., Tymiak, N.I., Volinsky, A.A., Bahr, D.F., and Kriese, M.D.: Nanoindentation-induced defect-interface interactions: Phenomena, methods and limitations. Acta Mater. 47, 4115 (1999).CrossRefGoogle Scholar
14.Gouldstone, A., Koh, H-J., Zeng, K-Y., Giannakopoulos, A.E., and Suresh, S.: Discrete and continuous deformation during nanoindentation of thin films. Acta Mater. 48, 2277 (2000).CrossRefGoogle Scholar
15.Gerberich, W.W., Mook, W.M., Chambers, M.D., Cordill, M.J., Perrey, C.R., Carter, C.B., Miller, R.E., Curtin, W.A., Mukherjee, R., and Girshick, S.L.: An energy balance criterion for nanoindentation-induced single and multiple dislocation events. J. Appl. Mech. 73, 327 (2006).CrossRefGoogle Scholar
16.Shibutani, Y., Tsuru, T., and Koyama, A.: Nanoplastic deformation of nanoindentation: Crystallographic dependence of displacement bursts. Acta Mater. 55, 1813 (2007).CrossRefGoogle Scholar
17.Bei, H., Gao, Y.F., Shim, S., George, E.P., and Pharr, G.M.: Strength differences arising from homogeneous versus heterogeneous dislocation nucleation. Phys. Rev. B 77, 060103 (2008).CrossRefGoogle Scholar
18.Lorenz, D., Zeckzer, A., Hilpert, U., and Grau, P.: Pop-in effect as homogeneous nucleation of dislocations during nanoindentation. Phys. Rev. B 67, 172101 (2003).CrossRefGoogle Scholar
19.Morris, J.R., Bei, H., Pharr, G.M., and George, E.P.: Size effects and stochastic behavior of nanoindentation pop in. Phys. Rev. Lett. 106, 165502 (2011).CrossRefGoogle ScholarPubMed
20.Johnson, K.L.: Contact Mechanics (Cambridge University Press, Cambridge, 1985).CrossRefGoogle Scholar
21.Simmons, G. and Wang, H.: Single Crystal Elastic Constants and Calculated Aggregate Properties – A Handbook (MIT Press, Cambridge, MA, 1971).Google Scholar
22.Ogata, S., Li, J., Hirosaki, N., Shibutani, Y., and Yip, S.: Ideal shear strain of metals and ceramics. Phys. Rev. B 70, 104104 (2004).CrossRefGoogle Scholar
23.Bei, H., Shim, S., Pharr, G.M., and George, E.P.: Effects of pre-strain on the compressive stress-strain response of Mo-alloy single-crystal micropillars. Acta Mater. 56, 4762 (2008).CrossRefGoogle Scholar
24.Dimiduk, D.M., Uchic, M.D., and Parthasarathy, T.A.: Size-affected single-slip behavior of nickel microcrystals. Acta Mater. 53, 4065 (2005).CrossRefGoogle Scholar
25.Zhou, C., Biner, S.B., and LeSar, R.: Discrete dislocation dynamics simulations of plasticity at small scales. Acta Mater. 58, 1565 (2010).CrossRefGoogle Scholar
26.Senger, J., Weygand, D., Gumbsch, P., and Kraft, O.: Discrete dislocation simulations of the plasticity of micro-pillars under uniaxial loading. Scr. Mater. 58, 587 (2008).CrossRefGoogle Scholar
27.Johnson, K.L.: The correlation of indentation experiments. J. Mech. Phys. Solids 18, 115 (1970).CrossRefGoogle Scholar
28.Bei, H., Lu, Z.P., and George, E.P.: Theoretical strength and the onset of plasticity in bulk metallic glasses investigated by nanoindentation with a spherical indenter. Phys. Rev. Lett. 93, 125511 (2004).CrossRefGoogle ScholarPubMed
29.Zielinski, W., Huang, H., and Gerberich, W.W.: Microscopy and microindentation mechanics of single crystal Fe-3 wt.%Si: Part II. TEM of the indentation plastic zone. J. Mater. Res. 8, 1300 (1993).CrossRefGoogle Scholar
30.Shan, Z.W., Mishra, R.K., Syed Asif, S.A., Warren, O.L., and Minor, A.M.: Mechanical annealing and source-limited deformation in submicrometre-diameter Ni crystals. Nat. Mater. 7, 115 (2008).CrossRefGoogle ScholarPubMed
31.Guiu, F. and Pratt, P.L.: The effect of orientation on the yielding and flow of molybdenum single crystals. Phys. Status Solidi B 15, 539 (1966).CrossRefGoogle Scholar
32.Bei, H., Shim, S., George, E.P., Miller, M.K., Herbert, E.G., and Pharr, G.M.: Compressive strengths of molybdenum alloy micro-pillars prepared using a new technique. Scr. Mater. 57, 397 (2007).CrossRefGoogle Scholar
33.Swain, M.V. and Lawn, B.R.: A study of dislocation arrays at spherical indentations in LiF as a function of indentation stress and strain. Phys. Status Solidi 35, 909 (1969).CrossRefGoogle Scholar