Published online by Cambridge University Press: 29 April 2019
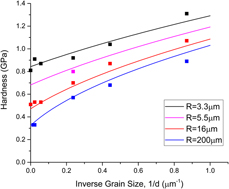
The measured hardness of a metal crystal depends on a variety of length scales. Microstructural features, such as grain size and precipitate spacing, determine the intrinsic material length scale. Extrinsic (test) length scales, such as the indentation depth, lead to the indentation size effect (ISE), whereby it is typically found that smaller is stronger. Nix and Gao [J. Mech. Phys. Solids46, 411 (1998)] developed a widely used model for interpreting the ISE based on forest hardening in single crystalline pure metals. This work extends that model to consider the hardness of polycrystals and alloys, as well as introducing a finite limit to the hardness at very small extrinsic length scales. The resulting expressions are validated against data from the literature. It is shown that a reasonable estimate of the intrinsic material length scale can be extracted from a suite of hardness tests conducted across a range of indentation depths using spherical indenters of various radii.