Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ribbing, Carl G.
and
Wäckelgård, Ewa
1991.
Reststrahlen bands as property indicators for materials in dielectric coatings.
Thin Solid Films,
Vol. 206,
Issue. 1-2,
p.
312.
van Delft, F.C.M.J.M.
1991.
Bulk and surface Debye temperatures in relation to cohesive energy and Lennard-Jones potentials.
Surface Science,
Vol. 251-252,
Issue. ,
p.
690.
Rosenmayer, C.T.
Brotzen, F.R.
McPherson, J.W.
and
Dunn, C.F.
1991.
Effect of stresses on electromigration.
p.
52.
Zimmerman, M.H
Baskin, D.M
Faber, K.T
Fuller, E.R
Allen, A.J
and
Keane, D.T
2001.
Fracture of a textured anisotropic ceramic.
Acta Materialia,
Vol. 49,
Issue. 16,
p.
3231.
Sree Harsha, K.S.
2006.
Principles of Vapor Deposition of Thin Films.
p.
685.
Zhu, Y. F.
Zheng, W. T.
and
Jiang, Q.
2009.
Modeling lattice expansion and cohesive energy of nanostructured materials.
Applied Physics Letters,
Vol. 95,
Issue. 8,
Sorace, C.M.
Louge, M.Y.
Crozier, M.D.
and
Law, V.H.C.
2009.
High apparent adhesion energy in the breakdown of normal restitution for binary impacts of small spheres at low speed.
Mechanics Research Communications,
Vol. 36,
Issue. 3,
p.
364.
Zhu, Y. F.
Lian, J. S.
and
Jiang, Q.
2009.
Modeling of the Melting Point, Debye Temperature, Thermal Expansion Coefficient, and the Specific Heat of Nanostructured Materials.
The Journal of Physical Chemistry C,
Vol. 113,
Issue. 39,
p.
16896.
Huang, Congliang
and
Lan, Yingying
2021.
Thermophysical study of surface phonon polaritons in multilayer systems for heat dissipation.
International Journal of Thermal Sciences,
Vol. 159,
Issue. ,
p.
106548.
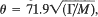 , where M is the atomic weight. This relation is derived theoretically in the Debye isotropic approximation by assuming that the interatomic potential is central. No restrictions are imposed on the range of the potential. The relation is obeyed very well by the observed values of θ and Γ in the case of many solids.
, where M is the atomic weight. This relation is derived theoretically in the Debye isotropic approximation by assuming that the interatomic potential is central. No restrictions are imposed on the range of the potential. The relation is obeyed very well by the observed values of θ and Γ in the case of many solids.