Published online by Cambridge University Press: 17 June 2019
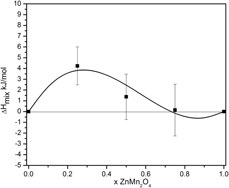
The thermodynamic properties of ZnxMn3−xO4 and Mg1−zCuzCr2O4 spinel solid solutions have been studied using high-temperature oxide melt solution calorimetry. Except for MgCr2O4 spinel, which possesses cubic structure, the other three end-members are tetragonal. The enthalpies of mixing are small endothermic and fit subregular solution behavior. The main contribution to the energetics of mixing of both spinel systems comes from the difference in the crystal structure between the end-members: a change in the tetragonal distortion for ZnxMn3−xO4 solid solutions and a transition from cubic to tetragonal for the Mg1−zCuzCr2O4 system. If all Mg1−zCuzCr2O4 spinels possessed the same structure, the mixing enthalpies would be close to zero. Because both series have normal cation distributions, the entropies of mixing are equal to the configurational entropies of mixing of Zn2+ and Mn2+ and of Mg2+ and Cu2+ on tetrahedral sites, and the activities would follow Raoult’s law. The calculated Gibbs energy of mixing confirms the absence of solvus at any temperature for both systems.
Present address: Materials Science and Engineering Department, University of Illinois, Urbana, Illinois 61801, USA.
This author was an editor of this journal during the review and decision stage. For the JMR policy on review and publication of manuscripts authored by editors, please refer to http://www.mrs.org/editor-manuscripts/.