Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ball, Justin
and
Brunner, Stephan
2021.
A non-twisting flux tube for local gyrokinetic simulations.
Plasma Physics and Controlled Fusion,
Vol. 63,
Issue. 6,
p.
064008.
C. J., Ajay
Brunner, Stephan
and
Ball, Justin
2021.
Effect of collisions on non-adiabatic electron dynamics in ITG-driven microturbulence.
Physics of Plasmas,
Vol. 28,
Issue. 9,
Hardman, M R
Parra, F I
Chong, C
Adkins, T
Anastopoulos-Tzanis, M S
Barnes, M
Dickinson, D
Parisi, J F
and
Wilson, H
2022.
Extended electron tails in electrostatic microinstabilities and the nonadiabatic response of passing electrons.
Plasma Physics and Controlled Fusion,
Vol. 64,
Issue. 5,
p.
055004.
Wang, Zihao
Dai, Zongliang
and
Wang, Shaojie
2022.
Nonlinear excitation of zonal flows by turbulent energy flux.
Physical Review E,
Vol. 106,
Issue. 3,
Hegna, C.C.
Anderson, D.T.
Bader, A.
Bechtel, T.A.
Bhattacharjee, A.
Cole, M.
Drevlak, M.
Duff, J.M.
Faber, B.J.
Hudson, S.R.
Kotschenreuther, M.
Kruger, T.G.
Landreman, M.
McKinney, I.J.
Paul, E.
Pueschel, M.J.
Schmitt, J.S.
Terry, P.W.
Ware, A.S.
Zarnstorff, M.
and
Zhu, C.
2022.
Improving the stellarator through advances in plasma theory.
Nuclear Fusion,
Vol. 62,
Issue. 4,
p.
042012.
Dif-Pradalier, Guilhem
Ghendrih, Philippe
Sarazin, Yanick
Caschera, Elisabetta
Clairet, Frédéric
Camenen, Yann
Donnel, Peter
Garbet, Xavier
Grandgirard, Virginie
Munschy, Yann
Vermare, Laure
and
Widmer, Fabien
2022.
Transport barrier onset and edge turbulence shortfall in fusion plasmas.
Communications Physics,
Vol. 5,
Issue. 1,
Mazzi, S.
Camenen, Y.
Garcia, J.
Zarzoso, D.
Frigione, D.
Garzotti, L.
Rimini, F.
Van Eester, D.
and
JET Contributors
2022.
Effects of the parallel flow shear on the ITG-driven turbulent transport in tokamak plasmas.
Nuclear Fusion,
Vol. 62,
Issue. 9,
p.
096024.
Volčokas, Arnas
Ball, Justin
and
Brunner, Stephan
2023.
Ultra long turbulent eddies, magnetic topology, and the triggering of internal transport barriers in tokamaks.
Nuclear Fusion,
Vol. 63,
Issue. 1,
p.
014003.
St-Onge, D A
Barnes, M
and
Parra, F I
2023.
A phase-shift-periodic parallel boundary condition for low-magnetic-shear scenarios.
Plasma Physics and Controlled Fusion,
Vol. 65,
Issue. 1,
p.
015016.
C.J., Ajay
McMillan, B.F.
and
Pueschel, M.J.
2023.
On the impact of temperature gradient flattening and system size on heat transport in microtearing turbulence.
Nuclear Fusion,
Vol. 63,
Issue. 6,
p.
066024.
Mariani, A
Brunner, S
Merlo, G
and
Sauter, O
2023.
Global ‘zero particle flux-driven’ gyrokinetic analysis of the density profile for a TCV plasma.
Plasma Physics and Controlled Fusion,
Vol. 65,
Issue. 5,
p.
054005.
Sun, Haomin
Ball, Justin
Brunner, Stephan
and
Volčokas, Arnas
2024.
A new quasilinear model for turbulent momentum transport in tokamaks with flow shear and plasma shaping.
Nuclear Fusion,
Vol. 64,
Issue. 3,
p.
036026.
Singh, Amit K.
Choudhary, S.
Gopal Krishna, M.
Mahapatra, J.
Bokshi, A.
Chowdhury, J.
Ganesh, R.
Hayward-Schneider, T.
Lanti, E.
Mishchenko, A.
McMillan, B.F.
and
Villard, L.
2024.
Magnetic shaping effects on turbulence in ADITYA-U tokamak.
Nuclear Fusion,
Vol. 64,
Issue. 10,
p.
106005.
Ajay, C. J.
McMillan, Ben
Bokshi, Arkaprava
di Siena, Alessandro
Pueschel, M. J.
and
Ruiz, Juan Ruiz
2024.
Gyrokinetic investigation of toroidal Alfvén eigenmode (TAE) turbulence.
AIP Advances,
Vol. 14,
Issue. 7,
Volčokas, Arnas
Ball, Justin
and
Brunner, Stephan
2025.
Numerical study of turbulent eddy self-interaction in tokamaks with low magnetic shear. Part I: Linear simulations.
Plasma Physics and Controlled Fusion,
Vol. 67,
Issue. 1,
p.
015001.
Volčokas, Arnas
Ball, Justin
and
Brunner, Stephan
2025.
Numerical study of turbulent eddy self-interaction in tokamaks with low magnetic shear. Part II: Nonlinear simulations.
Plasma Physics and Controlled Fusion,
Vol. 67,
Issue. 1,
p.
015002.
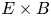 $E\times B$ zonal flow shear layers at radial locations near low-order mode rational surfaces (Weikl et al. Phys. Plasmas, vol. 25, 2018, 072305). We find that self-interaction, in fact, plays a very significant role in also generating fluctuating zonal flows, which is critical to regulating turbulent transport throughout the radial extent. Unlike the usual picture of zonal flow drive in which microinstability eigenmodes coherently amplify the flow via modulational instabilities, the self-interaction drive of zonal flows from these eigenmodes are uncorrelated with each other. It is shown that the associated shearing rate of the fluctuating zonal flows therefore reduces as more toroidal modes are resolved in the simulation. In simulations accounting for the full toroidal domain, such an increase in the density of toroidal modes corresponds to an increase in the toroidal system size, leading to a finite system size effect that is distinct from the well-known profile shearing effect.
$E\times B$ zonal flow shear layers at radial locations near low-order mode rational surfaces (Weikl et al. Phys. Plasmas, vol. 25, 2018, 072305). We find that self-interaction, in fact, plays a very significant role in also generating fluctuating zonal flows, which is critical to regulating turbulent transport throughout the radial extent. Unlike the usual picture of zonal flow drive in which microinstability eigenmodes coherently amplify the flow via modulational instabilities, the self-interaction drive of zonal flows from these eigenmodes are uncorrelated with each other. It is shown that the associated shearing rate of the fluctuating zonal flows therefore reduces as more toroidal modes are resolved in the simulation. In simulations accounting for the full toroidal domain, such an increase in the density of toroidal modes corresponds to an increase in the toroidal system size, leading to a finite system size effect that is distinct from the well-known profile shearing effect.

