Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-10T17:16:49.291Z Has data issue: false hasContentIssue false
  • English
  • Français

Toward laboratory torsional spine magnetic reconnection

Published online by Cambridge University Press:  06 November 2017

David L. Chesny Open the ORCID record for David L. Chesny [Opens in a new window] ,
N. Brice Orange ,
Hakeem M. Oluseyi  and
David R. Valletta
David L. Chesny*
Affiliation:
OrangeWave Innovative Science, LLC, Moncks Corner, SC, 29461, USA
N. Brice Orange
Affiliation:
OrangeWave Innovative Science, LLC, Moncks Corner, SC, 29461, USA
Hakeem M. Oluseyi
Affiliation:
Florida Institute of Technology, Department of Physics and Space Sciences, Melbourne, FL,  32901, USA
David R. Valletta
Affiliation:
OrangeWave Innovative Science, LLC, Moncks Corner, SC, 29461, USA
*
†Email address for correspondence: orangewavedc@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

Magnetic reconnection is a fundamental energy conversion mechanism in nature. Major attempts to study this process in controlled settings on Earth have largely been limited to reproducing approximately two-dimensional (2-D) reconnection dynamics. Other experiments describing reconnection near three-dimensional null points are non-driven, and do not induce any of the 3-D modes of spine fan, torsional fan or torsional spine reconnection. In order to study these important 3-D modes observed in astrophysical plasmas (e.g. the solar atmosphere), laboratory set-ups must be designed to induce driven reconnection about an isolated magnetic null point. As such, we consider the limited range of fundamental resistive magnetohydrodynamic (MHD) and kinetic parameters of dynamic laboratory plasmas that are necessary to induce the torsional spine reconnection (TSR) mode characterized by a driven rotational slippage of field lines – a feature that has yet to be achieved in operational laboratory magnetic reconnection experiments. Leveraging existing reconnection models, we show that within a 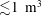${\lesssim}1~\text{m}^{3}$ apparatus, TSR can be achieved in dense plasma regimes (${\sim}10^{24}~\text{m}^{-3}$) in magnetic fields of 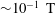${\sim}10^{-1}~\text{T}$. We find that MHD and kinetic parameters predict reconnection in thin ${\lesssim}20~\unicode[STIX]{x03BC}\text{m}$ current sheets on time scales of 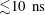${\lesssim}10~\text{ns}$. While these plasma regimes may not explicitly replicate the plasma parameters of observed astrophysical phenomena, studying the dynamics of the TSR mode within achievable set-ups signifies an important step in understanding the fundamentals of driven 3-D magnetic reconnection and the self-organization of current sheets. Explicit control of this reconnection mode may have implications for understanding particle acceleration in astrophysical environments, and may even have practical applications to fields such as spacecraft propulsion.

Keywords

plasma applicationsplasma devicesplasma sheaths
Type
Research Article
Information
Journal of Plasma Physics , Volume 83 , Issue 6 , December 2017 , 905830602
Copyright
© Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdou, A. E., Ismail, M. I., Mohamed, A. E., Lee, S., Saw, S. H. & Verma, R. 2012 Preliminary results of Kansas State University dense plasma focus. IEEE Trans. Plasma Sci. 40, 2741–2744.Google Scholar
Bak, P., Tang, C. & Wiesenfeld, K. 1987 Self-organized criticality – an explanation of 1/f noise. Phys. Rev. Lett. 59, 381–384.CrossRefGoogle Scholar
Biskamp, D. 1986 Magnetic reconnection via current sheets. Phys. Fluids 29, 1520–1531.CrossRefGoogle Scholar
Brown, M. R., Cothran, C. D. & Fung, J. 2006 Two fluid effects on three-dimensional reconnection in the Swarthmore Spheromak Experiment with comparisons to space data. Phys. Plasmas 13 (5), 056503.Google Scholar
Büchner, J. 1999 Three-dimensional magnetic reconnection in astrophysical plasmas – kinetic approach. Astrophys. Space Sci. 264, 25–42.Google Scholar
Bures, B. L., Krishnan, M. & James, C. 2012 A plasma focus electronic neutron generator. IEEE Trans. Plasma Sci. 40, 1082–1088.CrossRefGoogle Scholar
Bures, B. L., Krishnan, M., Madden, R. E. & Blobner, F. 2010 Enhancing neutron emission from a 500-J plasma focus by altering the anode geometry and gas composition. IEEE Trans. Plasma Sci. 38, 667–671.Google Scholar
Caballero Bendixsen, L. S., Bott-Suzuki, S. C., Cordaro, S. W., Krishnan, M., Chapman, S., Coleman, P. & Chittenden, J. 2016 Axial mass fraction measurements in a 300 kA dense plasma focus. Phys. Plasmas 23 (9), 093112.Google Scholar
Cazzola, E., Curreli, D., Markidis, S. & Lapenta, G. 2016 On the ions acceleration via collisionless magnetic reconnection in laboratory plasmas. Phys. Plasmas 23 (11), 112108.Google Scholar
Chang, T. 1992 Low-dimensional behavior and symmetry breaking of stochastic systems near criticality - can these effects be observed in space and in the laboratory? IEEE Trans. Plasma Sci. 20, 691–694.CrossRefGoogle Scholar
Chang, T. 1999 Self-organized criticality, multi-fractal spectra, sporadic localized reconnections and intermittent turbulence in the magnetotail. Phys. Plasmas 6, 4137–4145.Google Scholar
Chesny, D.2013 Non-potential magnetic fields and magnetic reconnection in low collisional plasmas: discovery of solar EUV mini-sigmoids and development of novel in-space propulsion systems. PhD thesis, Florida Institute of Technology.Google Scholar
Chesny, D. L., Oluseyi, H. M. & Orange, N. B. 2016 Dynamic flaring non-potential fields on quiet sun network scales. Astrophys. J. 822, 72.CrossRefGoogle Scholar
Chesny, D. L., Oluseyi, H. M., Orange, N. B. & Champey, P. R. 2015 Quiet-sun network bright point phenomena with sigmoidal signatures. Astrophys. J. 814, 124.Google Scholar
Dahlin, J. T., Drake, J. F. & Swisdak, M. 2014 The mechanisms of electron heating and acceleration during magnetic reconnection. Phys. Plasmas 21 (9), 092304.Google Scholar
Dungey, J. W. 1953 Lxxvi. conditions for the occurrence of electrical discharges in astrophysical systems. Lond. Edin. Dublin Phil. Mag. J. Sci. 44 (354), 725–738.Google Scholar
Edwards, S. J. & Parnell, C. E. 2015 Null point distribution in global coronal potential field extrapolations. Solar Phys. 290, 2055–2076.Google Scholar
Egedal, J., Fasoli, A., Porkolab, M. & Tarkowski, D. 2000 Plasma generation and confinement in a toroidal magnetic cusp. Rev. Sci. Instrum. 71, 3351–3361.CrossRefGoogle Scholar
Egedal, J., Fasoli, A., Tarkowski, D. & Scarabosio, A. 2001 Collisionless magnetic reconnection in a toroidal cusp. Phys. Plasmas 8, 1935–1943.Google Scholar
Egedal, J., Fox, W., Katz, N., Porkolab, M., Reim, K. & Zhang, E. 2007 Laboratory observations of spontaneous magnetic reconnection. Phys. Rev. Lett. 98 (1), 015003.CrossRefGoogle ScholarPubMed
El-Aragi, G. M. 2010 Ion beam emission within a low energy focus plasma (0.1 kJ) operating with hydrogen. Z. Naturforsch. A 65, 606–612.Google Scholar
Filevich, J., Rocca, E., Hammarsten, E., Jankowska, M., Marconi, R., Smith, R., Keenan, J., Dunn, S., Moon, V., Shlyaptsev, J. et al. 2003 Interferometric studies of laser-created plasmas using compact soft x-ray lasers. Proc. SPIE 5197, 5197–5197–12.Google Scholar
Filevich, J., Rocca, J. J., Marconi, M. C., Smith, R. F., Dunn, J., Keenan, R., Hunter, J. R., Moon, S. J., Nilsen, J., Ng, A. et al. 2004 Picosecond-resolution soft-x-ray laser plasma interferometry. Appl. Opt. 43, 3938–3946.CrossRefGoogle ScholarPubMed
Frank, A. G., Bogdanov, S. Y., Markov, V. S., Ostrovskaya, G. V. & Dreiden, G. V. 2005 Experimental study of plasma compression into the sheet in three-dimensional magnetic fields with singular X lines. Phys. Plasmas 12 (5), 052316.Google Scholar
Freed, M. S., Longcope, D. W. & McKenzie, D. E. 2015 Three-year global survey of coronal null points from potential-field-source-surface (PFSS) modeling and solar dynamics observatory (SDO) observations. Solar Phys. 290, 467–490.Google Scholar
Fuselier, S. A., Lewis, W. S., Schiff, C., Ergun, R., Burch, J. L., Petrinec, S. M. & Trattner, K. J. 2016 Magnetospheric multiscale science mission profile and operations. Space Sci. Rev. 199, 77–103.Google Scholar
Galsgaard, K., Priest, E. R. & Titov, V. S. 2003 Numerical experiments on wave propagation towards a 3D null point due to rotational motions. J. Geophys. Res. 108, 1042.Google Scholar
Gonzalez, W. & Parker, E. 2016 Magnetic reconnection. Magnetic Reconnection: Concepts and Applications 427, 101–142.Google Scholar
Goodzeit, C. L., Meinke, R. B. & Ball, M.2005 Concentric tilted double-helix dipoles and higher-order multipole magnets. UNITED STATES PATENT US 6921042 B1, 10/067,487.Google Scholar
Grasso, D., Lazzaro, E., Borgogno, D. & Comisso, L. 2016 Open problems of magnetic island control by electron cyclotron current drive. J. Plasma Phys. 82 (6), 595820603.CrossRefGoogle Scholar
Grasso, D., Margheriti, L., Porcelli, F. & Tebaldi, C. 2006 Letter to the Editor: Magnetic islands and spontaneous generation of zonal flows. Plasma Phys. Control. Fusion 48, L87–L95.Google Scholar
Grava, J., Purvis, M. A., Filevich, J., Marconi, M. C., Rocca, J. J., Dunn, J., Moon, S. J. & Shlyaptsev, V. N. 2008 Dynamics of a dense laboratory plasma jet investigated using soft x-ray laser interferometry. Phys. Rev. E 78, 016403.Google ScholarPubMed
Guillory, J., Rose, D. V. & Lerner, E. J. 2009 Theory of electron current filamentation instability and ion density filamentation in the early development of a DPF discharge. In American Institute of Physics Conference Series (ed. Kusse, B. R. & Hammer, D. A.), American Institute of Physics Conference Series, vol. 1088, pp. 203–206. American Institute of Physics.Google Scholar
Hart, P. J. 1964 Modified snowplow model for coaxial plasma accelerators. J. Appl. Phys. 35, 3425–3431.Google Scholar
Heo, H. & Park, D. K. 2002 Measurement of argon ion beam and x-ray energies in a plasma focus discharge. Phys. Scr. 65, 350–355.Google Scholar
Hosseinpour, M. 2014 Test particle acceleration in torsional spine magnetic reconnection. Astrophys. Space Sci. 353, 379–387.Google Scholar
Hosseinpour, M., Mehdizade, M. & Mohammadi, M. A. 2014 Comparison of test particle acceleration in torsional spine and fan reconnection regimes. Phys. Plasmas 21 (10), 102904.Google Scholar
Huba, J. D.2016 NRL plasma formulary. In Naval Res. Lab., p. 71. U.S. Naval Research Laboratory.Google Scholar
Imshennik, V. S. & Syrovatskiǐ, S. I. 1967 Two-dimensional flow of an ideally conducting gas in the vicinity of the zero line of a magnetic field. Sov. J. Exp. and Theor. Phys. 25, 656.Google Scholar
Ji, H., Bhattacharjee, A., Prager, S., Daughton, W. S., Bale, S. D., Carter, T. A., Crocker, N., Drake, J. F., Egedal, J., Sarff, J. et al. 2014 FLARE (Facility for Laboratory Reconnection Experiments): a major next-step for laboratory studies of magnetic reconnection. In AGU Fall Meeting Abstracts, American Geophysical Union (AGU).Google Scholar
Ji, H., Yamada, M., Hsu, S. & Kulsrud, R. 1998 Experimental test of the sweet-parker model of magnetic reconnection. Phys. Rev. Lett. 80, 3256–3259.Google Scholar
Kallenrode, M.-B. 2004 Space Physics: An Introduction to Plasmas and Particles in the Heliosphere and Magnetospheres, 3rd edn. Springer.Google Scholar
Karlický, M. 2014 Solar flares: radio and X-ray signatures of magnetic reconnection processes. Research in Astronomy and Astrophysics 14, 753–772.Google Scholar
Kivelson, M. G. & Russell, C. T. 1995 Introduction to Space Physics Cambridge University Press.Google Scholar
Krishnan, M. & Thompson, J.2010 Dense Plasma Focus Apparatus. UNITED STATES PATENT US 7,679025 B1, 11/057,040.Google Scholar
Lee, S. 1983 An energy-consistent snow-plough model for pinch design. J. Phys. D 16, 2463–2469.Google Scholar
Lee, S. & Serban, A. 1996 Dimensions and lifetime of the plasma focus pinch. IEEE Trans. Plasma Sci. 24, 1101–1105.Google Scholar
Lerner, E. J., Krupakar Murali, S. & Haboub, A. 2011 Theory and experimental program for p-B $^{11}$ fusion with the dense plasma focus. J. Fusion Energy 30, 367–376.Google Scholar
Lindberg, L. & Jacobsen, C. 1961 On the amplification of the poloidal magnetic flux in a plasma. Astrophys. J. 133, 1043.Google Scholar
Longcope, D. W. & Parnell, C. E. 2009 The number of magnetic null points in the quiet sun corona. Solar Phys. 254, 51–75.CrossRefGoogle Scholar
Meinke, R., Senti, M. & Stelzer, G. 1997 Novel design of superconducting helical dipole magnet. In APS Meeting Abstracts, American Physical Society.Google Scholar
Mitrofanov, K. N., Krauz, V. I., Kubes, P., Scholz, M., Paduch, M. & Zielinska, E. 2014 Study of the fine structure of the plasma current sheath and magnetic fields in the axial region of the PF-1000 facility. Plasma Phys. Rep. 40, 623–639.Google Scholar
Nalewajko, K. 2016 Applying relativistic reconnection to blazar jets. Galaxies 4, 28.Google Scholar
Ngom, B. B., Smith, T. B., Huang, W. & Gallimore, A. D. 2008 Numerical simulation of the Zeeman effect in neutral xenon from NIR diode-laser spectroscopy. J. Appl. Phys. 104 (2), 023303–023303–14.Google Scholar
Nishizuka, N., Hayashi, Y., Tanabe, H., Kuwahata, A., Kaminou, Y., Ono, Y., Inomoto, M. & Shimizu, T. 2012 A laboratory experiment of magnetic reconnection: outflows, heating, and waves in chromospheric jets. Astrophys. J. 756, 152.Google Scholar
Noonan, W. A., Jones, T. G. & Ottinger, P. F. 1997 Laser induced fluorescence diagnostic for measuring small magnetic fields. Rev. Sci. Instrum. 68 (1), 1032–1035.Google Scholar
Olshevsky, V., Divin, A., Eriksson, E., Markidis, S. & Lapenta, G. 2015 Energy dissipation in magnetic null points at kinetic scales. Astrophys. J. 807, 155.Google Scholar
Ono, Y., Morita, A., Katsurai, M. & Yamada, M. 1993 Experimental investigation of three-dimensional magnetic reconnection by use of two colliding spheromaks. Phys. Fluids B 5, 3691–3701.Google Scholar
Parnell, C. E., Neukirch, T., Smith, J. M. & Priest, E. R. 1997 Structure and collapse of three-dimensional magnetic neutral points. Geophys. Astrophys. Fluid Dyn. 84, 245–271.Google Scholar
Parnell, C. E., Smith, J. M., Neukirch, T. & Priest, E. R. 1996 The structure of three-dimensional magnetic neutral points. Phys. Plasmas 3, 759–770.CrossRefGoogle Scholar
Pontin, D. I. 2011 Three-dimensional magnetic reconnection regimes: a review. Adv. Space Res. 47, 1508–1522.CrossRefGoogle Scholar
Pontin, D. I. 2012 Theory of magnetic reconnection in solar and astrophysical plasmas. Phil. Trans. R. Soc. Lond. A 370, 3169–3192.Google Scholar
Pontin, D. I., Al-Hachami, A. K. & Galsgaard, K. 2011 Generalised models for torsional spine and fan magnetic reconnection. Astron. Astrophys. 533, A78.Google Scholar
Pontin, D. I. & Galsgaard, K. 2007 Current amplification and magnetic reconnection at a three-dimensional null point: physical characteristics. J. Geophys. Res. 112, 3103.Google Scholar
Pontin, D. I., Priest, E. R. & Galsgaard, K. 2013 On the nature of reconnection at a solar coronal null point above a separatrix dome. Astrophys. J. 774, 154.Google Scholar
Priest, E. R. & Pontin, D. I. 2009 Three-dimensional null point reconnection regimes. Phys. Plasmas 16 (12), 122101–122101.Google Scholar
Raouafi, N.-E., Georgoulis, M. K., Rust, D. M. & Bernasconi, P. N. 2010 Micro-sigmoids as progenitors of coronal jets: is eruptive activity self-similarly multi-scaled? Astrophys. J. 718, 981–987.Google Scholar
Schmidt, J. B., Sands, B., Scofield, J., Gord, J. R. & Roy, S. 2017 Comparison of femtosecond- and nanosecond-two-photon-absorption laser-induced fluorescence (TALIF) of atomic oxygen in atmospheric-pressure plasmas. Plasma Sources Sci. Technol. 26 (5), 055004.CrossRefGoogle Scholar
Stenzel, R. L., Urrutia, J. M., Griskey, M. C. & Strohmaier, K. D. 2001 3D EMHD reconnection in a laboratory plasma. Earth, Planets, and Space 53, 553–560.Google Scholar
Stenzel, R. L., Urrutia, J. M., Griskey, M. C. & Strohmaier, K. D. 2002 A new laboratory experiment on magnetic reconnection. Phys. Plasmas 9, 1925–1930.Google Scholar
Tang, V., Adams, M. L. & Rusnak, B. 2010 Dense plasma focus Z-pinches for high-gradient particle acceleration. IEEE Trans. Plasma Sci. 38, 719–727.Google Scholar
Thakur, S. C., Adriany, K., Gosselin, J. J., McKee, J., Scime, E. E., Sears, S. H. & Tynan, G. R. 2016 Laser induced fluorescence measurements of axial velocity, velocity shear, and parallel ion temperature profiles during the route to plasma turbulence in a linear magnetized plasma device. Rev. Sci. Instrum. 87 (11), 11E513.Google Scholar
Thurgood, J. O., Pontin, D. I. & McLaughlin, J. A. 2017 Three-dimensional oscillatory magnetic reconnection. Astrophys. J. 844, 2.Google Scholar
Török, T., Aulanier, G., Schmieder, B., Reeves, K. K. & Golub, L. 2009 Fan-spine topology formation through two-step reconnection driven by twisted flux emergence. Astrophys. J. 704, 485–495.Google Scholar
Valdivia, J. A., Rogan, J., Munoz, V. & Toledo, B. 2006 Hysteresis provides self-organization in a plasma model. Space Sci. Rev. 122, 313–320.Google Scholar
Wendel, D. E. & Adrian, M. L. 2013 Current structure and nonideal behavior at magnetic null points in the turbulent magnetosheath. J. Geophys. Res. 118, 1571–1588.Google Scholar
Willenborg, D. L. & Hendricks, C. D.1976 Design and construction of a dense plasma focus device, part 1. Tech. Rep. Google Scholar
Wyper, P. F., Antiochos, S. K. & DeVore, C. R. 2017 A universal model for solar eruptions. Nature 544, 452–455.Google Scholar
Yamada, M., Ji, H., Hsu, S., Carter, T., Kulsrud, R., Bretz, N., Jobes, F., Ono, Y. & Perkins, F. 1997a Study of driven magnetic reconnection in a laboratory plasma. Phys. Plasmas 4, 1936–1944.Google Scholar
Yamada, M., Ji, H., Hsu, S., Carter, T., Kulsrud, R., Ono, Y. & Perkins, F. 1997b Identification of Y-shaped and O-shaped diffusion regions during magnetic reconnection in a laboratory plasma. Phys. Rev. Lett. 78, 3117–3120.Google Scholar
Yamada, M., Yoo, J., Jara-Almonte, J., Ji, H., Kulsrud, R. M. & Myers, C. E. 2014 Conversion of magnetic energy in the magnetic reconnection layer of a laboratory plasma. Nat. Commun. 5, 4774.Google Scholar
Ziemer, J. K. & Choueiri, E. Y. 2001 Scaling laws for electromagnetic pulsed plasma thrusters. Plasma Sources Sci. Technol. 10, 395–405.CrossRefGoogle Scholar