Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T04:57:57.007Z Has data issue: false hasContentIssue false
  • English
  • Français

Driving magnetic turbulence using flux ropes in a moderate guide field linear system

Published online by Cambridge University Press:  09 November 2017

Matthew I. Brookhart ,
Aaron Stemo ,
Roger Waleffe  and
Cary B. Forest Open the ORCID record for Cary B. Forest [Opens in a new window]
Matthew I. Brookhart*
Affiliation:
Physics Department, 1150 University Ave, University of Wisconsin-Madison, Madison, WI 53706, USA
Aaron Stemo
Affiliation:
Physics Department, 1150 University Ave, University of Wisconsin-Madison, Madison, WI 53706, USA Department of Astrophysical and Planetary Sciences, University of Colorado Boulder, 2000 Colorado Ave, Boulder, CO 80309, USA
Roger Waleffe
Affiliation:
Physics Department, 1150 University Ave, University of Wisconsin-Madison, Madison, WI 53706, USA
Cary B. Forest
Affiliation:
Physics Department, 1150 University Ave, University of Wisconsin-Madison, Madison, WI 53706, USA
*
†Email address for correspondence: matthewbrookhart@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a series of experiments on novel, line-tied plasma geometries as a study of the generation of chaos and turbulence in line-tied systems. Plasma production and the injection scale for magnetic energy is provided by spatially discrete plasma guns that inject both plasma and current. The guns represent a technique for controlling the injection scale of magnetic energy. A two-dimensional (2-D) array of magnetic probes provides spatially resolved time histories of the magnetic fluctuations at a single cross-section of the experimental cylinder, allowing simultaneous spatial measurements of chaotic and turbulent behaviour. The first experiment shows chaotic fluctuations and self-organization in a hollow-current line-tied screw pinch. These dynamics is modulated primarily by the applied magnetic field and weakly by the plasma current and safety factor. The second experiment analyses the interactions of multiple line-tied flux ropes. The flux ropes all exhibit chaotic behaviour, and under certain conditions develop an inverse cascade to larger scales and a turbulent inertial range with magnetic energy ($E$) related to perpendicular wave number (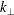$k_{\bot }$) as $E\propto k_{\bot }^{-2.5\pm 0.5}$.

Keywords

plasma dynamicsplasma instabilitiesplasma nonlinear phenomena
Type
Research Article
Information
Journal of Plasma Physics , Volume 83 , Issue 6 , December 2017 , 905830604
Copyright
© Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alexakis, A. 2011 Two-dimensional behavior of three-dimensional magnetohydrodynamic flow with a strong guiding field. Phys. Rev. E 84 (5), 1–9.Google Scholar
Bandt, C. & Pompe, B. 2002 Permutation entropy: a natural complexity measure for time series. Phys. Rev. Lett. 88 (17), 174102.Google Scholar
Bergerson, W. F., Forest, C. B., Fiksel, G., Hannum, D. A., Kendrick, R., Sarff, J. S. & Stambler, S. 2006 Onset and saturation of the kink instability in a current-carrying line-tied plasma. Phys. Rev. Lett. 96 (1), 015004.CrossRefGoogle Scholar
Brookhart, M. I., Stemo, A., Zuberbier, A., Zweibel, E. & Forest, C. B. 2015 Instability, turbulence, and 3D magnetic reconnection in a line-tied, zero net current screw pinch. Phys. Rev. Lett. 114 (14), 145001.Google Scholar
Cooper, C. M., Wallace, J., Brookhart, M., Clark, M., Collins, C., Ding, W. X., Flanagan, K., Khalzov, I., Li, Y., Milhone, J. et al. 2014 The Madison plasma dynamo experiment: a facility for studying laboratory plasma astrophysics. Phys. Plasmas 21, 013505.Google Scholar
Delzanno, G. L., Evstatiev, E. G. & Finn, J. M. 2007 The role of resistivity on line-tied kink modes in cylindrical geometry. Phys. Plasmas 14 (7), 070702.Google Scholar
Fiksel, G., Almagri, A. F., Craig, D., Iida, M., Prager, S. C. & Sarff, J. S. 1996 High current plasma electron emitter. Plasma Sources Sci. Technol. 5 (1), 78–83.Google Scholar
Forest, C. B., Flanagan, K., Brookhart, M., Clark, M., Cooper, C. M., Désangles, V., Egedal, J., Endrizzi, D., Khalzov, I. V., Li, H. et al. 2015 The wisconsin plasma astrophysics laboratory. J. Plasma Phys. 81 (5), 1–21.CrossRefGoogle Scholar
Furno, I., Intrator, T. P., Hemsing, E. W., Hsu, S. C., Abbate, S., Ricci, P. & Lapenta, G. 2005 Coalescence of two magnetic flux ropes via collisional magnetic reconnection. Phys. Plasmas 12 (5), 055702.Google Scholar
Furno, I., Intrator, T. P., Lapenta, G., Dorf, L., Abbate, S. & Ryutov, D. D. 2007 Effects of boundary conditions and flow on the kink instability in a cylindrical plasma column. Phys. Plasmas 14 (2), 022103.Google Scholar
Gekelman, W., Maggs, J. E. & Pfister, H. 1992 Experiments on the interaction of current channels in a laboratory plasma: relaxation to the force-free state. Plasma Sci. IEEE 20 (6), 614–621.Google Scholar
Gekelman, W., Van Compernolle, B., Dehaas, T. & Vincena, S. 2014 Chaos in magnetic flux ropes. Plasma Phys. Control. Fusion 56 (6), 064002.Google Scholar
Hood, A. W. 1993 The stability of line-tied coronal loops and an extended Suydam criterion. Adv. Space Res. 13 (9), 105–108.Google Scholar
Hsu, S. C. & Bellan, P. M. 2003 Experimental identification of the kink instability as a poloidal flux amplification mechanism for coaxial gun spheromak formation. Phys. Rev. Lett. 90 (21), 1–4.Google Scholar
Intrator, T. P., Sun, X., Lapenta, G., Dorf, L. & Furno, I. 2009 Experimental onset threshold and magnetic pressure pile-up for 3D reconnection. Nat. Phys. 5 (7), 521–526.Google Scholar
Khalzov, I. V., Ebrahimi, F., Schnack, D. D. & Mirnov, V. V. 2012 Minimum energy states of the cylindrical plasma pinch in single-fluid and Hall magnetohydrodynamics. Phys. Plasmas 19 (1), 012111.CrossRefGoogle Scholar
Lawrence, E. & Gekelman, W. 2009 Identification of a quasiseparatrix layer in a reconnecting laboratory magnetoplasma. Phys. Rev. Lett. 103 (10), 2–5.Google Scholar
Maggs, J. E. & Morales, G. J. 2013 Permutation entropy analysis of temperature fluctuations from a basic electron heat transport experiment. Plasma Phys. Control. Fusion 55 (8), 085015.CrossRefGoogle Scholar
Paz-Soldan, C., Bergerson, W. F., Brookhart, M. I., Hannum, D. A., Kendrick, R., Fiksel, G. & Forest, C. B. 2010 The rotating wall machine: a device to study ideal and resistive magnetohydrodynamic stability under variable boundary conditions. Rev. Sci. Instrum. 81 (12), 123503.Google Scholar
Paz-Soldan, C., Brookhart, M. I., Clinch, A. J., Hannum, D. A. & Forest, C. B. 2011 Two-dimensional axisymmetric and three-dimensional helical equilibrium in the line-tied screw pinch. Phys. Plasmas 18 (5), 052114.Google Scholar
Rappazzo, A. F., Velli, M. & Einaudi, G. 2013 Field lines twisting in a noisy corona: implications for energy storage and release, and initiation of solar eruptions. Astrophys. J. 771 (2), 76.Google Scholar
Rosso, O., Larrondo, H., Martin, M., Plastino, a. & Fuentes, M. 2007 Distinguishing noise from chaos. Phys. Rev. Lett. 99 (15), 154102.Google Scholar
Russell, C. T., Priest, E. R. & Lee, L. C.(Eds) 1990 Physics of Magnetic Flux Ropes. American Geophysical Union.Google Scholar
Ryutov, D. D., Furno, I., Intrator, T. P., Abbate, S. & Madziwa-Nussinov, T. 2006 Phenomenological theory of the kink instability in a slender plasma column. Phys. Plasmas 13 (3), 032105.Google Scholar
Sun, X., Intrator, T. P., Dorf, L., Sears, J., Furno, I. & Lapenta, G. 2010 Flux rope dynamics: Experimental study of bouncing and merging. Phys. Rev. Lett. 105 (25), 3–6.Google Scholar
Suydam, B. R. 1958 Stability of a linear pinch. J. Nuclear Energy 7 (3–4), 275–276.Google Scholar
Taylor, J. B. 1986 Relaxation and magnetic reconnection in plasmas. Rev. Mod. Phys. 58 (3), 741–763.Google Scholar
Van Compernolle, B. & Gekelman, W. 2012 Morphology and dynamics of three interacting kink-unstable flux ropes in a laboratory magnetoplasma. Phys. Plasmas 19 (10), 102102.Google Scholar
Supplementary material: File

Brookhart et al supplementary material 1

Brookhart et al supplementary material

Download Brookhart et al supplementary material 1(File)
File 17.4 MB