Hostname: page-component-6bf8c574d5-b4m5d Total loading time: 0 Render date: 2025-03-01T02:40:14.074Z Has data issue: false hasContentIssue false
  • English
  • Français

Inverse cascade and magnetic vortices in kinetic Alfvén-wave turbulence

Part of: Focus on Plasma Astrophysics

Published online by Cambridge University Press:  08 March 2021

G. Miloshevich Open the ORCID record for G. Miloshevich [Opens in a new window] ,
D. Laveder ,
T. Passot  and
P. L. Sulem Open the ORCID record for P. L. Sulem [Opens in a new window]
G. Miloshevich*
Affiliation:
Université Côte d'Azur, Observatoire de la Côte d'Azur, CNRS, Laboratoire J. L. Lagrange, Boulevard de l'Observatoire, CS 34229, 06304Nice CEDEX 4, France Ècole Normale Supérieure de Lyon Laboratoire de Physique, 46, allée d'Italie, F-69364, Lyon cedex 07, France
D. Laveder
Affiliation:
Université Côte d'Azur, Observatoire de la Côte d'Azur, CNRS, Laboratoire J. L. Lagrange, Boulevard de l'Observatoire, CS 34229, 06304Nice CEDEX 4, France
T. Passot
Affiliation:
Université Côte d'Azur, Observatoire de la Côte d'Azur, CNRS, Laboratoire J. L. Lagrange, Boulevard de l'Observatoire, CS 34229, 06304Nice CEDEX 4, France
P. L. Sulem
Affiliation:
Université Côte d'Azur, Observatoire de la Côte d'Azur, CNRS, Laboratoire J. L. Lagrange, Boulevard de l'Observatoire, CS 34229, 06304Nice CEDEX 4, France
*
†Email address for correspondence: george.miloshevich@ens-lyon.fr
Get access Rights & Permissions [Opens in a new window]

Abstract

A Hamiltonian two-field gyrofluid model for kinetic Alfvén waves (KAWs) in a magnetized electron–proton plasma, retaining ion finite-Larmor-radius corrections and parallel magnetic field fluctuations, is used to study the inverse cascades that develop when turbulence is randomly driven at sub-ion scales. In the directions perpendicular to the ambient field, the dynamics of the cascade turns out to be non-local and the ratio 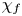$\chi _f$ of the wave period to the characteristic nonlinear time at the driving scale affects some of its properties. For example, at small values of $\chi _f$, parametric decay instability of the modes driven by the forcing can develop, enhancing for a while inverse transfers. The balanced state, obtained at early time when the two counter-propagating waves are equally driven, also becomes unstable at small 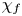$\chi _f$, leading to an inverse cascade. For $\beta _e$ smaller than a few units, the cascade slows down when reaching the low-dispersion spectral range. For higher 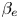$\beta _e$, the ratio of the KAW to the Alfvén frequencies displays a local minimum. At the corresponding transverse wavenumber, a condensate is formed, and the cascade towards larger scales is then inhibited. Depending on the parameters, a parallel inverse cascade can develop, enhancing the elongation of the ion-scale magnetic vortices that generically form.

Keywords

astrophysical plasmasplasma wavesplasma simulation
Type
Research Article
Information
Journal of Plasma Physics , Volume 87 , Issue 2 , April 2021 , 905870201
Check for updates
Copyright
Copyright © The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abdelhamid, H. M., Lingam, M. & Mahajan, S. M. 2016 Extended MHD turbulence and its applications to the solar wind. Astrophys. J. 829, 87.CrossRefGoogle Scholar
Alexakis, A. & Biferale, L. 2018 Cascades and transitions in turbulent flows. Phys. Rep. 767–769, 1–101.CrossRefGoogle Scholar
Alexakis, A., Bigot, B., Politano, H. & Galtier, S. 2007 Anisotropic fluxes and nonlocal interactions in magnetohydrodynamic turbulence. Phys. Rev. E 76, 056313.CrossRefGoogle ScholarPubMed
Alexakis, A., Mininni, P. D. & Pouquet, A. 2006 On the inverse cascade of magnetic helicity. Astrophys. J 640, 335–343.CrossRefGoogle Scholar
Alexandrova, O. 2008 Solar wind vs magnetosheath turbulence and Alfvén vortices. Nonlinear Proc. Geophys. 15 (1), 95–108.CrossRefGoogle Scholar
Alexandrova, O., Saur, J., Lacombe, C., Mangeney, A., Mitchell, J., Schwartz, S. J. & Robert, P. 2009 Universality of solar-wind turbulent spectrum from MHD to electron scales. Phys. Rev. Lett. 103 (16), 165003.CrossRefGoogle ScholarPubMed
Balsara, D. & Pouquet, A. 1999 The formation of large-scale structures in supersonic magnetohydrodynamic flows. Phys. Plasmas 6 (1), 89–99.CrossRefGoogle Scholar
Belcher, J. W. & Davis, L. Jr. 1971 Large-amplitude Alfvén waves in the interplanetary medium, 2. J. Geophys. Res. 76, 3534.CrossRefGoogle Scholar
Benavides, S. J. & Alexakis, A. 2017 Critical transitions in thin layer turbulence. J. Fluid Mech. 822, 364–385.CrossRefGoogle Scholar
Biskamp, D., Schwarz, E., Zeiler, A., Celani, A. & Drake, J. F. 1999 Electron magnetohydrodynamic turbulence. Phys. Plasmas 6, 751–758.CrossRefGoogle Scholar
Boldyrev, S., Horaites, K., Xia, Q. & Perez, J. C. 2013 Toward a theory of astrophysical plasma turbulence at subproton scales. Astrophys. J. 777, 41.CrossRefGoogle Scholar
Brandenburg, A. 2001 The inverse cascade and nonlinear alpha-effect in simulations of isotropic helical hydromagnetic turbulence. Astrophys. J. 550 (2), 824–840.CrossRefGoogle Scholar
Brandenburg, A. & Matthaeus, W. H. 2004 Magnetic helicity evolution in a periodic domain with imposed field. Phys. Rev. E 69, 056407.CrossRefGoogle Scholar
Bruno, R., Telloni, D., DeIure, D. & Pietropaolo, E. 2017 Solar wind magnetic field background spectrum from fluid to kinetic scales. Mon. Not. R. Astron. Soc. 472, 1052–1059.CrossRefGoogle Scholar
Bruno, R., Trenchi, L. & Telloni, D. 2014 Spectral slope variation at proton scales from fast to slow solar wind. Astrophys. J. Lett. 793 (1), L15.CrossRefGoogle Scholar
Cerri, S. S. & Califano, F. 2017 Reconnection and small-scale fields in 2D-3V hybrid-kinetic driven turbulence simulations. New J. Phys. 19, 025007.CrossRefGoogle Scholar
Chen, C. H. K., Bale, S. D., Bonnell, J. W., Borovikov, D., Bowen, T. A., Burgess, D., Case, A. W., Chandran, B. D. G., de Wit, T. D., Goetz, K., et al. 2020 The evolution and role of solar wind turbulence in the inner heliosphere. Astrophys. J. Suppl. 246 (2), 53.CrossRefGoogle Scholar
Chen, C. H. K. & Boldyrev, S. 2017 Nature of kinetic scale turbulence in the Earth's magnetosheath. Astrophys. J. 842, 122.CrossRefGoogle Scholar
D'Amicis, R., Matteini, L. & Bruno, R. 2019 On the slow solar wind with high Alfvénicity: from composition and microphysics to spectral properties. Mon. Not. R. Astron. Soc. 483, 4665–4677.Google Scholar
Del Zanna, L., Velli, M. & Londrillo, P. 2001 Parametric decay of circularly polarized Alfvén waves: multidimensional simulations in periodic and open domains. Astron. Astrophys. 367, 705–718.CrossRefGoogle Scholar
Fjørtoft, R. 1953 On changes in the spectral distribution of kinetic energy for two-dimensional nondivergent flow. Tellus 5, 225.CrossRefGoogle Scholar
Franci, L., Cerri, S. S., Califano, F., Landi, S., Papini, E., Verdini, A., Matteini, L., Jenko, F. & Hellinger, P. 2017 Magnetic reconnection as a driver for a sub-ion-scale cascade in plasma turbulence. Astrophys. J. Lett. 850, L16.CrossRefGoogle Scholar
Frisch, U., Pouquet, A., Léorat, J. & Mazure, A. 1975 Possiblility of an inverse cascade of magnetic helicity in magnetohydrodynamic turbulence. J. Fluid Mech. 68, 769–778.CrossRefGoogle Scholar
Fu, X., Li, H., Guo, F., Li, X. & Roytershteyn, V. 2018 Parametric decay instability and dissipation of low-frequency Alfvén waves in low-beta turbulent plasmas. Astrophys. J. 855 (2), 139.CrossRefGoogle Scholar
Galtier, S. & Meyrand, R. 2015 Entanglement of helicity and energy in kinetic Alfvén wave/whistler turbulence. J. Plasma Phys. 81, 325810106.CrossRefGoogle Scholar
He, J., Duan, D., Zhu, X., Yan, L. & Wang, L. 2019 Observational evidences of wave excitation and inverse cascade in a distant earth foreshock region. Sci. China 62, 619–630.CrossRefGoogle Scholar
Howes, G. G., Cowley, S. C., Dorland, W., Hammett, G. W., Quataert, E. & Schekochihin, A. A. 2006 Astrophysical gyrokinetics: basic equations and linear theory. Astrophys. J. 651 (1), 590–614.CrossRefGoogle Scholar
Jovanović, D., Alexandrova, O., Maksimović, M. & Belić, M. 2020 Fluid theory of coherent magnetic vortices in high-${{\beta }}$ space plasmas. Astrophys. J. 896 (1), 8.CrossRefGoogle Scholar
Kim, H. & Cho, J. 2015 Inverse cascade in imbalanced electron magnetohydrodynamic turbulence. Astrophys. J. 801, 75.CrossRefGoogle Scholar
Linkmann, M. & Dallas, V. 2016 Large-scale dynamics of magnetic helicity. Phys. Rev. E 94, 053209.CrossRefGoogle ScholarPubMed
Linkmann, M. & Dallas, V. 2017 Triad interactions and the bidirectional turbulent cascade of magnetic helicity. Phys. Rev. Fluids 2, 054605.CrossRefGoogle Scholar
Lucek, E. A. & Balogh, A. 1998 The identification and characterization of Alfvénic fluctuations in Ulysses data at midlatitudes. Astrophys. J. 507, 984–990.CrossRefGoogle Scholar
Müller, W.-C., Malapaka, S. K. & Busse, A. 2012 Inverse cascade of magnetic helicity in magnetohydrodynamic turbulence. Phys. Rev. E 85, 015302(R).CrossRefGoogle ScholarPubMed
Marino, R., Mininni, P. D., Rosenberg, D. & Pouquet, A. 2013 Inverse cascades in rotating stratified turbulence: fast growth of large scales. Europhys. Lett. 102 (4), 44006.CrossRefGoogle Scholar
Maron, J. & Goldreich, P. 2001 Simulations of incompressible magnetohydrodynamic turbulence. Astrophys. J. 554 (2), 1175–1196.CrossRefGoogle Scholar
Marsch, E. & Tu, C.-Y. 1990 On the radial evolution of MHD turbulence in the inner heliosphere. J. Geophys. Res. 95 (A6), 8211–8229.CrossRefGoogle Scholar
Matthaeus, W. H. & Goldstein, M. L. 1982 Measurement of the rugged invariants of magnetohydrodynamic turbulence in the solar wind. J. Geophys. Res. 87, 6011–6028.CrossRefGoogle Scholar
Meneguzzi, M., Frisch, U. & Pouquet, A. 1981 Helical and non helical tubulent dynamo. Phys. Rev. Lett. 47, 1660–1664.CrossRefGoogle Scholar
Miloshevich, G., Lingam, M. & Morrison, P. J. 2017 On the structure and statistical theory of turbulence of extended magnetohydrodynamics. New J. Phys. 19, 015007.CrossRefGoogle Scholar
Miloshevich, G., Passot, T. & Sulem, P. L. 2019 Modeling imbalanced collisionless Alfvén wave turbulence with nonlinear diffusion equations. Astrophys. J. 888, L7.CrossRefGoogle Scholar
Mininni, P. D., Alexakis, A. & Pouquet, A. 2007 Energy transfer in hall-MHD turbulence: cascades, backscatter, and dynamo action. J. Plasma Phys. 73 (3), 377–401.CrossRefGoogle Scholar
Nazarenko, S. 2011 Wave turbulence. Lectures Notes in Physics, vol. 825. Springer.CrossRefGoogle Scholar
Passot, T. & Sulem, P. L. 2019 Imbalanced kinetic Alfvén wave turbulence: from weak turbulence theory to nonlinear diffusion models for the strong regime. J. Plasma Phys. 85, 905850301.CrossRefGoogle Scholar
Passot, T., Sulem, P. L. & Tassi, E. 2018 Gyrofluid modeling and phenomenology of low-$\beta _e$ Alfvén wave turbulence. Phys. Plasmas 25, 042107.CrossRefGoogle Scholar
Perrone, D., Alexandrova, O., Roberts, O. W., Lion, S., Lacombe, C., Walsh, A., Maksimovic, M. & Zouganelis, I. 2017 Coherent structures at ion scales in fast solar wind: cluster observations. Astrophys. J. 849 (1), 49.CrossRefGoogle Scholar
Petviashvili, V. & Pokhotelov, O. 1992 Solitary Waves in Plasmas and in the Atmosphere. Gordon and Breach.Google Scholar
Podesta, J. J. 2013 Evidence of kinetic Alfvén waves in the solar wind at 1 AU. Solar Phys. 286, 529–548.CrossRefGoogle Scholar
Pouquet, A., Frisch, U. & Leorat, J. 1976 Strong MHD helical turbulence and the nonlinear dynamo effect. J. Fluid Mech. 77, 321–354.CrossRefGoogle Scholar
Pouquet, A., Rosenberg, D., Stawarz, J. E. & Marino, R. 2019 Helicity dynamics, inverse, and bidirectional cascades in fluid and magnetohydrodynamic turbulence: a brief review. Earth Space Sci. 6, 351–369.CrossRefGoogle Scholar
Pouquet, A., Stawarz, J. E. & Rosenberg, D. 2020 Coupling large eddies and waves in turbulence: case study of magnetic helicity at the ion inertial scale. Atmosphere 11 (2), 203.CrossRefGoogle Scholar
Réville, V., Velli, M., Panasenco, O., Tenerani, A., Shi, C., Badman, S. T., Bale, S. D., Kasper, J. C., Stevens, M. L., Korreck, K. E., et al. 2020 The role of Alfvén wave dynamics on the large-scale properties of the solar wind: comparing an MHD simulation with parker solar probe E1 data. Astrophys. J. Suppl. 246 (2), 24.CrossRefGoogle Scholar
Roberts, D. A., Goldstein, M. L., Klein, L. W. & Matthaeus, W. H. 1987 Origin and evolution of fluctuations in the solar wind: Helios observations and Helios–Voyager comparisons. J. Geophys. Res. 92 (A11), 12023–12035.CrossRefGoogle Scholar
Sahraoui, F., Goldstein, M. L., Belmont, G., Canu, P. & Rezeau, L. 2010 Three dimensional anisotropic $k$ spectra of turbulence at subproton scales in the solar wind. Phys. Rev. Lett. 105, 131101.CrossRefGoogle ScholarPubMed
Salem, C. S., Howes, G. G., Sundkvist, D., Bale, S. D., Chaston, C. C., Chen, C. H. K. & Mozer, F. S. 2012 Identification of kinetic Alfvén wave turbulence in the solar wind. Astrophys. J. Lett. 745, L9.CrossRefGoogle Scholar
Schekochihin, A. A., Cowley, S. C., Dorland, W., Hammett, G. W., Howes, G. G., Quataert, E. & Tatsuno, T. 2009 Astrophysical gyrokinetics: kinetic and fluid turbulent cascades in magnetized weakly collisional plasmas. Astrophys. J. Suppl. 182, 310–377.CrossRefGoogle Scholar
Seshasayanan, K., Benavides, S. J. & Alexakis, A. 2014 On the edge of an inverse cascade. Phys. Rev. E 90, 051003.CrossRefGoogle ScholarPubMed
Shi, M., Li, H., Xiao, C. & Wang, X. 2017 The parametric decay instability of Alfvén waves in turbulent plasmas and the applications in the solar wind. Astrophys. J. 842 (1), 63.CrossRefGoogle Scholar
Shoda, M. & Yokoyama, T. 2018 Anisotropic magnetohydrodynamic turbulence driven by parametric decay instability: the onset of phase mixing and Alfvén wave turbulence. Astrophys. J. 859 (2), L17.CrossRefGoogle Scholar
Slepyan, L. I. 2015 On the energy partition in oscillations and waves. Proc. R. Soc. Lond. A 471, 20140838.Google Scholar
Solano, E. R., Lomas, P. J., Alper, B., Xu, G. S., Andrew, Y., Arnoux, G., Boboc, A., Barrera, L., Belo, P., Beurskens, M. N. A., et al. 2010 Observation of confined current ribbon in jet plasmas. Phys. Rev. Lett. 104, 185003.CrossRefGoogle ScholarPubMed
Stribling, T., Matthaeus, W. H. & Ghosh, S. 1994 Nonlinear decay of magnetic helicity in magnetohydrodynamic turbulence with a mean magnetic field. J. Geophys. Res. 99, 2567–2576.CrossRefGoogle Scholar
Tassi, E., Passot, T. & Sulem, P. L. 2020 A Hamiltonian gyrofluid model based on a quasi-static closure. J. Plasma Phys. 86, 835860402.CrossRefGoogle Scholar
Tu, C. Y., March, E. & Rausenbauer, H. 1990 The dependence of MHD turbulence spectra on the inner solar wind stream structure near solar minimum. Geophys. Res. Lett. 17, 283–286.CrossRefGoogle Scholar
Tu, C.-Y., Marsch, E. & Thieme, K. M. 1989 Basic properties of solar wind MHD turbulence near 0.3 AU analyzed by means of elsasser variables. J. Geophys. Res. 94 (A9), 11739–11759.CrossRefGoogle Scholar
Viñas, A. F. & Goldstein, M. L. 1991 Parametric instabilities of circularly polarized large-amplitude dispersive Alfvén waves: excitation of obliquely-propagating daughter and side-band waves. J. Plasma Phys. 46, 129–152.CrossRefGoogle Scholar
Voitenko, Y. M. 1998 a Three-wave coupling and parametric decay of kinetic Alfvén waves. J. Plasma Phys. 60, 497–514.CrossRefGoogle Scholar
Voitenko, Y. M. 1998 b Three-wave coupling and weak turbulence of kinetic Alfvén waves. J. Plasma Phys. 60, 515–527.CrossRefGoogle Scholar
Wang, T., Alexandrova, O., Perrone, D., Dunlop, M., Dong, X., Bingham, R., Khotyaintsev, Y. V., Russell, C. T., Giles, B. L., Torbert, R. B., et al. 2019 Magnetospheric multiscale observation of kinetic signatures in the Alfvén vortex. Astrophys. J. Lett. 871 (2), L22.CrossRefGoogle Scholar
Wicks, R. T., Roberts, D. A., Mallet, A., Schekochihin, A. A., Horbury, T. S. & Chen, C. H. K. 2013 Correlations at large scales and the onset of turbulence in the fast solar wind. Astrophys. J. 778, 177.CrossRefGoogle Scholar
Zakharov, V. E., L'Vov, V. S. & Falkovich, G. 1992 Kolmogorov Spectra of Turbulence I: Wave Turbulence. Springer Series in Nonlinear Dynamics, Springer Verlag.CrossRefGoogle Scholar
Zhao, J. S., Lu, J. Y. & Wu, D. J. 2010 a Parametric instability of whistler waves in the electron magnetohydrodynamics. Astrophys. J. 714, 138–143.CrossRefGoogle Scholar
Zhao, J. S., Wu, D. J. & Lu, J. Y. 2010 b On nonlinear decay of kinetic Alfvén waves and application to some processes in space plasmas. J. Geophys. Res. 115, A12227.Google Scholar
Zhou, M., Loureiro, N. F. & Uzdensky, D. A. 2020 Multi-scale dynamics of magnetic flux tubes and inverse magnetic energy transfer. J. Plasma Phys. 86, 535860401.CrossRefGoogle Scholar
Zocco, A. & Schekochihin, A. A. 2011 Reduced fluid-kinetic equations for low-frequency dynamics, magnetic reconnection, and electron heating in low-beta plasmas. Phys. Plasmas 18, 102309.CrossRefGoogle Scholar