Article contents
COMPUTABLE POLISH GROUP ACTIONS
Published online by Cambridge University Press: 01 August 2018
Abstract
Using methods from computable analysis, we establish a new connection between two seemingly distant areas of logic: computable structure theory and invariant descriptive set theory. We extend several fundamental results of computable structure theory to the more general setting of topological group actions. As we will see, the usual action of 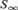 ${S_\infty }$ on the space of structures in a given language is effective in a certain algorithmic sense that we need, and
${S_\infty }$ on the space of structures in a given language is effective in a certain algorithmic sense that we need, and  ${S_\infty }$ itself carries a natural computability structure (to be defined). Among other results, we give a sufficient condition for an orbit under effective
${S_\infty }$ itself carries a natural computability structure (to be defined). Among other results, we give a sufficient condition for an orbit under effective 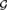 ${\cal G}$-action of a computable Polish
${\cal G}$-action of a computable Polish 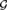 ${\cal G}$ to split into infinitely many disjoint effective orbits. Our results are not only more general than the respective results in computable structure theory, but they also tend to have proofs different from (and sometimes simpler than) the previously known proofs of the respective prototype results.
${\cal G}$ to split into infinitely many disjoint effective orbits. Our results are not only more general than the respective results in computable structure theory, but they also tend to have proofs different from (and sometimes simpler than) the previously known proofs of the respective prototype results.
Keywords
Information
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2018
References
REFERENCES
- 8
- Cited by

