Article contents
Extremely undecidable sentences
Published online by Cambridge University Press: 12 March 2014
Extract
Let ‘ϕ’, ‘χ’, and ‘ψ’ be variables ranging over functions from the sentence letters P0, P1, … Pn, … of (propositional) modal logic to sentences of P(eano) Arithmetic), and for each sentence A of modal logic, inductively define Aϕ by
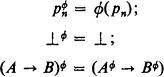
[and similarly for other nonmodal propositional connectives]; and

where Bew(x) is the standard provability predicate for PA and ⌈F⌉ is the PA numeral for the Gödel number of the formula F of PA. Then for any ϕ, (−□⊥)ϕ = −Bew(⌈⊥⌉), which is the consistency assertion for PA; a sentence S is undecidable in PA iff both 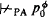 and
and 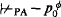 , where ϕ(p0) = S. If ψ(p0) is the undecidable sentence constructed by Gödel, then ⊬PA (−□⊥→ −□p0 & − □ − p0)ψ and ⊢PA(P0 ↔ −□⊥)ψ. However, if ψ(p0) is the undecidable sentence constructed by Rosser, then the situation is the other way around: ⊬PA(P0 ↔ −□⊥)ψ and ⊢PA (−□⊥→ −□−p0 & −□−p0)ψ. We call a sentence S of PA extremely undecidable if for all modal sentences A containing no sentence letter other than p0, if for some ψ, ⊬PAAψ, then ⊬PAAϕ, where ϕ(p0) = S. (So, roughly speaking, a sentence is extremely undecidable if it can be proved to have only those modal-logically characterizable properties that every sentence can be proved to have.) Thus extremely undecidable sentences are undecidable, but neither the Godel nor the Rosser sentence is extremely undecidable. It will follow at once from the main theorem of this paper that there are infinitely many inequivalent extremely undecidable sentences.
, where ϕ(p0) = S. If ψ(p0) is the undecidable sentence constructed by Gödel, then ⊬PA (−□⊥→ −□p0 & − □ − p0)ψ and ⊢PA(P0 ↔ −□⊥)ψ. However, if ψ(p0) is the undecidable sentence constructed by Rosser, then the situation is the other way around: ⊬PA(P0 ↔ −□⊥)ψ and ⊢PA (−□⊥→ −□−p0 & −□−p0)ψ. We call a sentence S of PA extremely undecidable if for all modal sentences A containing no sentence letter other than p0, if for some ψ, ⊬PAAψ, then ⊬PAAϕ, where ϕ(p0) = S. (So, roughly speaking, a sentence is extremely undecidable if it can be proved to have only those modal-logically characterizable properties that every sentence can be proved to have.) Thus extremely undecidable sentences are undecidable, but neither the Godel nor the Rosser sentence is extremely undecidable. It will follow at once from the main theorem of this paper that there are infinitely many inequivalent extremely undecidable sentences.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1982
References
REFERENCES
- 18
- Cited by

