Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mostowski, Marcin
and
Zdanowski, Konrad
2005.
New Computational Paradigms.
Vol. 3526,
Issue. ,
p.
358.
Mostowski, Marcin
and
Zdanowski, Konrad
2005.
Computer Science Logic.
Vol. 3634,
Issue. ,
p.
263.
Ferrarotti, Flavio A.
and
Torres, José M. Turull
2006.
Foundations of Information and Knowledge Systems.
Vol. 3861,
Issue. ,
p.
92.
Ferrarotti, Flavio Antonio
and
Turull Torres, José María
2007.
Arity and alternation: a proper hierarchy in higher order logics.
Annals of Mathematics and Artificial Intelligence,
Vol. 50,
Issue. 1-2,
p.
111.
Krynicki, Michał
Tomasik, Jerzy
and
Zdanowski, Konrad
2011.
Theories of initial segments of standard models of arithmetics and their complete extensions.
Theoretical Computer Science,
Vol. 412,
Issue. 31,
p.
3975.


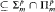 , then additionally
, then additionally 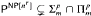 .
.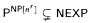 .
. arity hierarchy to be strict over finite models.
arity hierarchy to be strict over finite models.