Hostname: page-component-745bb68f8f-mzp66 Total loading time: 0 Render date: 2025-02-06T06:39:28.606Z Has data issue: false hasContentIssue false
  • English
  • Français

MONADIC INTUITIONISTIC AND MODAL LOGICS ADMITTING PROVABILITY INTERPRETATIONS

Part of: General logic Proof theory and constructive mathematics

Published online by Cambridge University Press:  02 December 2021

GURAM BEZHANISHVILI ,
KRISTINA BRANTLEY  and
JULIA ILIN
GURAM BEZHANISHVILI
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES NEW MEXICO STATE UNIVERSITY LAS CRUCES, NM 88003, USA E-mail: guram@nmsu.edu
KRISTINA BRANTLEY*
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES NEW MEXICO STATE UNIVERSITY LAS CRUCES, NM 88003, USA E-mail: guram@nmsu.edu
JULIA ILIN
Affiliation:
INSTITUTE OF LOGIC, LANGUAGE AND COMPUTATION UNIVERSITY OF AMSTERDAM P.O.BOX 94242, 1090 GE AMSTERDAM, THE NETHERLANDS
*
E-mail: kleifest@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

The Gödel translation provides an embedding of the intuitionistic logic 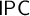$\mathsf {IPC}$ into the modal logic $\mathsf {Grz}$ , which then embeds into the modal logic 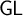$\mathsf {GL}$ via the splitting translation. Combined with Solovay’s theorem that $\mathsf {GL}$ is the modal logic of the provability predicate of Peano Arithmetic 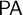$\mathsf {PA}$ , both $\mathsf {IPC}$ and $\mathsf {Grz}$ admit provability interpretations. When attempting to ‘lift’ these results to the monadic extensions $\mathsf {MIPC}$ 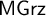, $\mathsf {MGrz}$ , and 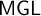$\mathsf {MGL}$ of these logics, the same techniques no longer work. Following a conjecture made by Esakia, we add an appropriate version of Casari’s formula to these monadic extensions (denoted by a ‘+’), obtaining that the Gödel translation embeds $\mathsf {M^{+}IPC}$ into 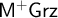$\mathsf {M^{+}Grz}$ and the splitting translation embeds $\mathsf {M^{+}Grz}$ into 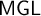$\mathsf {MGL}$ . As proven by Japaridze, Solovay’s result extends to the monadic system $\mathsf {MGL}$ , which leads us to a provability interpretation of both $\mathsf {M^{+}IPC}$ and 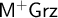$\mathsf {M^{+}Grz}$ .

Keywords

Intuitionistic logicmodal logicGödel translationprovability interpretationpredicate logicsmonadic logicsfinite model property

MSC classification

Primary: 03B45: Modal logic (including the logic of norms)
Secondary: 03F45: Provability logics and related algebras (e.g., diagonalizable algebras) 03B55: Intermediate logics
Type
Article
Information
The Journal of Symbolic Logic , Volume 88 , Issue 1 , March 2023 , pp. 427 - 467
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abashidze, M. A., Some properties of Magari algebras , Studies in Logic and Semantics, Metsniereba, Tbilisi, 1981, pp. 111–127 (Russian).Google Scholar
Artemov, S. N. and Beklemishev, L. D., Provability logic , Handbook of Philosophical Logic, vol. 13, second ed. (D. Gabbay and F. Guenthner, editors), Springer, Dordrecht, 2004, pp. 189–360.Google Scholar
Bezhanishvili, G., Varieties of monadic Heyting algebras. I . Studia Logica, vol. 61 (1998), no. 3, pp. 367–402.CrossRefGoogle Scholar
Bezhanishvili, G., Varieties of monadic Heyting algebras. II. Duality theory , Studia Logica, vol. 62 (1999), no. 1, pp. 21–48.CrossRefGoogle Scholar
Bezhanishvili, G., Varieties of monadic Heyting algebras. III . Studia Logica, vol. 64 (2000), no. 2, pp. 215–256.CrossRefGoogle Scholar
Bezhanishvili, G. and Carai, L., Temporal interpretation of monadic intuitionistic quantifiers . Review of Symbolic Logic, 2021, doi:10.1017/S1755020321000496.Google Scholar
Boolos, G., On systems of modal logic with provability interpretations . Theoria, vol. 46 (1980), no. 1, pp. 7–18.CrossRefGoogle Scholar
Boolos, G., The Logic of Provability, Cambridge University Press, Cambridge, 1993.Google Scholar
Brantley, K., Monadic Intuitionistic and Modal Logics Admitting Provability Interpretations, ProQuest LLC, Ann Arbor, 2019, Ph.D. thesis, New Mexico State University.Google Scholar
Bull, R. A., A modal extension of intuitionist logic . Notre Dame Journal of Formal Logic, vol. 6 (1965), pp. 142–146.Google Scholar
Bull, R. A., $\textrm{MIPC}$ as the formalisation of an intuitionist concept of modality , this Journal, vol. 31 (1966), pp. 609–616.Google Scholar
Chagrov, A. and Zakharyaschev, M., Modal Logic, Oxford Logic Guides, vol. 35, The Clarendon Press; Oxford University Press; Oxford Science Publications, New York, 1997.Google Scholar
Esakia, L. L., Topological Kripke models . Soviet Mathematics Doklady, vol. 15 (1974), pp. 147–151.Google Scholar
Esakia, L. L., On modal companions of superintuitionistic logics, VII Soviet Symposium on Logic , 1976, (Russian).Google Scholar
Esakia, L. L., On the variety of Grzegorczyk algebras , Studies in Nonclassical Logics and Set Theory , Nauka, Moscow, 1979, pp. 257–287 (Russian).Google Scholar
Esakia, L. L., Heyting Algebras I: Duality Theory, Metsniereba, Tbilisi, 1985 (Russian).Google Scholar
Esakia, L. L., Provability logic with quantifier modalities , Intensional Logics and the Logical Structure of Theories (Telavi, 1985), Metsniereba, Tbilisi, 1988, pp. 4–9 (Russian).Google Scholar
Esakia, L. L., Quantification in intuitionistic logic with provability smack . Bulletin of the Section of Logic, vol. 27 (1998), pp. 26–28.Google Scholar
Esakia, L. L., Intuitionistic logic and modality via topology . Annals of Pure and Applied Logic, vol. 127, 2004, pp. 155–170.CrossRefGoogle Scholar
Esakia, L. L., Heyting Algebras: Duality Theory (G. Bezhanishvili and W.H. Holliday, editors), Trends in Logic—Studia Logica Library, vol. 50, Springer, Cham, 2019, Translated from the 1985 Russian edition by A. Evseev.CrossRefGoogle Scholar
Fine, K., Logics containing $K4$ . I , this Journal, vol. 39 (1974), pp. 31–42.Google Scholar
Fischer-Servi, G., On modal logic with an intuitionistic base . Studia Logica, vol. 36 (1977), no. 3, pp. 141–149.CrossRefGoogle Scholar
Fischer-Servi, G., The finite model property for $\textsf{\textit{MIPQ}}$ and some consequences . Notre Dame Journal of Formal Logic, vol. 19 (1978), no. 4, pp. 687–692.CrossRefGoogle Scholar
Fischer-Servi, G., Semantics for a class of intuitionistic modal calculi . Bulletin of the Section of Logic, vol. 7 (1978), no. 1, pp. 26–30.Google Scholar
Gabbay, D. M., Kurucz, A., Wolter, F., and Zakharyaschev, M., Many-Dimensional Modal Logics: Theory and Applications , Studies in Logic and the Foundations of Mathematics, vol. 148, North-Holland Publishing Co., Amsterdam, 2003.Google Scholar
Gabbay, D. M., Shehtman, V. B., and Skvortsov, D. P., Quantification in Nonclassical Logic, vol. 1 , Studies in Logic and the Foundations of Mathematics, vol. 153, Elsevier B. V., Amsterdam, 2009.Google Scholar
Gödel, K., Eine Interpretation des intuitionistischen Aussagenkalküls . Ergebnisse eines mathematischen Kolloquiums , vol. 4 (1933), pp. 39–40, English translation in: Feferman, S. et al., editors, Kurt Gödel Collected Works, Vol. 1, pages 301–303. Oxford University Press, Oxford, Clarendon Press, New York, 1986.Google Scholar
Goldblatt, R., Arithmetical necessity, provability and intuitionistic logic . Theoria, vol. 44 (1978), no. 1, pp. 38–46.Google Scholar
Grefe, C., Fischer Servi’s intuitionistic modal logic has the finite model property , Advances in Modal Logic, vol. 1 (Berlin, 1996), CSLI Lecture Notes, vol. 87, CSLI Publications, Stanford, 1998, pp. 85–98.Google Scholar
Japaridze, G. K., Arithmetical completeness of provability logic with quantifier modalities . Soobshcheniya Akademii Nauk Gruzinskoĭ SSR , vol. 132 (1988), no. 2, pp. 265–268 (Russian).Google Scholar
Japaridze, G. K., Decidable and enumerable predicate logics of provability . Studia Logica, vol. 49 (1990), no. 1, pp. 7–21.CrossRefGoogle Scholar
Kuznetsov, A. V. and Muravitsky, A. Yu., Provability as modality , Current Problems of Logic and Methodology of Science, Naukova Dumka, Kiev, (1980), pp. 193–230 (Russian).Google Scholar
McKinsey, J. C. C. and Tarski, A., Some theorems about the sentential calculi of Lewis and Heyting , this Journal, vol. 13 (1948), pp. 1–15.Google Scholar
Montagna, F., The predicate modal logic of provability . Notre Dame Journal of Formal Logic, vol. 25 (1984), no. 2, pp. 179–189.Google Scholar
Monteiro, A. and Varsavsky, O., Algebras de Heyting monàdicas, Actas de las X jornadas , Unión Matemática Argentina, Instituto de Matemáticas, Universidad Nacional del Sur, Bahiá Blanca, 1957, pp. 52–62.Google Scholar
Ono, H., On some intuitionistic modal logics . Kyoto University, vol. 13 (1977/78), no. 3, pp. 687–722.CrossRefGoogle Scholar
Ono, H. and Suzuki, N.Y., Relations between intuitionistic modal logics and intermediate predicate logics . Reports on Mathematical Logic, vol. 22 (1988), pp. 65–87.Google Scholar
Prior, A. N., Time and Modality, Clarendon Press, Oxford, 1957.Google Scholar
Rasiowa, H. and Sikorski, R., The Mathematics of Metamathematics, Monografie Matematyczne, vol. 41, Państwowe Wydawnictwo Naukowe, Warsaw, 1963.Google Scholar
Solovay, R. M., Provability interpretations of modal logic . Israel Journal of Mathematics , vol. 25 (1976), nos. 3–4, pp. 287–304.CrossRefGoogle Scholar