Article contents
Provably total functions of intuitionistic bounded arithmetic
Published online by Cambridge University Press: 12 March 2014
Extract
This note deals with a proof-theoretic characterisation of certain complexity classes of functions in fragments of intuitionistic bounded arithmetic. In this Introduction we survey the background and state our main result.
We follow Buss [B1] and consider a language for arithmetic whose nonlogical symbols are 0, S (the successor operation Sx = x + 1), +, ·, ∣ ∣ (∣x∣ being the number of digits in the binary notation for x), 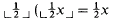 rounded down to the nearest integer), # (x#y = 2∣x∣∣y∣) and ≤. We define 1 = S0, 2 = S1, s0x = 2x and s1x = 2x + 1. In Buss's approach the functions s0 and s1 play a special role. Notice that six is the number obtained from x by suffixing the digit i to its binary representation, and thus the natural numbers are generated from 0 by repeated applications of the operations s0 and s1. This means that they satisfy the induction scheme
rounded down to the nearest integer), # (x#y = 2∣x∣∣y∣) and ≤. We define 1 = S0, 2 = S1, s0x = 2x and s1x = 2x + 1. In Buss's approach the functions s0 and s1 play a special role. Notice that six is the number obtained from x by suffixing the digit i to its binary representation, and thus the natural numbers are generated from 0 by repeated applications of the operations s0 and s1. This means that they satisfy the induction scheme
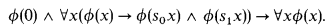
Using the fact that  is x with its last binary digit deleted, this can be stated more compactly in the following form, called by Buss the polynomial induction or PIND schema:
is x with its last binary digit deleted, this can be stated more compactly in the following form, called by Buss the polynomial induction or PIND schema:
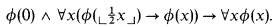
Buss defined a theory S2 consisting of a finite set BASIC of open axioms and the PIND-schema restricted to bounded formulas ϕ. The topic of bounded arithmetic is concerned with S2 and its fragments.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1992
References
REFERENCES
- 12
- Cited by

